如果你也在 怎样代写半导体物理Semiconductor Physics这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。半导体物理Semiconductor Physics它们具有适度的导电性。这类材料的例子有锗、硅、碳等。由于这些材料的导电性介于良好导体和绝缘体之间,这些材料被称为半导体。
半导体物理Semiconductor Physics半导体材料的电导率值介于导体(如金属铜)和绝缘体(如玻璃)之间。它的电阻率随着温度的升高而下降;而金属的表现则相反。它的导电性能可以通过在晶体结构中引入杂质(”掺杂”)的方式进行有用的改变。当同一晶体中存在两个不同的掺杂区域时,就会产生一个半导体结。电荷载体(包括电子、离子和电子空穴)在这些结上的行为是二极管、晶体管和大多数现代电子产品的基础。半导体的一些例子是硅、锗、砷化镓和周期表上所谓 “金属阶梯 “附近的元素。继硅之后,砷化镓是第二种最常见的半导体,用于激光二极管、太阳能电池、微波频率集成电路和其他。硅是制造大多数电子电路的一个关键元素。
my-assignmentexpert™半导体物理Semiconductor Physics作业代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。my-assignmentexpert™, 最高质量的半导体物理Semiconductor Physics作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于统计Statistics作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此半导体物理Semiconductor Physics作业代写的价格不固定。通常在经济学专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
my-assignmentexpert™ 为您的留学生涯保驾护航 在物理Physics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的物理Physics代写服务。我们的专家在半导体物理Semiconductor Physics代写方面经验极为丰富,各种半导体物理Semiconductor Physics相关的作业也就用不着 说。
我们提供的半导体物理Semiconductor Physics及其相关学科的代写,服务范围广, 其中包括但不限于:

物理代写|半导体物理代写Semiconductor Physics代考|Equilibrium
We first consider a single-component system with an equilibrium of two phases like solid silicon in equilibrium with its own melt. The solid surface represents a boundary between the two phases, and the amount of substance leaving one phase
equals the amount of substance entering the other phase, i.e., $-d n_{\mathrm{Si}, \text { liquid }}=+d n_{\mathrm{Si}, \text { solid }}$. In equilibrium, $d n_{\mathrm{Si}, \text { liquid }}=d n_{\mathrm{Si} \text {,solid }}=0$. The deviation from equilibrium required to drive particles across the phase boundary toward the solid phase is obtained from the chemical potential $\mu$. For the considered single-component system, $\mu(T, P)=G(T, P) / n$. The state function Gibbs energy $G(T, P)$ of the system is related to the internal energy $U$ $(S, V)$ by $^{4} G(T, P)=U+P V-T S$, and $n$ is the amount of substance (usually expressed in units of mole). For two coexisting phases, $\mu$ is composed of the respective two parts, i.e., $\mu=\mu_{\mathrm{Si}, \text { liquid }}+\mu_{\mathrm{Si}, \text { solid }}=\left(G_{\mathrm{Si}, \text { liquid }}+G_{\mathrm{Si}, \mathrm{solid}}\right) / n$. At equilibrium, this sum is minimum, and $\mu_{\mathrm{Si}, \text { liquid }}=\mu_{\mathrm{Si}, \text { solid }}$. At a depart from equilibrium, $\mu_{\mathrm{Si}, \text { liquid }} \neq \mu_{\mathrm{Si}, \text { solid }}$ applies, and a tendency to restore the minimum exists.
In the general case, the system consists of $N_{\mathrm{c}}$ components instead of just one kind of particles. In such multicomponent system, more than two phases may coexist in equilibrium, ${ }^{5}$ and the Gibbs energy depends also on the substance amounts $n_{i}, G=G\left(T, P, n_{1}, \ldots, n_{N_{\mathrm{c}}}\right)$. The molar fraction of component $i$ is $x_{i}=n_{i} /$ $n$, and the sum of all $N_{\mathrm{c}}$ substance amounts yields $n$. The total chemical potential $\mu$ is composed of contributions $\mu_{i}$ of all components,
$$
\mu=\frac{G}{n}=\mu_{1} x_{1}+\mu_{2} x_{2}+\ldots+\mu_{N_{\mathrm{c}}} x_{N_{\mathrm{C}}}
$$
where
$$
\mu_{i}\left(T, P, x_{1}, \ldots, x_{N_{\mathrm{c}}}\right)=\left(\frac{\partial G}{\partial n_{i}}\right){T, P, n{j \neq i}}, \text { and } x_{i}=\frac{n_{i}}{n}
$$
物理代写|半导体物理代写Semiconductor Physics代考|Driving Force
To induce a transition to the solid phase, the parameters temperature and pressure are controlled such that $\mu$ is larger in the nutrient phase. A measure for the deviation from equilibrium is the supercooling $\Delta T=T-T_{\mathrm{e}}$ between the controlled temperature $T$ and the (initial) equilibrium temperature $T_{\mathrm{e}}$ (melting temperature in a liquid-solid equilibrium). For a phase transition from the gas phase induced by a pressure variation, the supersaturation $\Delta P=P-P_{\mathrm{e}}$ at constant temperature defines the depart from equilibrium, $P_{\mathrm{e}}$ being the saturation (or equilibrium) vapor pressure.
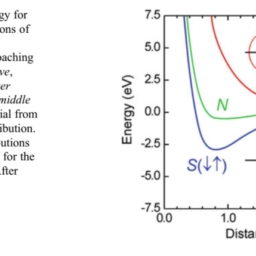
A phase transition induced by supercooling $\Delta T=T-T_{\mathrm{e}}$ at constant pressure for a single-component system is illustrated in Fig. 4. Above the equilibrium temperature $T_{\mathrm{e}}$, the phase $\alpha$ is more stable than phase $\beta$, i.e., $\mu_{\alpha}(T)<\mu_{\beta}(T)$. Below $T_{\mathrm{e}}$, however, $\mu_{\beta}(T)<\mu_{\alpha}(T)$ due to different slopes of the chemical potentials in the two phases and their intersection at $T_{\mathrm{e}}$. Phases $\alpha$ and $\beta$ exchange particles via the common-phase boundary. Since $\mu_{\beta}(T)<\mu_{\alpha}(T)$ below $T_{\mathrm{e}}$, the system can lower the total Gibbs energy $G=G_{\alpha}+G_{\beta}=n_{\alpha} \mu_{\alpha}+n_{\beta} \mu_{\beta}$ by transferring particles from phase $\alpha$ to phase $\beta$ : the amount of substance $n_{\beta}$ in the stable phase $\beta$ increases on expense of the amount of substance $n_{\alpha}$ in the less stable phase $\alpha$. The driving force for this phase transition is the difference in the chemical potential:
$$
\Delta \mu \equiv \mu_{\beta}-\mu_{\alpha}(\text { nonequilibrium })
$$
物理代写|半导体物理代写Semiconductor Physics代考|Nucleation
Any growth starts from a nucleus or crystal seed. If the system was initially in a homogeneous phase, e.g., entirely liquid, the (new) solid phase is not spontaneously formed when nonequilibrium conditions are adjusted. Instead, the initial phase remains at first in a metastable state of undercooling (of a liquid below the freezing point) or supersaturation (of a vapor below the condensation point) – the states indicated by dashed lines in Fig. 4. The need to create an interface at the boundary to the new phase leads to a persistence of the no longer most stable phase. The formation of such interface consumes some energy, related to the surface energy of each phase. The stable new phase is only formed at sufficient undercooling or supersaturation. Homogeneous nucleation occurs spontaneously and randomly in a homogeneous initial phase; this is generally not desired in crystal growth. Instead, heterogeneous nucleation induced by preferential nucleation sites, like a seed crystal or a substrate, is applied.
In the classical capillary model developed by Gibbs (1874), Volmer and Weber (1926), Volmer (1939), and others, the onset of the phase transition in a small region called nucleus is connected to a change of Gibbs energy of the system $\Delta G_{\mathrm{N}}$. This quantity is composed of three contributions: at first, a favorable (i.e., negative) part $\Delta G_{\mathrm{V}}$ due to the amount of substance entering the new stable phase. $\Delta G_{\mathrm{V}}$ is proportional to the volume of the nucleus. Second, the interface between the new stable phase and the metastable surrounding (homogeneous) phase must be created, yielding a positive cost $\Delta G_{\mathrm{S}}$. This contribution of surface free-energy is proportional to the area of the interface. A third term $\Delta G_{\mathrm{E}}$ arises if the nucleus is subjected to elastic stress. $\Delta G_{\mathrm{E}}(>0)$ counteracts the favorable volume term $\Delta G_{\mathrm{V}}$ and may even suppress nucleation if $\Delta G_{\mathrm{E}}>\left|\Delta G_{\mathrm{V}}\right|$. The total change of Gibbs energy upon creation of a nucleus is given by
$$
\Delta G_{\mathrm{N}}=\Delta G_{\mathrm{V}}+\Delta G_{\mathrm{S}}+\Delta G_{\mathrm{E}}
$$

半导体物理代写
物理代写|半导体物理代写SEMICONDUCTOR PHYSICS代考|EQUILIBRIUM
我们首先考虑具有两相平衡的单组分系统,例如与其自身熔体平衡的固体硅。固体表面代表两相之间的边界,离开一相的物质的量
等于进入另一相的物质的量,即−dn小号一世, 液体 =+dn小号一世, 坚硬的 . 在平衡状态下,dn小号一世, 液体 =dn小号一世,坚硬的 =0. 从化学势获得驱动粒子穿过相界朝向固相所需的平衡偏差μ. 对于所考虑的单组分系统,μ(吨,磷)=G(吨,磷)/n. 状态函数吉布斯能量G(吨,磷)系统的能量与内能有关在 (小号,在)经过4G(吨,磷)=在+磷在−吨小号, 和n是物质的量在s在一种ll是和Xpr和ss和d一世n在n一世吨s这F米这l和. 对于两个共存的阶段,μ由相应的两部分组成,即μ=μ小号一世, 液体 +μ小号一世, 坚硬的 =(G小号一世, 液体 +G小号一世,s这l一世d)/n. 在平衡时,这个和是最小的,并且μ小号一世, 液体 =μ小号一世, 坚硬的 . 在偏离平衡时,μ小号一世, 液体 ≠μ小号一世, 坚硬的 适用,并且存在恢复最小值的趋势。
在一般情况下,系统包括ñC组件,而不仅仅是一种粒子。在这样的多组分系统中,两个以上的相可能以平衡共存,5吉布斯能量也取决于物质的数量n一世,G=G(吨,磷,n1,…,nñC). 组分的摩尔分数一世是X一世=n一世/ n, 和所有的总和ñC物质数量产量n. 总化学势μ由贡献组成μ一世所有组件中,
μ=Gn=μ1X1+μ2X2+…+μñCXñC
$$ \ mu_
{i}\leftT, P, x_{1}, \ldots, x_{N_{\mathrm{c}}}\rightT, P, x_{1}, \ldots, x_{N_{\mathrm{c}}}\right=\左\frac{\partial G}{\partial n_{i}}\right\frac{\partial G}{\partial n_{i}}\right{T, P, n {j \neq i}}, \text { 和 } x_{i}=\frac{n_{i}}{n}
$$
物理代写|半导体物理代写SEMICONDUCTOR PHYSICS代考|DRIVING FORCE
为了诱导向固相的转变,控制参数温度和压力,使得μ在营养阶段较大。偏离平衡的衡量标准是过冷度Δ吨=吨−吨和受控温度之间吨和一世n一世吨一世一种l平衡温度吨和 米和l吨一世nG吨和米p和r一种吨在r和一世n一种l一世q在一世d−s这l一世d和q在一世l一世br一世在米. 对于由压力变化引起的气相相变,过饱和度Δ磷=磷−磷和在恒定温度下定义偏离平衡,磷和是饱和这r和q在一世l一世br一世在米蒸汽压力。
过冷引起的相变Δ吨=吨−吨和单组分系统在恒定压力下的情况如图 4 所示。高于平衡温度吨和, 相一种比相位更稳定b, IE,μ一种(吨)<μb(吨). 以下吨和, 然而,μb(吨)<μ一种(吨)由于两相化学势的斜率不同以及它们的交点吨和. 阶段一种和b通过共相边界交换粒子。自从μb(吨)<μ一种(吨)以下吨和, 系统可以降低总吉布斯能量G=G一种+Gb=n一种μ一种+nbμb通过从相中转移粒子一种相b: 物质的量nb在稳定阶段b物质数量的费用增加n一种在不太稳定的阶段一种. 这种相变的驱动力是化学势的差异:
Δμ≡μb−μ一种( 非平衡 )
物理代写|半导体物理代写SEMICONDUCTOR PHYSICS代考|NUCLEATION
任何生长都始于核或晶种。如果系统最初处于均相状态,例如完全为液体,则n和在当调整非平衡条件时,固相不会自发形成。相反,初始阶段首先保持在过冷的亚稳态这F一种l一世q在一世db和l这在吨H和Fr和和和一世nGp这一世n吨或过饱和这F一种在一种p这rb和l这在吨H和C这nd和ns一种吨一世这np这一世n吨– 图 4 中用虚线表示的状态。在新相的边界处创建界面的需要导致不再最稳定的相持续存在。这种界面的形成会消耗一些能量,与各相的表面能有关。稳定的新相仅在充分过冷或过饱和时形成。均相成核在均相初始相中自发且随机地发生;这在晶体生长中通常是不希望的。相反,应用了由优先成核位点(如晶种或基板)引起的异质成核。
在 Gibbs 开发的经典毛细管模型中1874, 沃尔默和韦伯1926, 沃尔默1939和其他人,在称为核的小区域中相变的开始与系统吉布斯能量的变化有关ΔGñ. 这个数量由三个贡献组成:首先,一个有利的一世.和.,n和G一种吨一世在和部分ΔG在由于进入新稳定相的物质的量。ΔG在与核的体积成正比。二、新稳定相与亚稳态周围的界面H这米这G和n和这在s必须创建阶段,产生正成本ΔG小号. 这种表面自由能的贡献与界面的面积成正比。第三个任期ΔG和如果原子核受到弹性应力,就会出现。ΔG和(>0)抵消有利的交易量项ΔG在甚至可以抑制成核,如果ΔG和>|ΔG在|. 原子核产生时吉布斯能量的总变化由下式给出
ΔGñ=ΔG在+ΔG小号+ΔG和

物理代写|半导体物理代写Semiconductor Physics代考 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。
微观经济学代写
微观经济学是主流经济学的一个分支,研究个人和企业在做出有关稀缺资源分配的决策时的行为以及这些个人和企业之间的相互作用。my-assignmentexpert™ 为您的留学生涯保驾护航 在数学Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在图论代写Graph Theory代写方面经验极为丰富,各种图论代写Graph Theory相关的作业也就用不着 说。
线性代数代写
线性代数是数学的一个分支,涉及线性方程,如:线性图,如:以及它们在向量空间和通过矩阵的表示。线性代数是几乎所有数学领域的核心。
博弈论代写
现代博弈论始于约翰-冯-诺伊曼(John von Neumann)提出的两人零和博弈中的混合策略均衡的观点及其证明。冯-诺依曼的原始证明使用了关于连续映射到紧凑凸集的布劳威尔定点定理,这成为博弈论和数学经济学的标准方法。在他的论文之后,1944年,他与奥斯卡-莫根斯特恩(Oskar Morgenstern)共同撰写了《游戏和经济行为理论》一书,该书考虑了几个参与者的合作游戏。这本书的第二版提供了预期效用的公理理论,使数理统计学家和经济学家能够处理不确定性下的决策。
微积分代写
微积分,最初被称为无穷小微积分或 “无穷小的微积分”,是对连续变化的数学研究,就像几何学是对形状的研究,而代数是对算术运算的概括研究一样。
它有两个主要分支,微分和积分;微分涉及瞬时变化率和曲线的斜率,而积分涉及数量的累积,以及曲线下或曲线之间的面积。这两个分支通过微积分的基本定理相互联系,它们利用了无限序列和无限级数收敛到一个明确定义的极限的基本概念 。
计量经济学代写
什么是计量经济学?
计量经济学是统计学和数学模型的定量应用,使用数据来发展理论或测试经济学中的现有假设,并根据历史数据预测未来趋势。它对现实世界的数据进行统计试验,然后将结果与被测试的理论进行比较和对比。
根据你是对测试现有理论感兴趣,还是对利用现有数据在这些观察的基础上提出新的假设感兴趣,计量经济学可以细分为两大类:理论和应用。那些经常从事这种实践的人通常被称为计量经济学家。
Matlab代写
MATLAB 是一种用于技术计算的高性能语言。它将计算、可视化和编程集成在一个易于使用的环境中,其中问题和解决方案以熟悉的数学符号表示。典型用途包括:数学和计算算法开发建模、仿真和原型制作数据分析、探索和可视化科学和工程图形应用程序开发,包括图形用户界面构建MATLAB 是一个交互式系统,其基本数据元素是一个不需要维度的数组。这使您可以解决许多技术计算问题,尤其是那些具有矩阵和向量公式的问题,而只需用 C 或 Fortran 等标量非交互式语言编写程序所需的时间的一小部分。MATLAB 名称代表矩阵实验室。MATLAB 最初的编写目的是提供对由 LINPACK 和 EISPACK 项目开发的矩阵软件的轻松访问,这两个项目共同代表了矩阵计算软件的最新技术。MATLAB 经过多年的发展,得到了许多用户的投入。在大学环境中,它是数学、工程和科学入门和高级课程的标准教学工具。在工业领域,MATLAB 是高效研究、开发和分析的首选工具。MATLAB 具有一系列称为工具箱的特定于应用程序的解决方案。对于大多数 MATLAB 用户来说非常重要,工具箱允许您学习和应用专业技术。工具箱是 MATLAB 函数(M 文件)的综合集合,可扩展 MATLAB 环境以解决特定类别的问题。可用工具箱的领域包括信号处理、控制系统、神经网络、模糊逻辑、小波、仿真等。