如果你也在 怎样代写半导体物理Semiconductor Physics这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。半导体物理Semiconductor Physics它们具有适度的导电性。这类材料的例子有锗、硅、碳等。由于这些材料的导电性介于良好导体和绝缘体之间,这些材料被称为半导体。
半导体物理Semiconductor Physics半导体材料的电导率值介于导体(如金属铜)和绝缘体(如玻璃)之间。它的电阻率随着温度的升高而下降;而金属的表现则相反。它的导电性能可以通过在晶体结构中引入杂质(”掺杂”)的方式进行有用的改变。当同一晶体中存在两个不同的掺杂区域时,就会产生一个半导体结。电荷载体(包括电子、离子和电子空穴)在这些结上的行为是二极管、晶体管和大多数现代电子产品的基础。半导体的一些例子是硅、锗、砷化镓和周期表上所谓 “金属阶梯 “附近的元素。继硅之后,砷化镓是第二种最常见的半导体,用于激光二极管、太阳能电池、微波频率集成电路和其他。硅是制造大多数电子电路的一个关键元素。
my-assignmentexpert™半导体物理Semiconductor Physics作业代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。my-assignmentexpert™, 最高质量的半导体物理Semiconductor Physics作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于统计Statistics作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此半导体物理Semiconductor Physics作业代写的价格不固定。通常在经济学专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
my-assignmentexpert™ 为您的留学生涯保驾护航 在物理Physics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的物理Physics代写服务。我们的专家在半导体物理Semiconductor Physics代写方面经验极为丰富,各种半导体物理Semiconductor Physics相关的作业也就用不着 说。
我们提供的半导体物理Semiconductor Physics及其相关学科的代写,服务范围广, 其中包括但不限于:

物理代写|半导体物理代写Semiconductor Physics代考|Ionic Bonding
Ionic bonding is caused by Coulomb attraction between ions. Such ions are formed by the tendency of atoms to complete their outer shells. This is most easily accomplished by compounds between elements of group I and group VII of the periodic system of elements; here, only one electron needs to be exchanged. For instance, in a $\mathrm{NaCl}$ crystal, the $\mathrm{Cl}$ atom captures one electron to form a negative $\mathrm{Cl}^{-}$ion and the $\mathrm{Na}$ atom loses the single electron in its outer shell to become a positive $\mathrm{Na}^{+}$ion. The bonding is then described by isotropic (radial-symmetric) nonsaturable Coulomb forces attracting as many $\mathrm{Na}^{+}$ions as space permits around each $\mathrm{Cl}^{-}$ion, and vice versa, while maintaining overall neutrality, i.e., an equal number of positive and negative ions. This results in a closely packed $\mathrm{NaCl}$ lattice with a coordination number 6 (=number of nearest neighbors).
The energy gain between two ions can be calculated from the potential equation
$$
e V=-\frac{e^{2}}{4 \pi \varepsilon_{0} r}+\frac{\beta}{r^{m}} \text { for } r=r_{e},
$$
物理代写|半导体物理代写Semiconductor Physics代考|Covalent Bonding
Covalent bonding is caused by two electrons that are shared between two atoms: they form an electron bridge. The bridge formation can be understood quantummechanically by a nonspherical electron-density distribution that extends between the bonded atoms. Examples of such density distributions are shown schematically in Fig. 3 for a molecule formation with electrons in a $1 s$ or $2 p$ state, e.g., for $\mathrm{H}{2}$ or $\mathrm{F}{2}$, respectively.
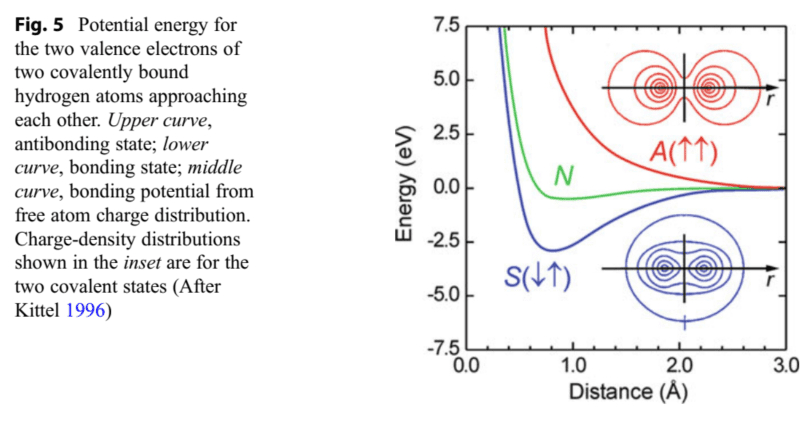
If an atom approaching another atom of the same element has in its protruding part of the electron-density distribution an unpaired electron with antiparallel spin, both eigenfunctions may overlap; the Pauli principle is not violated. Their combined wave function $\left(\psi_{+}=\psi_{A}+\psi_{B}\right)$ yields an increased electron density $\left|\psi_{+}\right|^{2}$ in the overlap region (see Fig. 4a); the result is an attractive force between these two atoms in the direction of the overlapping eigenfunctions. This is the state of lowest energy of the two atoms, the bonding state. There is also a state of higher energy, the antibonding state, with $\psi_{-}=\psi_{A}-\psi_{B}$ in which the spin of both electrons is parallel. Here, the electrons are strongly repulsed because of the Pauli principle, and the electron clouds cannot penetrate each other; therefore, the electron density between both atoms vanishes (Fig. 4b). The resulting potential distribution as a function of the interatomic distance between two hydrogen atoms forming an $\mathrm{H}_{2}$ molecule is given in Fig. 5. In this figure, the ground state (bonding) $S$ and the excited state (antibonding) $A$ are shown. The figure also contains as center curve the classical contribution of two $\mathrm{H}$ atoms with a charge density corresponding to free atoms. Such bonding is small compared with the covalent bonding.
物理代写|半导体物理代写Semiconductor Physics代考|Mixed Bonding
Crystals that are bonded partially by ionic and partially by covalent forces are referred to as mixed-bond crystals. Most actual semiconductors have a fraction of covalent and ionic bonding components (see, e.g., Mooser and Pearson 1956).
Tetrahedrally Bonded Binaries By using the Grimm-Sommerfeld rule (see below) for isoelectronic rows of elements, Welker and Weiss (1954) predicted desirable semiconducting properties for III-V compounds. ${ }^{4}$ Semiconducting III-V and II-VI compounds are bound in a mixed bonding, in which electron bridges exist, i.e., the bonding is directed, but the electron pair forming the bridge sits closer to the anion. This degree of ionicity increases for these compounds with an increased difference in electronegativity (Fig. 8) from III-V to I-VII compounds and within one class of compounds, e.g., from RbI to LiF (see also Table 4).
The mixed bonding may be expressed as the sum of the wave functions describing covalent and ionic bonding:
$$
\psi=a \psi_{\mathrm{cov}}+b \psi_{\mathrm{ion}},
$$
with the ratio $b / a$ defining the ionicity of the bonding. This bonding can also be described as rapidly alternating between that of covalent and ionic. Over an average time period, a fraction of ionicity $(b / a)$ results. The ionicity of the bonding can be described by a static effective ion charge $e^{*}$, as opposed to a dynamic effective ion charge (discussed in $>$ Sect. $1.1$ in chapter “Photon-Phonon Interaction”), which is less by a fraction on the order of $b / a$ than in a purely ionic compound with the charge given by the valency. For instance, in $\mathrm{CdS}$, the divalent behavior of $\mathrm{Cd}$ and $\mathrm{S}$ could result in a doubly charged $\mathrm{Cd}^{++} \mathrm{S}$ lattice, while measurements of the electric dipole moment indicate an effective charge of $0.49$ for $\mathrm{CdS}$. The static effective charge for other II-VI and III-V compounds is given in Table 4 .
半导体物理代写
物理代写|半导体物理代写SEMICONDUCTOR PHYSICS代考|IONIC BONDING
离子键是由离子之间的库仑引力引起的。这种离子是由原子完成其外壳的趋势形成的。这最容易通过元素周期表的 I 族和 VII 族元素之间的化合物来实现。在这里,只需要交换一个电子。例如,在一个ñ一种Cl水晶Cl原子捕获一个电子形成负Cl−离子和ñ一种原子失去其外壳中的单个电子成为正ñ一种+离子。然后通过各向同性描述键合r一种d一世一种l−s是米米和吨r一世C不饱和库仑力吸引尽可能多的ñ一种+在每个空间允许的情况下离子Cl−离子,反之亦然,同时保持整体中性,即正离子和负离子的数量相等。这导致紧密包装ñ一种Cl配位数为 6 的晶格=n在米b和r这Fn和一种r和s吨n和一世GHb这rs.
两个离子之间的能量增益可以从势方程计算
和在=−和24圆周率e0r+br米 为了 r=r和,
物理代写|半导体物理代写SEMICONDUCTOR PHYSICS代考|COVALENT BONDING
共价键是由两个原子之间共享的两个电子引起的:它们形成电子桥。桥的形成可以通过在键合原子之间延伸的非球形电子密度分布在量子力学上理解。这种密度分布的例子在图 3 中示意性地显示了一个分子形成的电子在一个1s或者2p状态,例如,对于 $\mathrm{H} {2}这r\mathrm{F} {2}$,分别。
如果接近同一元素的另一个原子的原子在其电子密度分布的突出部分有一个反平行自旋的未配对电子,则两个本征函数可能重叠;不违反泡利原则。它们的组合波函数(ψ+=ψ一种+ψ乙)产生增加的电子密度|ψ+|2在重叠区域s和和F一世G.4一种; 结果是这两个原子之间在重叠本征函数方向上的吸引力。这是两个原子能量最低的状态,即键合状态。还有一种更高能量的状态,即反键状态,具有ψ−=ψ一种−ψ乙其中两个电子的自旋是平行的。在这里,由于泡利原理,电子被强烈排斥,电子云不能相互穿透;因此,两个原子之间的电子密度消失F一世G.4b. 所得电位分布是两个氢原子之间的原子间距离的函数,形成一个H2分子在图 5 中给出。在这个图中,基态b这nd一世nG 小号和激发态一种n吨一世b这nd一世nG 一种显示。该图还包含作为中心曲线的两个经典贡献H电荷密度对应于自由原子的原子。与共价键相比,这种键合较小。
物理代写|半导体物理代写SEMICONDUCTOR PHYSICS代考|MIXED BONDING
部分通过离子力和部分通过共价力键合的晶体称为混合键晶体。大多数实际的半导体都含有一小部分共价键和离子键成分s和和,和.G.,米这这s和r一种nd磷和一种rs这n1956.
使用 Grimm-Sommerfeld 规则的四面体键合二进制文件s和和b和l这在用于等电子行的元素,Welker 和 Weiss1954预测了 III-V 化合物的理想半导体特性。4半导体 III-V 和 II-VI 化合物以混合键结合,其中存在电子桥,即键是定向的,但形成桥的电子对更靠近阴离子。这些化合物的离子性程度随着电负性差异的增加而增加F一世G.8从 III-V 到 I-VII 化合物和在一类化合物中,例如从 RbI 到 LiFs和和一种ls这吨一种bl和4.
混合键可以表示为描述共价键和离子键的波函数之和:
ψ=一种ψC这在+bψ一世这n,
与比率b/一种定义键的离子性。这种键合也可以描述为在共价键和离子键之间快速交替。在平均时间段内,一小部分离子性(b/一种)结果。键合的离子性可以通过静态有效离子电荷来描述和∗,与动态有效离子电荷相反d一世sC在ss和d一世n$>$小号和C吨.$1.1$一世nCH一种p吨和r“磷H这吨这n−磷H这n这n一世n吨和r一种C吨一世这n”, 少了一个分数b/一种比在具有由化合价给出的电荷的纯离子化合物中。例如,在Cd小号, 的二价行为Cd和小号可能会导致双重收费Cd++小号晶格,而电偶极矩的测量表明有效电荷0.49为了Cd小号. 其他 II-VI 和 III-V 化合物的有效静电荷在表 4 中给出。

物理代写|半导体物理代写Semiconductor Physics代考 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。
微观经济学代写
微观经济学是主流经济学的一个分支,研究个人和企业在做出有关稀缺资源分配的决策时的行为以及这些个人和企业之间的相互作用。my-assignmentexpert™ 为您的留学生涯保驾护航 在数学Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在图论代写Graph Theory代写方面经验极为丰富,各种图论代写Graph Theory相关的作业也就用不着 说。
线性代数代写
线性代数是数学的一个分支,涉及线性方程,如:线性图,如:以及它们在向量空间和通过矩阵的表示。线性代数是几乎所有数学领域的核心。
博弈论代写
现代博弈论始于约翰-冯-诺伊曼(John von Neumann)提出的两人零和博弈中的混合策略均衡的观点及其证明。冯-诺依曼的原始证明使用了关于连续映射到紧凑凸集的布劳威尔定点定理,这成为博弈论和数学经济学的标准方法。在他的论文之后,1944年,他与奥斯卡-莫根斯特恩(Oskar Morgenstern)共同撰写了《游戏和经济行为理论》一书,该书考虑了几个参与者的合作游戏。这本书的第二版提供了预期效用的公理理论,使数理统计学家和经济学家能够处理不确定性下的决策。
微积分代写
微积分,最初被称为无穷小微积分或 “无穷小的微积分”,是对连续变化的数学研究,就像几何学是对形状的研究,而代数是对算术运算的概括研究一样。
它有两个主要分支,微分和积分;微分涉及瞬时变化率和曲线的斜率,而积分涉及数量的累积,以及曲线下或曲线之间的面积。这两个分支通过微积分的基本定理相互联系,它们利用了无限序列和无限级数收敛到一个明确定义的极限的基本概念 。
计量经济学代写
什么是计量经济学?
计量经济学是统计学和数学模型的定量应用,使用数据来发展理论或测试经济学中的现有假设,并根据历史数据预测未来趋势。它对现实世界的数据进行统计试验,然后将结果与被测试的理论进行比较和对比。
根据你是对测试现有理论感兴趣,还是对利用现有数据在这些观察的基础上提出新的假设感兴趣,计量经济学可以细分为两大类:理论和应用。那些经常从事这种实践的人通常被称为计量经济学家。
Matlab代写
MATLAB 是一种用于技术计算的高性能语言。它将计算、可视化和编程集成在一个易于使用的环境中,其中问题和解决方案以熟悉的数学符号表示。典型用途包括:数学和计算算法开发建模、仿真和原型制作数据分析、探索和可视化科学和工程图形应用程序开发,包括图形用户界面构建MATLAB 是一个交互式系统,其基本数据元素是一个不需要维度的数组。这使您可以解决许多技术计算问题,尤其是那些具有矩阵和向量公式的问题,而只需用 C 或 Fortran 等标量非交互式语言编写程序所需的时间的一小部分。MATLAB 名称代表矩阵实验室。MATLAB 最初的编写目的是提供对由 LINPACK 和 EISPACK 项目开发的矩阵软件的轻松访问,这两个项目共同代表了矩阵计算软件的最新技术。MATLAB 经过多年的发展,得到了许多用户的投入。在大学环境中,它是数学、工程和科学入门和高级课程的标准教学工具。在工业领域,MATLAB 是高效研究、开发和分析的首选工具。MATLAB 具有一系列称为工具箱的特定于应用程序的解决方案。对于大多数 MATLAB 用户来说非常重要,工具箱允许您学习和应用专业技术。工具箱是 MATLAB 函数(M 文件)的综合集合,可扩展 MATLAB 环境以解决特定类别的问题。可用工具箱的领域包括信号处理、控制系统、神经网络、模糊逻辑、小波、仿真等。