如果你也在 怎样代写数值方法numerical methods这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。数值方法numerical methods在数值分析中,数值方法是一种旨在解决数值问题的数学工具。在编程语言中,带有适当收敛检查的数值方法的实现被称为数值算法。
数值方法numerical methods数值分析是研究使用数值逼近(相对于符号操作)来解决数学分析问题的算法(有别于离散数学)。数值分析在工程和物理科学的所有领域都有应用,在21世纪还包括生命科学和社会科学、医学、商业甚至艺术领域。目前计算能力的增长使得更复杂的数值分析得以使用,在科学和工程中提供详细和现实的数学模型。数值分析的例子包括:天体力学中的常微分方程(预测行星、恒星和星系的运动),数据分析中的数值线性代数,以及用于模拟医学和生物学中活细胞的随机微分方程和马尔科夫链。
my-assignmentexpert™数值方法numerical methods作业代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。my-assignmentexpert™, 最高质量的数值方法numerical methods作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于统计Statistics作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此数值方法numerical methods作业代写的价格不固定。通常在经济学专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
my-assignmentexpert™ 为您的留学生涯保驾护航 在数学Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在数值方法numerical methods代写方面经验极为丰富,各种数值方法numerical methods相关的作业也就用不着 说。
我们提供的数值方法numerical methods及其相关学科的代写,服务范围广, 其中包括但不限于:
数学代写|数值方法作业代写numerical methods代考|The Exact Solution
Theoretical models are built not only to analyze a variety of positive and normative issues, but also to be confronted with actual data, in an attempt to validate their implications. The continuous-time version of the Solow-Swan model can be used to produce time series for physical capital, output, consumption and investment by sampling at discrete points in time, from the continuous time processes obtained from (2.30) and the implied expressions for the remaining variables. Discrete sampling amounts to giving discrete values: $t=1,2,3, \ldots$ to the time index in those expressions. This apparently innocuous procedure is subject, however, to potential pitfalls, that will be illustrated numerically in the next chapter.
An alternative method consists on analyzing directly the discrete version of the Solow-Swan model. To do so, we could think of directly translating the law of motion into discrete time by substituting a time difference $k_{t+1}-k_{t}$ for the time derivative $\dot{k}{t}$, like in: $$ k{t+1}-k_{t}=s f\left(k_{t}\right)-(n+\delta+\gamma) k_{t}
$$
数学代写|数值方法作业代写numerical methods代考|Approximate Solutions to the Discrete-Time Model
As the continuous-time model, the discrete-time version of the Solow-Swan economy can be solved exactly through the use of (2.36), as we will show in a section below. That is an exception, since nonlinearities in growth models will usually preclude the existence of an exact solution. To familiarize the reader with that practice, we proceed in this section to obtain the solution to the linear and the quadratic approximations to the model.
Considering the nonlinear difference equation in (2.36) as a function $k_{t+1}=$ $\Psi\left(k_{t} ; \theta\right)$ and using Taylor’s expansion and (2.38), the linear approximation to that equation around steady-state is,
$$
\begin{aligned}
k_{t+1}-k_{s s}=& \Psi\left(k_{s s}\right)+\left(\frac{\partial \Psi\left(k_{t} ; \theta\right)}{\partial k_{t}}\right){s s}\left(k{t}-k_{s s}\right) \
\Rightarrow k_{t+1} \simeq &\left(\frac{1}{(1+n)(1+\gamma)} f\left(k_{s s}\right)+\frac{1-\delta}{(1+n)(1+\gamma)} k_{s s}\right) \
&+\left(\frac{1}{(1+n)(1+\gamma)} s f^{\prime}\left(k_{s s}\right)+\frac{1-\delta}{(1+n)(1+\gamma)}\right)\left(k_{t}-k_{s s}\right) \
=& k_{s s}+\frac{s f^{\prime}\left(k_{s s}\right)+(1-\delta)}{(1+n)(1+\gamma)}\left(k_{t}-k_{s s}\right)
\end{aligned}
$$
数学代写|数值方法作业代写NUMERICAL METHODS代考|Numerical Exercise: Solving the Deterministic Solow–Swan Model
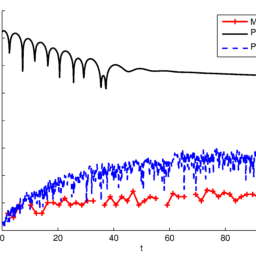
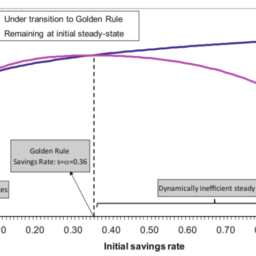
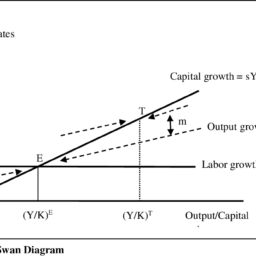
In the Discrete spreadsheet in the Solow_deterministic.xls file, time series are obtained for a deterministic, discrete-time version of the Solow-Swan economy from an initial capital stock of $k_{0}=20$. Aggregate technology is supposed to be of the Cobb-Douglas type, with a capital share of $\alpha=0.36$, and a technological constant $A=5.0$. Depreciation of physical capital is $\delta=7.5 \%$, savings are $36.0 \%$ of output each period, and we assume zero population growth, $n=0$. Since the savings rate is equal to the output elasticity of capital, the steady-state in this economy will be the Golden Rule. ${ }^{16}$ With these parameter values, steady state levels turn out to be: $k_{s s}=117.94, y_{s s}=27.85, c_{s s}=17.82, s_{s s}=i_{s s}=10.02$. Therefore, the economy starts to the left of the steady-state, with a stock of capital well below the steady-state level. The constant savings rate is relatively high, and capital accumulates quickly because the level of savings initially exceeds from total depreciation expenditures. ${ }^{17}$ After 16 periods, the economy has covered half the initial distance to steady-state, with a stock of capital above 70 units. The Discrete spreadsheet presents time series for 260 periods, and the discrete time model is solved using the exact solution (2.36), as well as using the solutions to the linear and quadratic approximations $(2.43),(2.44)$ to the discrete-time model. The resulting time series for the stock of capital under the different approaches are reported in the first panel. The time series for output, savings and consumption that are obtained under the exact solution are shown in Panel 2, while Panels 3 and 4 display the similar time series obtained under the linear and quadratic approximations to the model. Notice that, according to the model, output is obtained each period from the stock of capital accumulated at the end of the previous period. As in subsequent exercises, this is organized in the spreadsheet by making output to be a function of the stock of capital in the previous row. That is, in the row corresponding to time $t$ we have $k_{t+1}$ and variables like $y_{t}, c_{t}$. (The same exercise can be reproduced by MATLAB file: Solow_stochastic.m by setting the variance parameter sigmae to zero.) Consumers’ preferences do not play any role in this exercise. Nevertheless, to familiarize the reader with the type of welfare evaluation that will often be performed in the next chapters, consumers are supposed to have a constant relative risk aversion utility function, $U\left(c_{t}\right)=\frac{c_{t}^{1-\sigma}-1}{1-\sigma}$, with risk aversion coefficient of $\sigma=3.0$, and a time discount factor $\beta=0.95$, and we compute single-period as well as time-aggregate, discounted utility.
数值方法代写
数学代写|数值方法作业代写NUMERICAL METHODS代考|THE EXACT SOLUTION
建立理论模型不仅是为了分析各种积极和规范的问题,而且还要面对实际数据,以试图验证它们的含义。Solow-Swan 模型的连续时间版本可用于通过在离散时间点采样,从从获得的连续时间过程中生成物质资本、产出、消费和投资的时间序列2.30以及其余变量的隐含表达式。离散采样相当于给出离散值:吨=1,2,3,…到这些表达式中的时间索引。然而,这个看似无害的过程存在潜在的陷阱,下一章将用数字来说明。
另一种方法是直接分析 Solow-Swan 模型的离散版本。为此,我们可以考虑通过代入时间差,将运动定律直接转化为离散时间ķ吨+1−ķ吨对于时间导数 $\dot{k} {t},l一世ķ和一世n:$ k {t+1}-k_{t}=sf\leftk_{t}\对k_{t}\对-n+d+Ck_{t}
$$
数学代写|数值方法作业代写NUMERICAL METHODS代考|APPROXIMATE SOLUTIONS TO THE DISCRETE-TIME MODEL
作为连续时间模型,Solow-Swan 经济的离散时间版本可以通过使用2.36,正如我们将在下面的部分中展示的那样。这是一个例外,因为增长模型中的非线性通常会排除精确解的存在。为了让读者熟悉这种做法,我们在本节中继续获得模型的线性和二次近似的解。
考虑非线性差分方程2.36作为一个函数ķ吨+1= Ψ(ķ吨;θ)并使用泰勒展开式和2.38,该方程在稳态附近的线性近似是,
$$
\begin{aligned}
k_{t+1}-k_{s s}=& \Psi\left(k_{s s}\right)+\left(\frac{\partial \Psi\left(k_{t} ; \theta\right)}{\partial k_{t}}\right){s s}\left(k{t}-k_{s s}\right) \
\Rightarrow k_{t+1} \simeq &\left(\frac{1}{(1+n)(1+\gamma)} f\left(k_{s s}\right)+\frac{1-\delta}{(1+n)(1+\gamma)} k_{s s}\right) \
&+\left(\frac{1}{(1+n)(1+\gamma)} s f^{\prime}\left(k_{s s}\right)+\frac{1-\delta}{(1+n)(1+\gamma)}\right)\left(k_{t}-k_{s s}\right) \
=& k_{s s}+\frac{s f^{\prime}\left(k_{s s}\right)+(1-\delta)}{(1+n)(1+\gamma)}\left(k_{t}-k_{s s}\right)
\end{aligned}
$$
数学代写|数值方法作业代写NUMERICAL METHODS代考|NUMERICAL EXERCISE: SOLVING THE DETERMINISTIC SOLOW–SWAN MODEL
在 Solow_deterministic.xls 文件的离散电子表格中,从初始资本存量中获得确定性离散时间版本的 Solow-Swan 经济的时间序列ķ0=20. 聚合技术应该属于 Cobb-Douglas 类型,资本份额为一种=0.36, 和一个技术常数一种=5.0. 有形资本的折旧是d=7.5%, 储蓄是36.0%每个时期的产出,我们假设人口增长为零,n=0. 由于储蓄率等于资本的产出弹性,这个经济中的稳态将是黄金法则。16使用这些参数值,稳态水平变为:ķss=117.94,是ss=27.85,Css=17.82,sss=一世ss=10.02. 因此,经济开始处于稳态的左侧,资本存量远低于稳态水平。恒定储蓄率相对较高,由于储蓄水平最初超过总折旧支出,因此资本迅速积累。17在 16 个时期之后,经济已经覆盖了最初距离稳定状态的一半,资本存量超过 70 个单位。离散电子表格呈现 260 个周期的时间序列,离散时间模型使用精确解法求解2.36,以及使用线性和二次近似的解(2.43),(2.44)离散时间模型。第一个面板报告了不同方法下资本存量的最终时间序列。面板 2 显示了在精确解下获得的产出、储蓄和消耗的时间序列,而面板 3 和 4 显示了在模型的线性和二次近似下获得的相似时间序列。请注意,根据该模型,每个时期的产出都是从上一时期末积累的资本存量中获得的。在随后的练习中,这是通过将输出作为前一行中资本存量的函数而在电子表格中进行组织的。即在时间对应的行中吨我们有ķ吨+1和变量像是吨,C吨. 吨H和s一种米和和X和rC一世s和C一种nb和r和pr这d在C和db是米一种吨大号一种乙F一世l和:小号这l这在s吨这CH一种s吨一世C.米b是s和吨吨一世nG吨H和在一种r一世一种nC和p一种r一种米和吨和rs一世G米一种和吨这和和r这.消费者的偏好在这个练习中没有任何作用。然而,为了让读者熟悉下一章经常进行的福利评估类型,消费者应该有一个恒定的相对风险厌恶效用函数,在(C吨)=C吨1−σ−11−σ, 风险厌恶系数为σ=3.0, 和时间折扣因子b=0.95,我们计算单期以及时间聚合的贴现效用。

数学代写|数值方法作业代写numerical methods代考 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。
微观经济学代写
微观经济学是主流经济学的一个分支,研究个人和企业在做出有关稀缺资源分配的决策时的行为以及这些个人和企业之间的相互作用。my-assignmentexpert™ 为您的留学生涯保驾护航 在数学Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在图论代写Graph Theory代写方面经验极为丰富,各种图论代写Graph Theory相关的作业也就用不着 说。
线性代数代写
线性代数是数学的一个分支,涉及线性方程,如:线性图,如:以及它们在向量空间和通过矩阵的表示。线性代数是几乎所有数学领域的核心。
博弈论代写
现代博弈论始于约翰-冯-诺伊曼(John von Neumann)提出的两人零和博弈中的混合策略均衡的观点及其证明。冯-诺依曼的原始证明使用了关于连续映射到紧凑凸集的布劳威尔定点定理,这成为博弈论和数学经济学的标准方法。在他的论文之后,1944年,他与奥斯卡-莫根斯特恩(Oskar Morgenstern)共同撰写了《游戏和经济行为理论》一书,该书考虑了几个参与者的合作游戏。这本书的第二版提供了预期效用的公理理论,使数理统计学家和经济学家能够处理不确定性下的决策。
微积分代写
微积分,最初被称为无穷小微积分或 “无穷小的微积分”,是对连续变化的数学研究,就像几何学是对形状的研究,而代数是对算术运算的概括研究一样。
它有两个主要分支,微分和积分;微分涉及瞬时变化率和曲线的斜率,而积分涉及数量的累积,以及曲线下或曲线之间的面积。这两个分支通过微积分的基本定理相互联系,它们利用了无限序列和无限级数收敛到一个明确定义的极限的基本概念 。
计量经济学代写
什么是计量经济学?
计量经济学是统计学和数学模型的定量应用,使用数据来发展理论或测试经济学中的现有假设,并根据历史数据预测未来趋势。它对现实世界的数据进行统计试验,然后将结果与被测试的理论进行比较和对比。
根据你是对测试现有理论感兴趣,还是对利用现有数据在这些观察的基础上提出新的假设感兴趣,计量经济学可以细分为两大类:理论和应用。那些经常从事这种实践的人通常被称为计量经济学家。
Matlab代写
MATLAB 是一种用于技术计算的高性能语言。它将计算、可视化和编程集成在一个易于使用的环境中,其中问题和解决方案以熟悉的数学符号表示。典型用途包括:数学和计算算法开发建模、仿真和原型制作数据分析、探索和可视化科学和工程图形应用程序开发,包括图形用户界面构建MATLAB 是一个交互式系统,其基本数据元素是一个不需要维度的数组。这使您可以解决许多技术计算问题,尤其是那些具有矩阵和向量公式的问题,而只需用 C 或 Fortran 等标量非交互式语言编写程序所需的时间的一小部分。MATLAB 名称代表矩阵实验室。MATLAB 最初的编写目的是提供对由 LINPACK 和 EISPACK 项目开发的矩阵软件的轻松访问,这两个项目共同代表了矩阵计算软件的最新技术。MATLAB 经过多年的发展,得到了许多用户的投入。在大学环境中,它是数学、工程和科学入门和高级课程的标准教学工具。在工业领域,MATLAB 是高效研究、开发和分析的首选工具。MATLAB 具有一系列称为工具箱的特定于应用程序的解决方案。对于大多数 MATLAB 用户来说非常重要,工具箱允许您学习和应用专业技术。工具箱是 MATLAB 函数(M 文件)的综合集合,可扩展 MATLAB 环境以解决特定类别的问题。可用工具箱的领域包括信号处理、控制系统、神经网络、模糊逻辑、小波、仿真等。