如果你也在 怎样代写声学Acoustics PHYS1500这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。声学Acoustics是物理学的一个分支,涉及气体、液体和固体中机械波的研究,包括振动、声音、超声和次声等主题。在声学领域工作的科学家是声学家,而在声学技术领域工作的人可能被称为声学工程师。声学的应用几乎存在于现代社会的各个方面,最明显的是音频和噪音控制行业。
声学Acoustics听力是动物世界中最关键的生存手段之一,而语言则是人类发展和文化中最独特的特征之一。因此,声学科学遍布人类社会的许多方面–音乐、医学、建筑、工业生产、战争等等。同样,鸣禽和青蛙等动物物种也将声音和听觉作为交配仪式或标记领土的一个关键因素。艺术、工艺、科学和技术相互激荡,推动了整体的发展,就像在许多其他知识领域一样。罗伯特-布鲁斯-林赛(Robert Bruce Lindsay)的 “声学之轮 “是对声学各领域的一个公认的概述。
声学Acoustics代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。最高质量的声学Acoustics作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此声学Acoustics作业代写的价格不固定。通常在专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
同学们在留学期间,都对各式各样的作业考试很是头疼,如果你无从下手,不如考虑my-assignmentexpert™!
my-assignmentexpert™提供最专业的一站式服务:Essay代写,Dissertation代写,Assignment代写,Paper代写,Proposal代写,Proposal代写,Literature Review代写,Online Course,Exam代考等等。my-assignmentexpert™专注为留学生提供Essay代写服务,拥有各个专业的博硕教师团队帮您代写,免费修改及辅导,保证成果完成的效率和质量。同时有多家检测平台帐号,包括Turnitin高级账户,检测论文不会留痕,写好后检测修改,放心可靠,经得起任何考验!
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
我们在物理Physical代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的物理Physical代写服务。我们的专家在声学Acoustics代写方面经验极为丰富,各种声学Acoustics相关的作业也就用不着说。
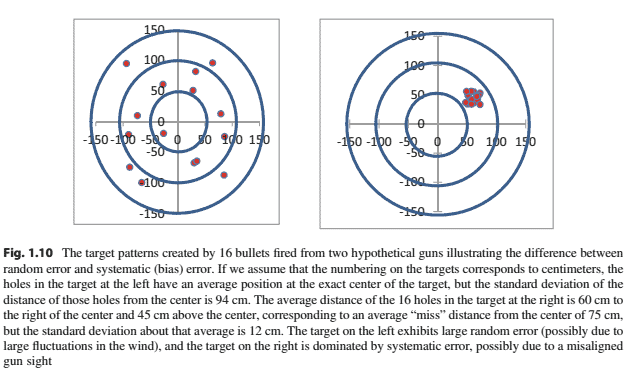
物理代写|声学代写Acoustics代考|Windscreen Noise
We will complete this section by examining an acoustical example that does not easily yield to conventional mathematical analysis. Windscreens are placed around microphones to reduce the noise that such microphones detect when they measure sound in the presence of flowing air (wind). Figure $1.8$ shows measurements of the sound pressure levels in one-third octave bands, $p_{1 / 3}$, as a function of the band-centered frequencies for air flow velocities from $2 \mathrm{~m} / \mathrm{s}$ to $14 \mathrm{~m} / \mathrm{s}$.
To apply our dimensional analysis, we first need to determine the relevant parameters. Some thought is required to select a physically significant set. Obviously, frequency, $f$; one-third octaveband pressure level, $p_{1 / 3}$; flow velocity, $v$; and windscreen diameter, $D$, seem to be potentially relevant. We also might include the properties of the fluid. Three possible candidates are the density, $\rho$; the sound speed, $c$; and the shear viscosity, $\mu$, of the flowing air. As we saw with the pendulum frequency, we can choose the wrong (or suboptimal) parameter set and still end up with a valid solution. Our practical interest is in understanding the noise pressure levels.
If we examine the first four parameters that are identified in Table 1.1, we notice that there is no other parameter that includes mass other than $p_{I / 3}$. To nondimensionalize $p_{I / 3}$, we have to include a fluid parameter that has units of mass. Since the flow speeds are much less than the sound speed (which does not contain units of mass) and we expect that the windscreen noise is generated by turbulence around the screen, I will select density rather than viscosity. ${ }^{23}$
We have identified five parameters that can be expressed using three basic units, so we will be able to form two dimensionless $\Pi$-groups: $(m-n)=5-3=2$. Again, since our interest is in the pressure levels at different frequencies, the choice suggested by the raw data plotted in Fig. $1.8$ is to create a dimensionless frequency, $\Pi_f$ and a dimensionless pressure, $\Pi_p$. For the calculation of $\Pi_f$, we know that the units for $f$ do not include mass, so we can eliminate $\rho$ from the $\Pi_f$ group.
$$
\Pi_f=f^a \nu^b D^c=\frac{1}{T^a} \frac{L^b}{T^b} L^c=T^0 L^0
$$
The length terms suggest $b+c=0$, or $b=-c$, and the time terms make $a+b=0$, so $a=-b=c=1$; hence $\Pi_f=f D / v$. This dimensionless group is known as the Strouhal number.
物理代写|声学代写Acoustics代考|Similitude Summary
Fourier’s requirement for dimensional homogeneity introduced dimensional constants that can provide sets of “natural units.” Such “derived units” lead to important restrictions on the functional form (to within a multiplicative constant) of mathematical expressions for the behavior of physical systems. We have used a formalism introduced by Buckingham [15] to extract those functions and to determine how many independent dimensionless groups can be formed that control the behavior of the physical system under the assumption that we have identified all the controlling parameters correctly. Although we may not be able to derive or even guess the form of the functions, we know that those functions can only depend on those dimensionless $\Pi$-groups.
Figure $1.9$ demonstrated how the use of $\Pi$-groups as the plotting variables can produce a universal relationship that unifies seemingly diverse sets of data, like those in Fig. 1.8, to produce a single correlation across a large variation of experimental parameters.
The $\Pi$-groups also provide guidance in the design and planning of experiments. Using the $\Pi_{\mathrm{f}_{-}}$ group, we could select flow velocities and windscreen diameters to optimize our variation of Strouhal number. The $\Pi_p$-group introduces the option of using gases of different densities to further increase the range of an experimental investigation, either by using less dense gases like $\mathrm{H}_2$ or He or a denser gas like $\mathrm{SF}_6$ (sulfur hexafluoride), in addition to air, or by changing the mean pressure to modify the density of a single gas.
Experiments are expensive! It is very valuable to be able to span the largest range of parameters with the fewest measurements.
Since the use of similitude is not commonly taught as a powerful technique for acoustics and vibration (although it is indispensable to the education of mechanical engineers in fields like fluid flow and heat transfer), the steps in determining П-groups will be summarized again below:
- List all of the parameters involved and their SI “base units.” An arrangement like Table $1.1$ can be useful.
- The difference between the number of parameters, $m$, and the required number of SI units, $n$, needed to express the $m$ parameters will determine the number of dimensionless $\Pi$-groups. The resultant number of $\Pi$-groups equals $(m-n)$.
- Select $n$ of the $m$ parameters you will use as the “natural units,” keeping in mind that you cannot use parameters that differ by only an exponent, for example, a base unit like length, $L$, and the volume, $L^3$.
- Express the other $(m-n)$ parameters, one at a time, in terms of the “natural units” using the requirement of dimensional homogeneity (e.g., Eqs. (1.78), (1.80), (1.81), (1.82), (1.85), and (1.86)) to produce $(m-n)$ dimensionless $\Pi$-groups.
- Check to be sure that the $\Pi$-groups you have formed are dimensionless.
声学代写
物理代写|声学代写声学代考|挡风玻璃噪音
.
我们将通过研究一个传统数学分析不容易得出结论的声学例子来结束本节。在麦克风周围放置挡风玻璃,以减少麦克风在流动空气(风)的存在下测量声音时所检测到的噪音。图$1.8$显示了三分之一八度频带$p_{1 / 3}$的声压级的测量结果,作为从$2 \mathrm{~m} / \mathrm{s}$到$14 \mathrm{~m} / \mathrm{s}$的空气流速带中心频率的函数。
要应用量纲分析,我们首先需要确定相关参数。选择一个物理上有意义的集合需要一些思考。显然,频率,$f$;三分之一倍频压力级,$p_{1 / 3}$;流速,$v$;挡风玻璃直径$D$似乎也有潜在的关联。我们也可以考虑流体的性质。三个可能的候选人是密度,$\rho$;声速,$c$;流动空气的剪切粘度$\mu$。正如我们看到的钟摆频率,我们可以选择错误的(或次优的)参数集,但仍然可以得到一个有效的解决方案。我们的实际兴趣是了解噪声压力水平
如果我们检查表1.1中确定的前四个参数,我们注意到除了$p_{I / 3}$外没有其他参数包含mass。为了使$p_{I / 3}$无量纲化,我们必须包含一个具有质量单位的流体参数。由于流动速度远小于声速(声速不包含质量单位),我们预计挡风玻璃噪音是由屏幕周围的湍流产生的,我将选择密度而不是粘度。${ }^{23}$
我们已经确定了五个可以用三个基本单位表示的参数,因此我们将能够形成两个无量纲的$\Pi$ -groups: $(m-n)=5-3=2$。同样,由于我们感兴趣的是不同频率下的压力水平,图$1.8$中绘制的原始数据建议的选择是创建一个无量次的频率$\Pi_f$和一个无量次的压力$\Pi_p$。对于$\Pi_f$的计算,我们知道$f$的单位不包括质量,所以我们可以从$\Pi_f$组中删除$\rho$。
$$
\Pi_f=f^a \nu^b D^c=\frac{1}{T^a} \frac{L^b}{T^b} L^c=T^0 L^0
$$
长度项建议$b+c=0$,或$b=-c$,时间项是$a+b=0$,所以$a=-b=c=1$;因此,$\Pi_f=f D / v$。这个无量纲群被称为Strouhal数
物理代写|声学代写Acoustics代考|相似摘要
傅里叶对维度同质性的要求引入了可以提供“自然单位”集合的维度常数。这样的“衍生单位”导致了对物理系统行为的数学表达式的函数形式(在乘法常数内)的重要限制。我们使用了Buckingham[15]引入的形式主义来提取这些函数,并确定在假设我们已经正确识别了所有控制参数的情况下,可以形成多少个独立的无量纲群来控制物理系统的行为。虽然我们可能无法推导或甚至猜测函数的形式,但我们知道,这些函数只能依赖于那些无量纲的$\Pi$ -groups.
图$1.9$展示了如何使用$\Pi$ -groups作为绘图变量,可以产生一个统一看似不同的数据集的普遍关系,如图1.8所示,从而在大量变化的实验参数中产生单一的相关性
$\Pi$ -groups还在实验的设计和计划中提供指导。使用$\Pi_{\mathrm{f}_{-}}$组,我们可以选择流速和挡风玻璃直径来优化我们的斯特劳哈尔数的变化。$\Pi_p$ -小组介绍了使用不同密度的气体来进一步扩大实验调查范围的选择,除了空气之外,使用密度较低的气体,如$\mathrm{H}_2$或He,或密度较高的气体,如$\mathrm{SF}_6$(六氟化硫),或通过改变平均压力来改变单一气体的密度
实验是昂贵的!能够以最少的测量值跨越最大的参数范围是非常有价值的
由于在声学和振动方面,通常不把相似性作为一种强有力的技术来教授(尽管它对流体流动和传热等领域的机械工程师的教育是必不可少的),确定П-groups的步骤将在下面再次总结
- 列出所有涉及的参数及其SI“基本单位”。像Table这样的排列 $1.1$ 可能会有用。
- 参数个数之差, $m$,以及所需的SI单元数量, $n$,需要表达的 $m$ 参数将决定无量纲数 $\Pi$-组。的总和 $\Pi$-groups = $(m-n)$.
- 选中 $n$ 的 $m$ 您将使用的参数作为“自然单位”,请记住,您不能使用只相差一个指数的参数,例如,像长度这样的基本单位, $L$,体积, $L^3$
- 表示另一个 $(m-n)$ 参数,一次一个,根据“自然单位”使用尺寸同质性的要求(例如,方程式。(1.78), (1.80), (1.81), (1.82), (1.85), (1.86)) $(m-n)$ 无量纲 $\Pi$-组。
- 检查以确保 $\Pi$

物理代写|声学代写Acoustics代考 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。
微观经济学代写
微观经济学是主流经济学的一个分支,研究个人和企业在做出有关稀缺资源分配的决策时的行为以及这些个人和企业之间的相互作用。my-assignmentexpert™ 为您的留学生涯保驾护航 在数学Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在图论代写Graph Theory代写方面经验极为丰富,各种图论代写Graph Theory相关的作业也就用不着 说。
线性代数代写
线性代数是数学的一个分支,涉及线性方程,如:线性图,如:以及它们在向量空间和通过矩阵的表示。线性代数是几乎所有数学领域的核心。
博弈论代写
现代博弈论始于约翰-冯-诺伊曼(John von Neumann)提出的两人零和博弈中的混合策略均衡的观点及其证明。冯-诺依曼的原始证明使用了关于连续映射到紧凑凸集的布劳威尔定点定理,这成为博弈论和数学经济学的标准方法。在他的论文之后,1944年,他与奥斯卡-莫根斯特恩(Oskar Morgenstern)共同撰写了《游戏和经济行为理论》一书,该书考虑了几个参与者的合作游戏。这本书的第二版提供了预期效用的公理理论,使数理统计学家和经济学家能够处理不确定性下的决策。
微积分代写
微积分,最初被称为无穷小微积分或 “无穷小的微积分”,是对连续变化的数学研究,就像几何学是对形状的研究,而代数是对算术运算的概括研究一样。
它有两个主要分支,微分和积分;微分涉及瞬时变化率和曲线的斜率,而积分涉及数量的累积,以及曲线下或曲线之间的面积。这两个分支通过微积分的基本定理相互联系,它们利用了无限序列和无限级数收敛到一个明确定义的极限的基本概念 。
计量经济学代写
什么是计量经济学?
计量经济学是统计学和数学模型的定量应用,使用数据来发展理论或测试经济学中的现有假设,并根据历史数据预测未来趋势。它对现实世界的数据进行统计试验,然后将结果与被测试的理论进行比较和对比。
根据你是对测试现有理论感兴趣,还是对利用现有数据在这些观察的基础上提出新的假设感兴趣,计量经济学可以细分为两大类:理论和应用。那些经常从事这种实践的人通常被称为计量经济学家。
Matlab代写
MATLAB 是一种用于技术计算的高性能语言。它将计算、可视化和编程集成在一个易于使用的环境中,其中问题和解决方案以熟悉的数学符号表示。典型用途包括:数学和计算算法开发建模、仿真和原型制作数据分析、探索和可视化科学和工程图形应用程序开发,包括图形用户界面构建MATLAB 是一个交互式系统,其基本数据元素是一个不需要维度的数组。这使您可以解决许多技术计算问题,尤其是那些具有矩阵和向量公式的问题,而只需用 C 或 Fortran 等标量非交互式语言编写程序所需的时间的一小部分。MATLAB 名称代表矩阵实验室。MATLAB 最初的编写目的是提供对由 LINPACK 和 EISPACK 项目开发的矩阵软件的轻松访问,这两个项目共同代表了矩阵计算软件的最新技术。MATLAB 经过多年的发展,得到了许多用户的投入。在大学环境中,它是数学、工程和科学入门和高级课程的标准教学工具。在工业领域,MATLAB 是高效研究、开发和分析的首选工具。MATLAB 具有一系列称为工具箱的特定于应用程序的解决方案。对于大多数 MATLAB 用户来说非常重要,工具箱允许您学习和应用专业技术。工具箱是 MATLAB 函数(M 文件)的综合集合,可扩展 MATLAB 环境以解决特定类别的问题。可用工具箱的领域包括信号处理、控制系统、神经网络、模糊逻辑、小波、仿真等。

