如果你也在 怎样代写统计物理Statistical Physics of Matter PHYC30017这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。统计物理Statistical Physics of Matter是在统计力学的基础上发展起来的一个物理学分支,它在解决物理问题时使用了概率论和统计学的方法,特别是处理大群体和近似的数学工具。
统计物理Statistical Physics of Matter解释并定量描述了超导性、超流性、湍流、固体和等离子体的集体现象以及液体的结构特征。它是现代天体物理学的基础。在固态物理学中,统计物理学有助于液晶、相变和临界现象的研究。许多物质的实验研究完全基于系统的统计描述。其中包括冷中子、X射线、可见光等的散射。统计物理学在材料科学、核物理学、天体物理学、化学、生物学和医学(如研究传染病的传播)中也发挥了作用。
统计物理Statistical Physics of Matter代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。 最高质量的物理Statistical Physics of Matter作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此统计物理Statistical Physics of Matter作业代写的价格不固定。通常在专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
同学们在留学期间,都对各式各样的作业考试很是头疼,如果你无从下手,不如考虑my-assignmentexpert™!
my-assignmentexpert™提供最专业的一站式服务:Essay代写,Dissertation代写,Assignment代写,Paper代写,Proposal代写,Proposal代写,Literature Review代写,Online Course,Exam代考等等。my-assignmentexpert™专注为留学生提供Essay代写服务,拥有各个专业的博硕教师团队帮您代写,免费修改及辅导,保证成果完成的效率和质量。同时有多家检测平台帐号,包括Turnitin高级账户,检测论文不会留痕,写好后检测修改,放心可靠,经得起任何考验!
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
我们在数学Mathematics代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在数学分析Mathematical Analysis代写方面经验极为丰富,各种数学分析Mathematical Analysis相关的作业也就用不着 说。
物理代写|统计物理代写Statistical Physics of Matter代考|Ising Model
Because of its historical relevance for the study of phase transitions in statistical physics and its broad applicability in many other fields, we now apply the outlined terminology from Section $3.1$ to the Ising model. Wilhelm Lenz proposed this model to his doctoral student Ernst Ising (see Figure 3.5) to describe systems that are composed of magnetic dipole moments. Each dipole moment can be in one of two states $(+1$ or $-1)$. The original goal was to model phase transitions in magnetic materials. As part of his doctoral thesis in 1924, Ernst Ising showed that the one-dimensional model exhibits no phase transition. For that reason, it was expected that this model was of no particular use. Then in 1944, Lars Onsager (see Figure 15.4) derived the partition function of the two-dimensional Ising model without magnetic field, finding a critical point at a finite temperature $T_c[111]$ and later in 1949 published an equation to describe the temperature dependence of the magnetization. Onsager provided no proof for his formula, but it is known that he derived it using Toeplitz determinants [112]. A few years passed until a proof was established by Yang in 1952 [113]. Since then, the Ising model has been successfully applied to a large number of physical and nonphysical problems such as magnetic systems, binary mixtures, and models of opinion formation. Also when compared with experimental data, the Ising model has been found to generally agree very well with observations made for certain magnetic materials [114]. To date, no general analytical solution for the Ising model in three dimensions is known. This is the reason why this mathematical model has been studied so intensively from a numerical perspective [115-119], using tools from statistical physics-some of which we describe in this section.
物理代写|统计物理代写Statistical Physics of Matter代考|Order Parameter
The magnetization is
$$
M(T, H)=\left\langle\frac{1}{N} \sum_{i=1}^N \sigma_i\right\rangle,
$$
and corresponds to the ensemble average of the mean value of all spins. If the external field vanishes, the Hamiltonian is invariant under a simultaneous reversal of all spins. In other words, a certain equilibrated configuration of spins would also have the same energy if we would change the sign of every single spin.
Thus, the ensemble average defined by eq. (3.21) would not be a good measure of the magnetization because it corresponds to an ensemble average over all possible configurations. For every temperature, $M(T)$ vanishes because for every configuration there exists a configuration of opposite sign which neutralizes it. Therefore, we use the so-called spontaneous magnetization
$$
M_{\mathrm{S}}(T)=\lim {H \rightarrow 0^{+}}\left\langle\frac{1}{N} \sum{i=1}^N \sigma_i\right\rangle
$$
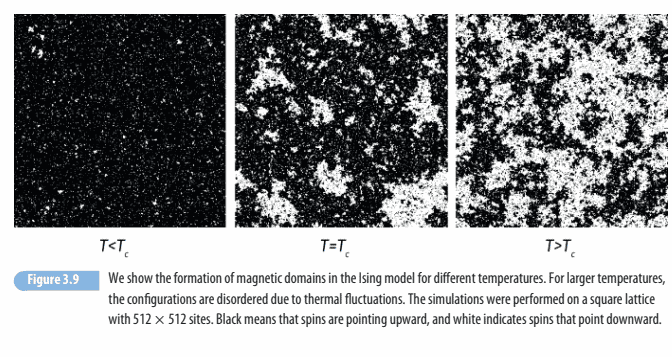
as the order parameter of the Ising model. In the definition of $M_{\mathrm{S}}(T)$, the symmetry of the Ising model is broken by applying a vanishing positive field $H$ that aligns the spins in one direction. Another possibility of breaking the symmetry can be realized by keeping the boundaries of the lattice in a certain state. This is, however, impracticable if periodic boundaries are being used. In Figure 3.9, we illustrate typical domain configurations for different temperatures. If $T>T_c$, one observes that thermal fluctuations lead to disordered configurations. On the other hand, magnetic domains form for small enough temperatures. Similar to eq. (2.1) in the case of percolation, in the vicinity of the critical temperature for $T<T_c$, the spontaneous magnetization scales as $[109]$
$$
M_{\mathrm{S}}(T) \propto\left(T_c-T\right)^\beta,
$$
where $\beta=1 / 8$ in two and $\beta=0.326419(3)$ in three dimensions [118]. For $T=T_c$ and $H \rightarrow 0$, we find [109]
$$
M\left(T=T_c, H\right) \propto H^{1 / \delta}
$$
where $\delta=15$ in two and $\delta=4.78984$ (1) in three dimensions [118]. The exponents $\beta$ and $\delta$ are critical exponents and characterize, together with other exponents, the underlying phase transition. Different techniques such as series expansions, field-theoretic methods, and very sophisticated Monte Carlo algorithms exist to determine critical exponents and critical temperatures with high precision.

统计物理代写
物理代写|统计物理代写物质统计物理学代考|Ising Model
由于它与统计物理学中相变研究的历史相关性以及它在许多其他领域的广泛适用性,我们现在将$3.1$节中概述的术语应用于Ising模型。Wilhelm Lenz向他的博士生Ernst Ising(见图3.5)提出了这个模型来描述由磁偶极矩组成的系统。每个偶极矩可以处于$(+1$或$-1)$两种状态之一。最初的目标是模拟磁性材料的相变。Ernst Ising在1924年的博士论文中指出,一维模型不存在相变。因此,预期这种模式没有特别的用处。随后在1944年,Lars Onsager(见图15.4)推导了二维无磁场Ising模型的配分函数,在有限温度处找到了一个临界点$T_c[111]$,随后在1949年发表了一个描述磁化温度依赖性的方程。Onsager没有为他的公式提供证明,但众所周知,他用Toeplitz行列式推导出了这个公式[112]。几年过去了,直到1952年Yang建立了一个证明[113]。从那时起,Ising模型成功地应用于大量的物理和非物理问题,如磁性系统、二元混合物和意见形成模型。同样,当与实验数据进行比较时,Ising模型也被发现大体上与对某些磁性材料的观测非常一致[114]。到目前为止,还不知道Ising模型在三维上的一般解析解。这就是为什么这个数学模型已经从数值的角度进行了如此深入的研究[115-119],使用了统计物理学的工具——其中一些我们将在本节中介绍
物理代写|统计物理代写物质统计物理代考|顺序参数
.
磁化强度
$$
M(T, H)=\left\langle\frac{1}{N} \sum_{i=1}^N \sigma_i\right\rangle,
$$
,对应于所有自旋平均值的集合平均值。如果外部场消失,在所有自旋同时反转的情况下,哈密顿量是不变的。换句话说,某个自旋的平衡构型也会有相同的能量如果我们改变每个自旋的符号。因此,eq.(3.21)定义的集合平均值不是一个很好的磁化度量,因为它对应于所有可能构型的集合平均值。对于每一种温度,$M(T)$都消失了,因为对于每一种构型,都存在一种相反的构型来中和它。因此,我们使用所谓自发磁化
$$
M_{\mathrm{S}}(T)=\lim {H \rightarrow 0^{+}}\left\langle\frac{1}{N} \sum{i=1}^N \sigma_i\right\rangle
$$
作为Ising模型的序参数。在$M_{\mathrm{S}}(T)$的定义中,Ising模型的对称性通过应用一个消失的正场$H$而被打破,使自旋在一个方向上对齐。打破对称性的另一种可能性可以通过保持晶格的边界处于某种状态来实现。然而,如果使用周期边界,这是不可行的。在图3.9中,我们演示了不同温度下的典型域配置。如果$T>T_c$,人们观察到热波动导致无序的结构。另一方面,在足够小的温度下形成磁畴。与渗流情况下式(2.1)类似,在$T<T_c$的临界温度附近,自发磁化尺度为$[109]$
$$
M_{\mathrm{S}}(T) \propto\left(T_c-T\right)^\beta,
$$
,其中二维为$\beta=1 / 8$,三维为$\beta=0.326419(3)$[118]。对于$T=T_c$和$H \rightarrow 0$,我们发现[109]
$$
M\left(T=T_c, H\right) \propto H^{1 / \delta}
$$
其中$\delta=15$是二维的,$\delta=4.78984$(1)是三维的[118]。指数$\beta$和$\delta$是临界指数,和其他指数一起描述了基本的相变。不同的技术,如级数展开,场理论方法,和非常复杂的蒙特卡罗算法存在高精度地确定临界指数和临界温度

物理代写|统计物理代写Statistical Physics of Matter代考 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。
微观经济学代写
微观经济学是主流经济学的一个分支,研究个人和企业在做出有关稀缺资源分配的决策时的行为以及这些个人和企业之间的相互作用。my-assignmentexpert™ 为您的留学生涯保驾护航 在数学Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在图论代写Graph Theory代写方面经验极为丰富,各种图论代写Graph Theory相关的作业也就用不着 说。
线性代数代写
线性代数是数学的一个分支,涉及线性方程,如:线性图,如:以及它们在向量空间和通过矩阵的表示。线性代数是几乎所有数学领域的核心。
博弈论代写
现代博弈论始于约翰-冯-诺伊曼(John von Neumann)提出的两人零和博弈中的混合策略均衡的观点及其证明。冯-诺依曼的原始证明使用了关于连续映射到紧凑凸集的布劳威尔定点定理,这成为博弈论和数学经济学的标准方法。在他的论文之后,1944年,他与奥斯卡-莫根斯特恩(Oskar Morgenstern)共同撰写了《游戏和经济行为理论》一书,该书考虑了几个参与者的合作游戏。这本书的第二版提供了预期效用的公理理论,使数理统计学家和经济学家能够处理不确定性下的决策。
微积分代写
微积分,最初被称为无穷小微积分或 “无穷小的微积分”,是对连续变化的数学研究,就像几何学是对形状的研究,而代数是对算术运算的概括研究一样。
它有两个主要分支,微分和积分;微分涉及瞬时变化率和曲线的斜率,而积分涉及数量的累积,以及曲线下或曲线之间的面积。这两个分支通过微积分的基本定理相互联系,它们利用了无限序列和无限级数收敛到一个明确定义的极限的基本概念 。
计量经济学代写
什么是计量经济学?
计量经济学是统计学和数学模型的定量应用,使用数据来发展理论或测试经济学中的现有假设,并根据历史数据预测未来趋势。它对现实世界的数据进行统计试验,然后将结果与被测试的理论进行比较和对比。
根据你是对测试现有理论感兴趣,还是对利用现有数据在这些观察的基础上提出新的假设感兴趣,计量经济学可以细分为两大类:理论和应用。那些经常从事这种实践的人通常被称为计量经济学家。
Matlab代写
MATLAB 是一种用于技术计算的高性能语言。它将计算、可视化和编程集成在一个易于使用的环境中,其中问题和解决方案以熟悉的数学符号表示。典型用途包括:数学和计算算法开发建模、仿真和原型制作数据分析、探索和可视化科学和工程图形应用程序开发,包括图形用户界面构建MATLAB 是一个交互式系统,其基本数据元素是一个不需要维度的数组。这使您可以解决许多技术计算问题,尤其是那些具有矩阵和向量公式的问题,而只需用 C 或 Fortran 等标量非交互式语言编写程序所需的时间的一小部分。MATLAB 名称代表矩阵实验室。MATLAB 最初的编写目的是提供对由 LINPACK 和 EISPACK 项目开发的矩阵软件的轻松访问,这两个项目共同代表了矩阵计算软件的最新技术。MATLAB 经过多年的发展,得到了许多用户的投入。在大学环境中,它是数学、工程和科学入门和高级课程的标准教学工具。在工业领域,MATLAB 是高效研究、开发和分析的首选工具。MATLAB 具有一系列称为工具箱的特定于应用程序的解决方案。对于大多数 MATLAB 用户来说非常重要,工具箱允许您学习和应用专业技术。工具箱是 MATLAB 函数(M 文件)的综合集合,可扩展 MATLAB 环境以解决特定类别的问题。可用工具箱的领域包括信号处理、控制系统、神经网络、模糊逻辑、小波、仿真等。


