如果你也在 怎样代写分形几何和混沌系统Fractal Geometry & Chaotic Dynamics MATH3062这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。分形几何和混沌系统Fractal Geometry & Chaotic Dynamics在数学中,分形是一种在任意小的尺度上包含详细结构的几何形状,通常具有严格超过拓扑维数的分形维数。许多分形在不同尺度上看起来都很相似,如曼德布罗特集的连续放大图。这种在越来越小的尺度上展示相似的图案被称为自相似性,也被称为扩展对称性或展开对称性;如果这种复制在每个尺度上都完全相同,如门格尔海绵,这种形状被称为仿生自相似性。
分形几何和混沌系统Fractal Geometry & Chaotic Dynamics分形与有限几何图形的一个不同之处在于它们的尺度。将一个填充多边形的边长增加一倍,其面积就会乘以4,也就是2(新边长与旧边长之比)提高到2的幂(填充多边形的常规尺寸)。同样,如果一个填充球体的半径增加一倍,它的体积就会增加8,也就是2(新与旧半径之比)到3的幂(填充球体的常规尺寸)。然而,如果一个分形的一维长度全部翻倍,那么分形的空间内容就会以一个不一定是整数的幂来扩展,一般来说,这个幂大于其常规维度。这个幂被称为几何对象的分形维度,以区别于常规维度(正式名称为拓扑维度)。
分形几何和混沌系统Fractal Geometry & Chaotic Dynamics代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。最高质量的分形几何和混沌系统Fractal Geometry & Chaotic Dynamics作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此分形几何和混沌系统Fractal Geometry & Chaotic Dynamics作业代写的价格不固定。通常在专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
同学们在留学期间,都对各式各样的作业考试很是头疼,如果你无从下手,不如考虑my-assignmentexpert™!
my-assignmentexpert™提供最专业的一站式服务:Essay代写,Dissertation代写,Assignment代写,Paper代写,Proposal代写,Proposal代写,Literature Review代写,Online Course,Exam代考等等。my-assignmentexpert™专注为留学生提供Essay代写服务,拥有各个专业的博硕教师团队帮您代写,免费修改及辅导,保证成果完成的效率和质量。同时有多家检测平台帐号,包括Turnitin高级账户,检测论文不会留痕,写好后检测修改,放心可靠,经得起任何考验!

数学代写|分形几何和混沌系统代考Fractal Geometry & Chaotic Dynamics代写|Basic Concepts and Examples
a. A threefold cord: fractals, dynamics, and chaos. The word “fractal” is one which has wriggled its way into the popular consciousness over the past few decades, to the point where a Google search for “fractal” yields over 12 million results (at the time of this writing), more than six times as many as a search for the rather more fundamental mathematical notion of “isomorphism”. With a few clicks of a mouse and without any need to enter the jargon-ridden world of academic publications, one may find websites devoted to fractals for kids, a blog featuring the fractal of the day, photo galleries of fractals occurring in nature, online stores selling posters brightly emblazoned with computer-generated images of fractals, … the list goes on.
Faced with this jungle of information, we may rightly ask, echoing Paul Gauguin, “What are fractals? Where do they come from? Where do we go with them?”
The answers to the second and third questions, at least as far as we are concerned, will have to do with the other two strands of the threefold cord holding this book together-namely, dynamical systems and chaos. 1 As an initial, naïve formulation, we may say that in most cases where a dynamical system exhibits chaotic behaviour, this behaviour is associated with the presence of a fractal. For our purposes, fractals will come from particular dynamical systems, and will lead us to an understanding of certain aspects of chaos.
But all in good time. We must begin by addressing the first question, “What are fractals?”
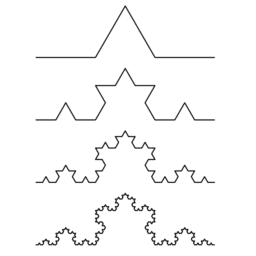
b. Fractals: intricate geometry and self-similarity. Consider an oak tree in the dead of winter, 2 viewed from a good distance away, as in the first panel of Figure 1.1. Its trunk rises from the ground to the point where it bifurcates into two large boughs; each of these boughs leads away from the centre of the tree and eventually sends off smaller branches of its own. Walking closer to the tree, one sees that these branches in turn send off still smaller branches, which were not visible from further away, and more careful inspection reveals a similar branching structure all the way down to the level of tiny twigs only an inch or two long. The various scales are shown in the second and third panels of Figure 1.1.
The key points to observe are as follows. First, the tree has a complicated and intricate shape, which is not well captured by more familiar geometric objects, such as lines, circles, polygons, and so on. Second, we see the same sort of shape on all scales: whether we view the tree from fifty yards away or from fifty inches, we will see a branching structure in which the largest branch (or trunk) in our field of view splits into smaller branches, which then divide themselves, and so on. This self-similarity is one of the key characteristics of fractals.
数学代写|分形几何和混沌系统代考Fractal Geometry & Chaotic Dynamics代写|Population models and the logistic map
b.1. A rather unrealistic population model. Consider a population of duck-billed platypi (or bacteria, or whatever species you fancy), whose size will be represented by a variable x. Given the size of the population at the present time, we want to predict the size of next year’s population (or perhaps the next hour’s, in the case of bacteria). So if there are x platypi this year, there will be f(x) next year, where f is a suitable function which models the change in the platypus population from year to year. Of course, since we cannot have a negative number of platypi, we must restrict x to lie in the interval [0,∞), which will be the domain of definition for f.
What form should f take? As a first (simplistic) approximation, we may suppose that the platypi reproduce at a constant rate, and so if there are x of them this year, there will be rx next year, where r>1 is a real number, and r−1 represents the proportion of newborns each year.

分形几何和混沌系统
数学代写|分形几何和混沌系统代考FRACTAL GEOMETRY \& CHAOTIC DYNAMICS代写|BASIC CONCEPTS AND EXAMPLES
一个。三重绳索: 分形、动态和混沌。在过唟的几十年里, “分形这个词已经进入大众意识,以至于谷歌搜索“分形”会产生超过 1200 万个结果
atthetimeofthiswriting,是寻找更基本的数学概念“同构”的六倍多。只需点击几下鼠标,无需进入充满行话的学术出版物世界,您就可以找到专门为孩子们设 计的分形网站、一个以当今分形为特色的博宮、自然界中发生的分形照片库、在线商店出售的海报上印有计算机生成的分形图像,….不胜枚举。
面对这个信息从林,我们可以正确地问,呼应保罗.高更,“什么是分形? 他们来自哪里? 我们和他们一起去哪里? ”
至少就伐们而言,第二个和第三个问题的答案与将本书联系在一起的三股绳䒺的另外两条线有关一-即动力系统和混沌。 1 作为一个初始的、朴龶的表述,我们可以
说,在动力系统表现出混沌行为的大多数情况下,这种行为与分形的存在有关。就伐们的目的而言,分形将来自特定的动力系统,并将引导我们理解混沌的某些方
但一切都来得及。我们必须从解决第一个问题开始, “什么是分形? ”
湾。分形: 复杂的几何形状和自相似性。想一想严冬中的一棵橡㳔, 2 从很远的地方观看,如图 1.1 的第一幅图所示。它的树干从地面上升到分叉成两个大树枝的地
方。这些树枝中的每一个都从树的中心引出,并最终发出自己的小树枝。赽近树,你会看到这些树枝依次发出更小的树枝,这些树枝从更远的地方看不到,更仔细
此类推。这种自相似性是分形的关键特征之一。
数学代写|分形几何和混沌系统代考FRACTAL GEOMETRY \&CHAOTIC DYNAMICS代写|POPULATION MODELS AND THE LOGISTIC MAP
b.1。一个相当不切实际的人口模型。考虑一群鸭哺鸭orbacteria, orwhateverspeciesyou fancy,其大小将由一个变量表示 x. 㧛于目前的人口规模,我们想预测
应该用什么形式 f 拿? 作为第一simplistic近似,我们可以假设鸭哺鲁以恒定的速率慗殖,所以如果有 x 今年将有 rx 明年在哪里 r>1 是一个实数,并且 r−1 代表
每年新生儿的比例。

数学代写|分形几何和混沌系统代考Fractal Geometry & Chaotic Dynamics代写 请认准exambang™. exambang™为您的留学生涯保驾护航。
微观经济学代写
微观经济学是主流经济学的一个分支,研究个人和企业在做出有关稀缺资源分配的决策时的行为以及这些个人和企业之间的相互作用。my-assignmentexpert™ 为您的留学生涯保驾护航 在数学Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在图论代写Graph Theory代写方面经验极为丰富,各种图论代写Graph Theory相关的作业也就用不着 说。
线性代数代写
线性代数是数学的一个分支,涉及线性方程,如:线性图,如:以及它们在向量空间和通过矩阵的表示。线性代数是几乎所有数学领域的核心。
博弈论代写
现代博弈论始于约翰-冯-诺伊曼(John von Neumann)提出的两人零和博弈中的混合策略均衡的观点及其证明。冯-诺依曼的原始证明使用了关于连续映射到紧凑凸集的布劳威尔定点定理,这成为博弈论和数学经济学的标准方法。在他的论文之后,1944年,他与奥斯卡-莫根斯特恩(Oskar Morgenstern)共同撰写了《游戏和经济行为理论》一书,该书考虑了几个参与者的合作游戏。这本书的第二版提供了预期效用的公理理论,使数理统计学家和经济学家能够处理不确定性下的决策。
微积分代写
微积分,最初被称为无穷小微积分或 “无穷小的微积分”,是对连续变化的数学研究,就像几何学是对形状的研究,而代数是对算术运算的概括研究一样。
它有两个主要分支,微分和积分;微分涉及瞬时变化率和曲线的斜率,而积分涉及数量的累积,以及曲线下或曲线之间的面积。这两个分支通过微积分的基本定理相互联系,它们利用了无限序列和无限级数收敛到一个明确定义的极限的基本概念 。
计量经济学代写
什么是计量经济学?
计量经济学是统计学和数学模型的定量应用,使用数据来发展理论或测试经济学中的现有假设,并根据历史数据预测未来趋势。它对现实世界的数据进行统计试验,然后将结果与被测试的理论进行比较和对比。
根据你是对测试现有理论感兴趣,还是对利用现有数据在这些观察的基础上提出新的假设感兴趣,计量经济学可以细分为两大类:理论和应用。那些经常从事这种实践的人通常被称为计量经济学家。
Matlab代写
MATLAB 是一种用于技术计算的高性能语言。它将计算、可视化和编程集成在一个易于使用的环境中,其中问题和解决方案以熟悉的数学符号表示。典型用途包括:数学和计算算法开发建模、仿真和原型制作数据分析、探索和可视化科学和工程图形应用程序开发,包括图形用户界面构建MATLAB 是一个交互式系统,其基本数据元素是一个不需要维度的数组。这使您可以解决许多技术计算问题,尤其是那些具有矩阵和向量公式的问题,而只需用 C 或 Fortran 等标量非交互式语言编写程序所需的时间的一小部分。MATLAB 名称代表矩阵实验室。MATLAB 最初的编写目的是提供对由 LINPACK 和 EISPACK 项目开发的矩阵软件的轻松访问,这两个项目共同代表了矩阵计算软件的最新技术。MATLAB 经过多年的发展,得到了许多用户的投入。在大学环境中,它是数学、工程和科学入门和高级课程的标准教学工具。在工业领域,MATLAB 是高效研究、开发和分析的首选工具。MATLAB 具有一系列称为工具箱的特定于应用程序的解决方案。对于大多数 MATLAB 用户来说非常重要,工具箱允许您学习和应用专业技术。工具箱是 MATLAB 函数(M 文件)的综合集合,可扩展 MATLAB 环境以解决特定类别的问题。可用工具箱的领域包括信号处理、控制系统、神经网络、模糊逻辑、小波、仿真等。