如果你也在 怎样代写有限元方法finite differences method ME335A这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。有限元方法finite differences method在数值分析中,是一类通过用有限差分逼近导数解决微分方程的数值技术。空间域和时间间隔(如果适用)都被离散化,或被分成有限的步骤,通过解决包含有限差分和附近点的数值的代数方程来逼近这些离散点的解的数值。
有限元方法finite differences method有限差分法将可能是非线性的常微分方程(ODE)或偏微分方程(PDE)转换成可以用矩阵代数技术解决的线性方程系统。现代计算机可以有效地进行这些线性代数计算,再加上其相对容易实现,使得FDM在现代数值分析中得到了广泛的应用。今天,FDM与有限元方法一样,是数值解决PDE的最常用方法之一。
有限元方法finite differences method作业代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。 最高质量的有限元方法finite differences method作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于统计Statistics作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此有限元方法finite differences method作业代写的价格不固定。通常在经济学专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
同学们在留学期间,都对各式各样的作业考试很是头疼,如果你无从下手,不如考虑my-assignmentexpert™!
my-assignmentexpert™提供最专业的一站式服务:Essay代写,Dissertation代写,Assignment代写,Paper代写,Proposal代写,Proposal代写,Literature Review代写,Online Course,Exam代考等等。my-assignmentexpert™专注为留学生提供Essay代写服务,拥有各个专业的博硕教师团队帮您代写,免费修改及辅导,保证成果完成的效率和质量。同时有多家检测平台帐号,包括Turnitin高级账户,检测论文不会留痕,写好后检测修改,放心可靠,经得起任何考验!
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
我们在数学Mathematics代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在微积分Calculus Assignment代写方面经验极为丰富,各种微积分Calculus Assignment相关的作业也就用不着 说。
数学代写|有限元方法作业代写finite differences method代考|Moments and Shear Forces
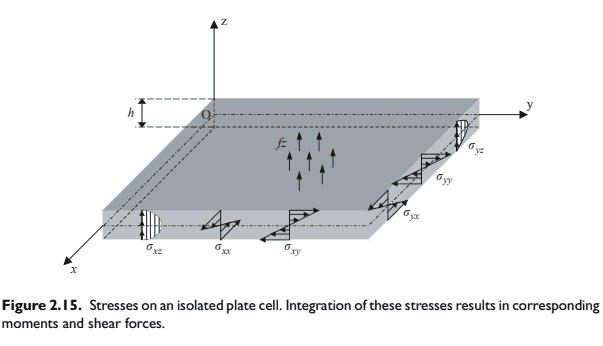
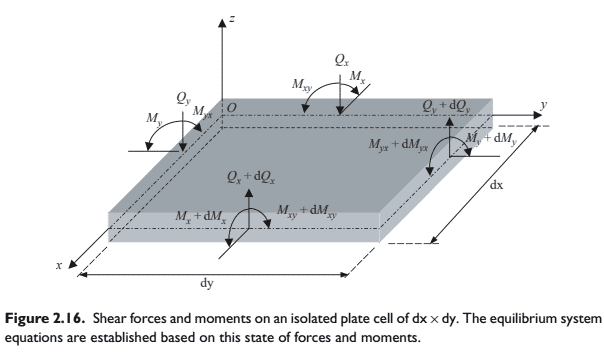
Figure $2.15$ shows a small representative cell of $\mathrm{d} x \times \mathrm{d} y$ from a plate of thickness $h$. The plate cell is subjected to external force $f_z$, and inertial force $\rho h \ddot{w}$, where $\rho$ is the density of the material. Figure $2.16$ shows the moments $M_x, M_y, M_z$ and $M_{x y}$, and shear forces $Q_x$ and $Q_y$ present. The moments and shear forces result from the distributed normal and shear stresses $\sigma_{x x}, \sigma_{y y}$ and $\sigma_{x y}$, shown in Figure 2.15. The stresses can be obtained by substituting Eq. (2.66) into Eq. (2.68)
$$
\sigma=-z \mathbf{c L} w
$$
Secondly, the displacements parallel to the undeformed middle plane, $u$ and $v$, at a distance $z$ from the centroidal axis can be expressed by
$$
\begin{aligned}
&u=-z \frac{\partial w}{\partial x} \
&v=-z \frac{\partial w}{\partial y}
\end{aligned}
$$
where $w$ is the deflection of the middle plane of the plate in the $z$ direction. The relationship between the components of strain and the deflection can be given by
$$
\begin{aligned}
\varepsilon_{x x} &=\frac{\partial u}{\partial x}=-z \frac{\partial^2 w}{\partial x^2} \
\varepsilon_{y y} &=\frac{\partial v}{\partial y}=-z \frac{\partial^2 w}{\partial y^2} \
\varepsilon_{x y} &=\frac{\partial u}{\partial y}+\frac{\partial v}{\partial x}=-2 z \frac{\partial^2 w}{\partial x \partial y}
\end{aligned}
$$
or in the matrix form
$$
\boldsymbol{\varepsilon}=-z \mathbf{L} w
$$
where $\boldsymbol{\varepsilon}$ is the vector of in-plane strains defined by Eq. (2.25), and $\mathbf{L}$ is the differential operator matrix given by
$$
\mathbf{L}=\left[\begin{array}{c}
\partial^2 / \partial x^2 \
\partial^2 / \partial y^2 \
2 \partial^2 / \partial x \partial y
\end{array}\right]
$$
数学代写|有限元方法作业代写finite differences method代考|Constitutive Equations
The original Hooke’s law is applicable for plates:
$$
\sigma=c \varepsilon
$$
where $\mathbf{c}$ has the same form for $2 \mathrm{D}$ solids defined by Eq. (2.31), the plane stress case, since $\sigma_{z z}$ is assumed to be zero.
Figure $2.15$ shows a small representative cell of $\mathrm{d} x \times \mathrm{d} y$ from a plate of thickness $h$. The plate cell is subjected to external force $f_z$, and inertial force $\rho h \ddot{w}$, where $\rho$ is the density of the material. Figure $2.16$ shows the moments $M_x, M_y, M_z$ and $M_{x y}$, and shear forces $Q_x$ and $Q_y$ present. The moments and shear forces result from the distributed normal and shear stresses $\sigma_{x x}, \sigma_{y y}$ and $\sigma_{x y}$, shown in Figure 2.15. The stresses can be obtained by substituting Eq. (2.66) into Eq. (2.68)
$$
\sigma=-z \mathbf{c L} w
$$
有限元方法代写
数学代写有限元方法作业代写FINITE DIFFERENCES METHOD 代考|MOMENTS AND SHEAR FORCES
数字 $2.15$ 显示了一个小的代表性单元格 $\mathrm{d} x \times \mathrm{d} y$ 从一块厚板 $h$. 板状电池受到外力 $f_z$, 和惯性力 $\rho h \ddot{w}$ ,在哪里 $\rho$ 是材料的密度。数字 $2.16$ 显示时刻 $M_x, M_y, M_z$ 和 $M_{x y}$, 和剪切力 $Q_x$ 和 $Q_y$ 当下。力矩和前力由分布的法向应力和剪应力产生 $\sigma_{x x}, \sigma_{y y}$ 和 $\sigma_{x y}$ ,如图 2.15所示。应力可以通过代入方程式获得。 $2.66$ 进入方程。 $2.68$ $\sigma=-z \mathbf{c L} w$
其次,平行于末变形中间平面的位移, $u$ 和 $v$, 在远处 $z$ 从质心轴可以表示为
$$
u=-z \frac{\partial w}{\partial x} \quad v=-z \frac{\partial w}{\partial y}
$$
在哪里 $w$ 是板的中间平面的挠度在 $z$ 方向。应变分量与挠度之间的关系可由下式给出
$$
\varepsilon_{x x}=\frac{\partial u}{\partial x}=-z \frac{\partial^2 w}{\partial x^2} \varepsilon_{y y} \quad=\frac{\partial v}{\partial y}=-z \frac{\partial^2 w}{\partial y^2} \varepsilon_{x y}=\frac{\partial u}{\partial y}+\frac{\partial v}{\partial x}=-2 z \frac{\partial^2 w}{\partial x \partial y}
$$
或以矩阵形式
$$
\varepsilon=-z \mathbf{L} w
$$
在哪里 $\varepsilon$ 是由等式定义的面内应变向量。 $2.25$ ,和 $\mathbf{L}$ 是由下式给出的微分算子矩阵
$$
\mathbf{L}=\left[\partial^2 / \partial x^2 \partial^2 / \partial y^2 2 \partial^2 / \partial x \partial y\right]
$$
数学代写有限元方法作业代写FINITE DIFFERENCES METHOD 代考|CONSTITUTIVE EQUATIONS
原始胡克定律适用于板材:
$$
\sigma=c \varepsilon
$$
在哪里 $\mathbf{c}$ 具有相同的形式 $2 \mathrm{D}$ 由方程式定义的固体。 $2.31$ ,平面应力情况,因为 $\sigma_{z z}$ 假定为零。
数字 $2.15$ 显示了一个小的代表性单元格 $\mathrm{d} x \times \mathrm{d} y$ 从一块厚板 $h$. 板状电池受到外力 $f_z$, 和惯性力 $\rho h \ddot{w} ,$ 在哪里 $\rho$ 是材料的密度。数字 $2.16$ 显示时刻 $M_x, M_y, M_z$ 和 $M_{x y}$, 和前切力 $Q_x$ 和 $Q_y$ 当下。力矩和前力由分布的法向应力和剪应力产生 $\sigma_{x x}, \sigma_{y y}$ 和 $\sigma_{x y}$ ,如图 2.15 所示。应力可以通过代入方程式获得。 $2.66$ 进入方程。 $2.68$
$$
\sigma=-z \mathbf{c L} w
$$

数学代写|有限元方法作业代写finite differences method代考 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。

