数学代写|Math432 Topology

MY-ASSIGNMENTEXPERT™可以为您提供 coursicle.com Math432 Topology拓扑学的代写代考和辅导服务！

Math432课程简介

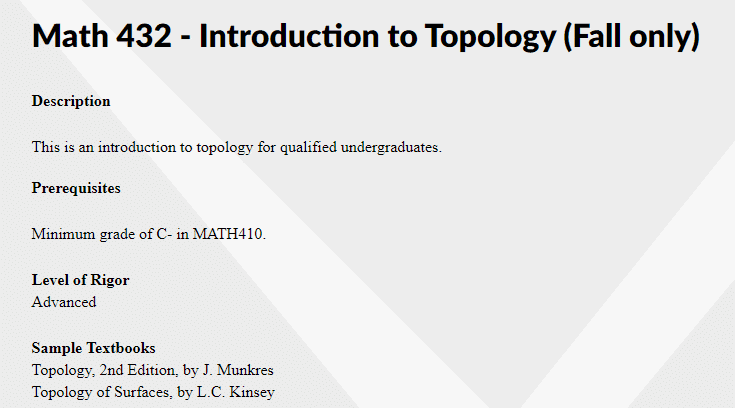
Description
Prerequisite: Minimum grade of C- in MATH410. Metric spaces, topological spaces, connectedness, compactness including Heine-Borel and Bolzano-Weierstrass theorems, Cantor sets, continuous maps and homeomorphisms, fundamental group homotopy, covering spaces, the fundamental theorem of algebra, Brouwer fixed point theorem, surfaces e.g., Euler characteristic, the index of a vector field, hairy sphere theorem, elements of combinatorial topology (graphs and trees, planarity, coloring problems.Credits3

Prerequisites 

Open Seat Checker
Get notified when MATH 432 has an open seat
Schedule Planner
Add MATH 432 to your schedule
Recent Semesters
Fall 2023, Fall 2022, Fall 2021, Fall 2020, Fall 2019

Usually Held
TuTh (9:30am-10:45am), TuTh (11:00am-12:15pm), TuTh (2:00pm-3:15pm)

Class Size
20-28

Math432 Topology HELP（EXAM HELP， ONLINE TUTOR）

Without using the Axiom of Choice, it is not possible to construct a choice function for the collection $\mathcal{A}$ of nonempty subsets of $\mathbb{Z}_{+}$.

To see this, consider the collection $\mathcal{A}’$ of nonempty subsets of $\mathbb{Z}_{+}$ that do not contain the number $1$. Then for any $A\in\mathcal{A}’$, we can define a choice function $f$ by setting $f(A)=\min A$. However, $\mathcal{A}’$ is nonempty and has no choice function, because if we take the union of any two sets in $\mathcal{A}’$, the resulting set will also be in $\mathcal{A}’$ and will not have a minimum element.

This shows that the collection $\mathcal{A}$ of nonempty subsets of $\mathbb{Z}_{+}$ cannot have a choice function without the Axiom of Choice.

It is possible to construct a choice function for the collection $\mathcal{B}$ of nonempty subsets of $\mathbb{Q}$ without using the Axiom of Choice.

Let $A\in\mathcal{B}$ be a nonempty subset of $\mathbb{Q}$. We can define a choice function $f$ for $\mathcal{B}$ as follows:

1. Choose an element $q_0\in A$.
2. For each positive integer $n$, let $q_n$ be the element of $A$ with the smallest denominator among those with denominator at most $n$ (if there are multiple such elements, choose the one with the smallest numerator).
3. Define $f(A)=\lim_{n\to\infty}q_n$.

Since $A$ is nonempty, we can always choose an element $q_0\in A$. The sequence $(q_n)$ is well-defined and nonempty because $A$ is nonempty, and it is Cauchy because the denominators are bounded. Therefore, the limit $\lim_{n\to\infty}q_n$ exists in $\mathbb{Q}$.

It remains to show that $f(A)$ is an element of $A$. Suppose not; then there exists an element $a\in A$ such that $a<f(A)$. Since $f(A)$ is the limit of $(q_n)$, there exists a positive integer $N$ such that $q_n>f(A)-\frac{a-f(A)}{2}$ for all $n\geq N$. But this implies that $a>f(A)-\frac{a-f(A)}{2}$, or equivalently, $a>\frac{f(A)}{2}+\frac{a}{2}$, which is a contradiction. Therefore, $f(A)\in A$, and $f$ is a choice function for $\mathcal{B}$.

Note that the above construction relies on the fact that $\mathbb{Q}$ is an ordered field, which allows us to use the idea of a “smallest denominator.” This technique would not work for collections of subsets of more general sets.

MY-ASSIGNMENTEXPERT™可以为您提供UNIVERSITY OF ILLINOIS URBANA-CHAMPAIGN MATH2940 linear algebra线性代数课程的代写代考和辅导服务！ 请认准MY-ASSIGNMENTEXPERT™. MY-ASSIGNMENTEXPERT™为您的留学生涯保驾护航。