数学代写|Math2050 Mathematical Analysis

MY-ASSIGNMENTEXPERT™可以为您提供catalog Math2050 Mathematical Analysis数学分析的代写代考和辅导服务！

Math2050课程简介

Add to My Favorites (opens a new window)
Share this Page
Print (opens a new window)
Help (opens a new window)
MATH 2050 – Probability and Statistics
3 credit hoursPrerequisite: MATH 1810 or MATH 1910. Data analysis, probability, and statistical inference. The inference material covers means, proportions, and variances for one and two samples, one-way ANOVA, regression and correlation, and chi-square analysis. TBR Common Course: MATH 2050

Prerequisites 

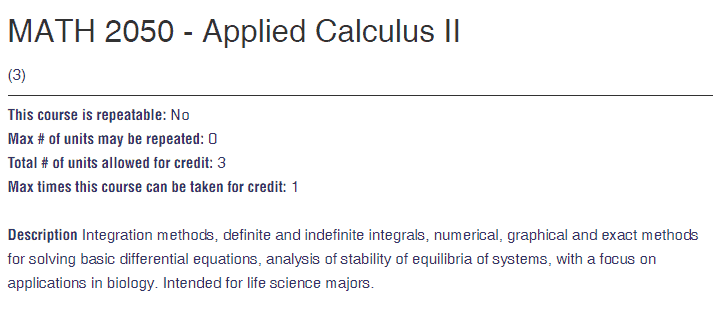
This course is repeatable: No
Max # of units may be repeated: 0
Total # of units allowed for credit: 3
Max times this course can be taken for credit: 1

Description Integration methods, definite and indefinite integrals, numerical, graphical and exact methods for solving basic differential equations, analysis of stability of equilibria of systems, with a focus on applications in biology. Intended for life science majors.

Grading: ABCDF
Mode of Delivery: Face to Face
Campus: Main Campus

Math2050 Mathematical Analysis HELP（EXAM HELP， ONLINE TUTOR）

First, note that $S$ is bounded above since $x<\frac{1}{x}$ implies $x^2<1$, which implies $|x|<1$ and thus $x<1$ (since $x$ is negative or positive). Hence, any upper bound $M$ of $S$ must satisfy $x<\frac{1}{x}<M$ for all $x\in S$. In particular, $M$ must be positive.

Let us show that $1$ is the supremum of $S$. That is, we need to show two things:

1. $1$ is an upper bound of $S$: If $x\in S$, then $x<\frac{1}{x}\leq 1$, so $x<1$. Hence $1$ is an upper bound of $S$.
2. If $M<1$ is any upper bound of $S$, then $M$ is not a supremum: Indeed, since $M<1$, we have $M<\frac{1}{M}$, so there exists $x\in S$ such that $x>M$. Therefore, $M$ is not a supremum of $S$, and we conclude that $\sup(S)=1$.

Next, we claim that $0$ is the infimum of $S$. To show this, we need to show two things:

1. $0$ is a lower bound of $S$: If $x\in S$, then $x<\frac{1}{x}$ implies $x^2<1$, so $x<0$ (since $x$ is negative). Hence, $0$ is a lower bound of $S$.
2. If $L>0$ is any lower bound of $S$, then $L$ is not an infimum: Indeed, since $L>0$, we have $\frac{1}{L}>0$, so there exists $x\in S$ such that $x<\frac{1}{L}<L$. Therefore, $L$ is not an infimum of $S$, and we conclude that $\inf(S)=0$.

Let $M=\sup S$ and $m=\inf{-s:s\in S}$. We want to show that $M=-m$.

Since $S$ is bounded from above, $M$ exists and by definition $s\leq M$ for all $s\in S$. Therefore, $-s\geq -M$ for all $s\in S$, which implies that $-M$ is a lower bound for the set ${-s:s\in S}$. Since this set is non-empty and bounded from below (since $S$ is bounded from above), we have that $m=\inf{-s:s\in S}$ exists.

We will now show that $M=-m$. First, we show that $M\geq -m$. Indeed, for any $s\in S$, we have $-s\leq m$, which implies that $s\geq -m$. Since $M$ is an upper bound for $S$, we have $s\leq M$ for all $s\in S$. Combining these two inequalities, we get $-m\leq s\leq M$ for all $s\in S$, which implies $-m\leq M$.

Next, we show that $M\leq -m$. To do this, we will show that $-M\geq m$. Since $m=\inf{-s:s\in S}$, we have $-s\geq m$ for all $s\in S$, which implies that $s\leq -m$ for all $s\in S$. Therefore, $-M$ is an upper bound for the set $S$. Since $M$ is the least upper bound for $S$, we have $M\leq -M$, which implies $M\leq -m$.

Combining the inequalities $-m\leq M$ and $M\leq -m$, we conclude that $M=-m$. This completes the proof.

MY-ASSIGNMENTEXPERT™可以为您提供UNIVERSITY OF ILLINOIS URBANA-CHAMPAIGN MATH2940 linear algebra线性代数课程的代写代考和辅导服务！ 请认准MY-ASSIGNMENTEXPERT™. MY-ASSIGNMENTEXPERT™为您的留学生涯保驾护航。