MY-ASSIGNMENTEXPERT™可以为您提供ph.tum.de MATH4604 Finite Element MethoD有限元方法的代写代考和辅导服务!
MATH4604课程简介
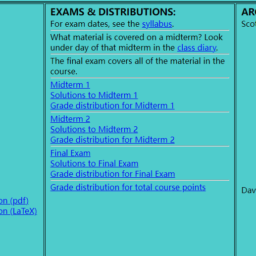
I either do “curve” or do not “curve” depending on how you define it. I do not preassign the number of students who will receive a specific grade. On the other hand, neither do I preassign the gradelines before seeing the distribution of grades. Gradelines will be announced on the web, as soon as possible after an exam.
Incompletes will be given only in cases where the student has completed all but a small fraction of the course with a grade of $\mathrm{C}$ or better and a severe unexpected event prevents completion of the course. In particular, if you get behind, you cannot “bail out” by taking an incomplete.
No extra credit work will be accepted.
All exams are closed book, with no calculators allowed. You may discuss homework with your classmates, even working on solutions, but the final writeup should be done individually.
Prerequisites
Office Hours:
Mondays: $12: 15 \mathrm{pm}-1: 15 \mathrm{pm}$, Vincent 354
Tuesdays: $1: 30 \mathrm{pm}-3: 30 \mathrm{pm}$, Vincent 354
Wednesdays: 10:00am-12:00noon, ZOOM
Thursdays: 2:45pm- $3: 45 \mathrm{pm}$, Vincent 354
NOTE: I do not hold office hours on University Holidays. I do not hold office hours before the first day of classes. I do not hold office hours during spring break. I do not hold office hours after the final exam.
NOTE: When no students are present, I may leave 10 minutes before the end of office hours. Also, on many days, even when students are present, I have to conclude my office hours on time, because of other obligations.
MATH4604 Finite Element Method HELP(EXAM HELP, ONLINE TUTOR)
12-4. Show: Let $V, W \in \mathrm{TS}, q, u, v \in V, f: V \rightarrow W, \phi:=f \circ \alpha_q^{u v}$.
Then $\left(\nabla_u \nabla_v f\right)q=\left(\partial_1 \partial_2 \phi\right){(0,0)}$.
We have:
\begin{align*} \left(\nabla_u \nabla_v f\right)q &= \nabla_u \left(\nabla_v f\right)q \ &= \nabla_u \left(\left(\nabla f\right)q(v)\right) \ &= \nabla_u \left(\lim{t \rightarrow 0} \frac{f(q+tv)-f(q)}{t}\right) \ &= \lim{t \rightarrow 0} \frac{\nabla_u\left(f(q+tv)-f(q)\right)-\nabla_u f(q)}{t} \ &= \lim{t \rightarrow 0} \frac{\left(\nabla f\right){q+tv}(u) \cdot v – \left(\nabla f\right)q(u) \cdot v}{t} \ &= \lim{t \rightarrow 0} \frac{\left(\nabla f\right){q+tv}(u) – \left(\nabla f\right)q(u)}{t} \cdot v \ &= \left(\nabla_v \left(\nabla f\right)q(u)\right) \ &= \left(\nabla_v \left(\lim{s \rightarrow 0} \frac{f(q+su)-f(q)}{s}\right)q(u)\right) \ &= \left(\nabla_v \left(\lim{s \rightarrow 0} \frac{\phi(s,0)-\phi(0,0)}{s}\right)\right){(0,0)} \ &= \left(\partial_2 \left(\lim_{s \rightarrow 0} \frac{\phi(s,0)-\phi(0,0)}{s}\right)\right){(0,0)} \ &= \left(\partial_2 \partial_1 \phi\right){(0,0)} \ &= \left(\partial_1 \partial_2 \phi\right)_{(0,0)} \end{align*}
Therefore, $\left(\nabla_u \nabla_v f\right)q=\left(\partial_1 \partial_2 \phi\right){(0,0)}$, as desired.
12-5. Show: Let $V, W \in \mathrm{TS}, f: V \rightarrow W, q \in \mathbb{D}_f^{\prime \prime}, u, v \in V$.
Then: $\quad\left(\nabla_u \nabla_v f\right)_q=\left(\nabla_v \nabla_u f\right)_q$.
To prove the equality $\left(\nabla_u \nabla_v f\right)_q=\left(\nabla_v \nabla_u f\right)_q$, we use the definition of the second derivative of a smooth map $f: V \rightarrow W$ between two tangent spaces $V$ and $W$. Let $\gamma: (-1,1) \rightarrow V$ be a smooth curve with $\gamma(0) = q$ and $\gamma'(0) = u$, and let $\delta: (-1,1) \rightarrow V$ be a smooth curve with $\delta(0) = q$ and $\delta'(0) = v$. Then we define
$$
\left(\nabla_u \nabla_v f\right)q=\left(\left.\frac{d}{d t}\right|{t=0} \nabla_v\left(\gamma^{\prime}(t)\right) f\right)q $$ and $$ \left(\nabla_v \nabla_u f\right)_q=\left(\left.\frac{d}{d t}\right|{t=0} \nabla_u\left(\delta^{\prime}(t)\right) f\right)_q
$$
Our goal is to show that these two expressions are equal.
First, we evaluate $\nabla_v (\gamma'(t))f$: \begin{align*} \nabla_v (\gamma'(t))f &= \left(\frac{d}{ds}\Big|{s=0} (\gamma'(t)+sv)f\right)q \ &= \left(\frac{d}{ds}\Big|{s=0} \frac{d}{dt}\Big|{t=0} (\gamma(t)+st)f\right)q \ &= \left(\frac{d^2}{ds,dt}\Big|{s=t=0} (\gamma(t)+st)f\right)_q \ &= \left(\nabla_u \nabla_v f\right)_q + \left(R(u,v)f\right)_q, \end{align*} where $R$ is the Riemann curvature tensor. In the second line, we used the chain rule for taking derivatives of composite functions, and in the third line we used the definition of the first derivative of $f$ and the fact that $\gamma'(0) = u$.
Similarly, we evaluate $\nabla_u (\delta'(t))f$: \begin{align*} \nabla_u (\delta'(t))f &= \left(\frac{d}{ds}\Big|{s=0} (\delta'(t)+su)f\right)q \ &= \left(\frac{d}{ds}\Big|{s=0} \frac{d}{dt}\Big|{t=0} (\delta(t)+st)f\right)q \ &= \left(\frac{d^2}{ds,dt}\Big|{s=t=0} (\delta(t)+st)f\right)_q \ &= \left(\nabla_v \nabla_u f\right)_q + \left(R(v,u)f\right)_q. \end{align*}
Since $R$ is a symmetric tensor, we have $R(u,v)f = R(v,u)f$. Therefore, we can subtract $\left(R(u,v)f\right)_q$ from both sides of the first equation and $\left(R(v,u)f\right)_q$ from both sides of the second equation to obtain

MY-ASSIGNMENTEXPERT™可以为您提供UNIVERSITY OF ILLINOIS URBANA-CHAMPAIGN MATH2940 linear algebra线性代数课程的代写代考和辅导服务! 请认准MY-ASSIGNMENTEXPERT™. MY-ASSIGNMENTEXPERT™为您的留学生涯保驾护航。