如果你也在 怎样代写离散数学Discrete Mathematics MA210这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。离散数学Discrete Mathematics是数学的一个分支,研究一般代数环境中的同源性。它是一门相对年轻的学科,其起源可以追溯到19世纪末的组合拓扑学(代数拓扑学的前身)和抽象代数(模块和共轭理论)的研究,主要是由亨利-庞加莱和大卫-希尔伯特提出。
离散数学Discrete Mathematics是研究同源漏斗和它们所带来的复杂的代数结构;它的发展与范畴理论的出现紧密地联系在一起。一个核心概念是链复合体,可以通过其同调和同调来研究。它在代数拓扑学中发挥了巨大的作用。它的影响逐渐扩大,目前包括换元代数、代数几何、代数理论、表示理论、数学物理学、算子矩阵、复分析和偏微分方程理论。K理论是一门独立的学科,它借鉴了同调代数的方法,正如阿兰-康尼斯的非交换几何一样。
离散数学Discrete Mathematics代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。 最高质量的离散数学Discrete Mathematics作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此离散数学Discrete Mathematics作业代写的价格不固定。通常在专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
同学们在留学期间,都对各式各样的作业考试很是头疼,如果你无从下手,不如考虑my-assignmentexpert™!
my-assignmentexpert™提供最专业的一站式服务:Essay代写,Dissertation代写,Assignment代写,Paper代写,Proposal代写,Proposal代写,Literature Review代写,Online Course,Exam代考等等。my-assignmentexpert™专注为留学生提供Essay代写服务,拥有各个专业的博硕教师团队帮您代写,免费修改及辅导,保证成果完成的效率和质量。同时有多家检测平台帐号,包括Turnitin高级账户,检测论文不会留痕,写好后检测修改,放心可靠,经得起任何考验!
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
我们在数学Mathematics代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在离散数学Discrete Mathematics代写方面经验极为丰富,各种离散数学Discrete Mathematics相关的作业也就用不着 说。

数学代写|离散数学代写Discrete Mathematics代考|The RSA Cryptosystem
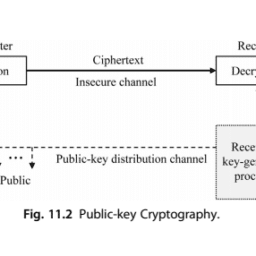
The RSA, named after the initials of its inventors, is the most widely used public-key cryptography for secure data transmission. Its effectiveness is based on the fact that very large prime numbers are fairly easy to produce on a computer, but it is enormously difficult (i.e., extremely time-consuming) to factor a product of two large unknown prime numbers. Digital envelopes and digital signatures are two important applications of RSA. However, these two security functions are mutually independent (i.e., neither, either, or both can be applied to a message). A digital envelope is the electronic equivalent of putting the message into a sealed envelope to provide privacy and prevent unauthorized alterations. A digital signature is the electronic equivalent of signing and sealing the letter. The digital envelope protects the digital signature.
The following steps show how the RSA keys can be generated:
(i) Choose two very large prime numbers $p$ and $q$ at random, on the order of a couple of hundred digits each, often using probabilistic computer algorithms, and calculate $n=p q$. As the number of digits in $n$ is approximately equal to the sum of digits in $p$ and $q$, no computer currently can factor it in a reasonable length of time. Note that due to the nature of factoring algorithms, $p$ and $q$ need to be of similar size to keep the RSA system secure. In addition, the large numbers are stored in binary form and generally 2048 to 4096 bits are needed for $n$ to ensure a reasonably high degree of security.
(ii) Compute $\varphi(n)=(p-1)(q-1)$, and choose an arbitrary integer $e$ satisfying $1<$ $e<\varphi(n)$, which is relatively prime to $\varphi(n)$. In other words, $\operatorname{gcd}(e, \varphi(n))=1$. Although for some applications, such as making encryption faster on small devices like smart cards, it is desirable to have small values of $e$, it is best not to choose a small value for $e$, as the secrecy of the cipher may then be compromised.
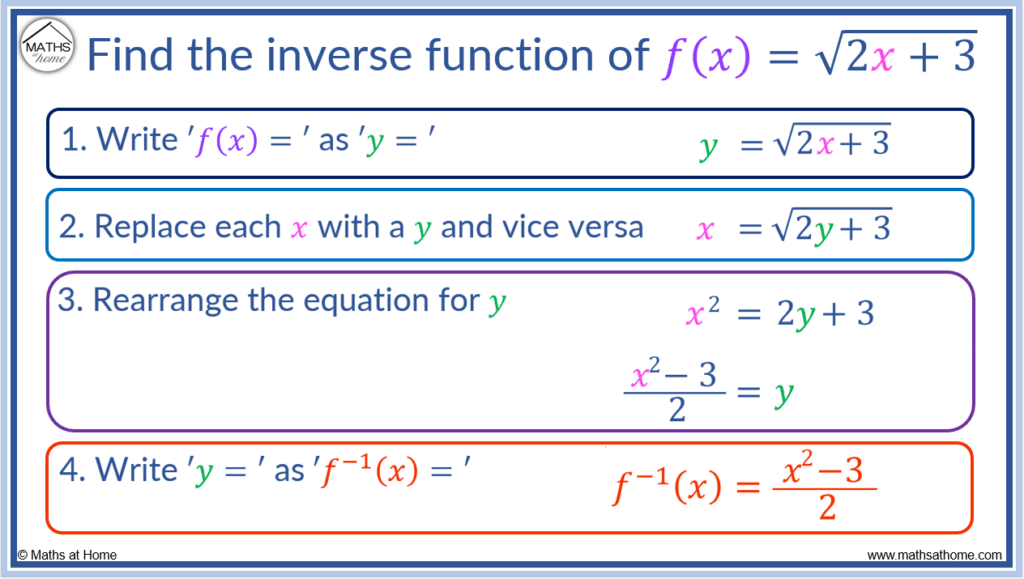
(iii) Find the positive integer $d$, an inverse of $e$ modulo $\varphi(n)$, that is, find an integer $d$ such that $e d \equiv 1(\bmod \varphi(n))$. Note that as $\operatorname{gcd}(e, \varphi(n))=1$, we have $d e+c \varphi(n)=1$, where the integers $c$ and $d$ can be found using the extended Euclidean algorithm, with the condition that $0<d<\varphi(n)$.
数学代写|离散数学代写Discrete Mathematics代考|Algorithm Requirements
An algorithm is a finite unambiguous sequence of instructions, set of rules, or number of steps that involves repetition of an operation or reiteration of a procedure for performing a computation, solving a mathematical problem, or accomplishing some end in a finite amount of time. Some of the important requirements for an algorithm are as follows:
An algorithm has input values from a specified set.
An algorithm produces the output values from the input values, which is the solution to a problem.
An algorithm possesses finiteness, that is, it produces the output after a finite number of steps.
An algorithm possesses definiteness, that is, all steps of the algorithm are precisely defined using unambiguous, well-defined operations.
An algorithm possesses correctness, that is, it produces correct output values for any set of input values.
An algorithm possesses effectiveness, that is, it performs each step precisely and in a finite amount of time, and no step can be impossible to do, such as division by zero.
An algorithm is well-ordered, as a computer can only execute an algorithm if it knows the exact order of steps to perform.
An algorithm possesses generality, that is, it should accept any general set of input values.

离散数学代写
数学代写|离散数学代写DISCRETE MATHEMATICS代考|THE RSA CRYPTOSYSTEM
RSA 以其发明者名字的首字母命名,是用于安全数据传输的最广泛使用的公钥密码术。它的有效性是基于这样一个事实,即非常大的素数很容易 在计算机上产生,但它非常困难i.e.,extremelytime – consuming分解两个大的末知质数的乘积。数字信封和数字签名是RSA的两个重要应 用。但是,这两个安全功能是相互独立的i.e., neither, either, orbothcanbeappliedtoamessage. 数字信封是将消息放入密封信封的电子等价 物,以提供隐私并防止末经授权的更改。数字签名相当于在信件上签名和盖章。数字信封保护数字签名。
以下步㗫显示了如何生成 RSA 密钥:
$i$ 选择两个非常大的素数 $p$ 和 $q$ 随机地,每个大约几百个数字,通常使用概率计算机算法,并计算 $n=p q$. 作为中的位数 $n$ 约等于中的数字之和 $p$ 和 $q$ ,目前没有计算机可以在合理的时间内将其分解。请注意,由于分解算法的性质, $p$ 和 $q$ 需要具有相似的大小以保证 RSA 系统的安全。另外,大数 是以二进制形式存储的,一般需要2048到4096位 $n$ 以确保相当高的安全性。
$i i$ 计算 $\varphi(n)=(p-1)(q-1)$, 并选择一个任意整数 $e$ 令人满意 $1<e<\varphi(n)$, 相对质数 $\varphi(n)$. 换句话说, $\operatorname{gcd}(e, \varphi(n))=1$. 尽管对于某些应用程 序,例如在智能卡等小型设备上加快加密速度,希望具有较小的值e,最好不要选择小的值 $e$ ,因为密码的保密性可能会受到损害。
iii找出正整数 $d$, 的倒数 e模块 $\varphi(n)$ ,即求一个整数 $d$ 这样 $e d \equiv 1(\bmod \varphi(n))$. 请注意,作为 $\operatorname{gcd}(e, \varphi(n))=1$ ,我们有 $d e+c \varphi(n)=1$ ,其中整 数c和 $d$ 可以使用扩展欧几里德算法找到,条件是 $0<d<\varphi(n)$.
数学代写|离散数学代写DISCRETE MATHEMATICS代考|ALGORITHM REQUIREMENTS
算法是有限明确的指令序列、规则集或步骤数,涉及重复操作或重复执行计算、解决数学问题或在有限时间内完成某个目标的过程。算法的一些重要要求如下:
算法具有来自指定集合的输入值。
算法根据输入值生成输出值,这是问题的解决方案。
算法具有有限性,即它在有限步后产生输出。
算法具有确定性,即算法的所有步骤都使用明确的、定义明确的操作精确定义。
算法具有正确性,即对任意一组输入值都能产生正确的输出值。
一个算法具有有效性,即它在有限的时间内精确地执行每一步,并且没有任何一步是不可能的,例如除零。
算法是有序的,因为计算机只有在知道要执行的步骤的确切顺序时才能执行算法。
算法具有通用性,即它应该接受任何通用的输入值集。

数学代写|离散数学代写Discrete Mathematics代考 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。
微观经济学代写
微观经济学是主流经济学的一个分支,研究个人和企业在做出有关稀缺资源分配的决策时的行为以及这些个人和企业之间的相互作用。my-assignmentexpert™ 为您的留学生涯保驾护航 在数学Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在图论代写Graph Theory代写方面经验极为丰富,各种图论代写Graph Theory相关的作业也就用不着 说。
线性代数代写
线性代数是数学的一个分支,涉及线性方程,如:线性图,如:以及它们在向量空间和通过矩阵的表示。线性代数是几乎所有数学领域的核心。
博弈论代写
现代博弈论始于约翰-冯-诺伊曼(John von Neumann)提出的两人零和博弈中的混合策略均衡的观点及其证明。冯-诺依曼的原始证明使用了关于连续映射到紧凑凸集的布劳威尔定点定理,这成为博弈论和数学经济学的标准方法。在他的论文之后,1944年,他与奥斯卡-莫根斯特恩(Oskar Morgenstern)共同撰写了《游戏和经济行为理论》一书,该书考虑了几个参与者的合作游戏。这本书的第二版提供了预期效用的公理理论,使数理统计学家和经济学家能够处理不确定性下的决策。
微积分代写
微积分,最初被称为无穷小微积分或 “无穷小的微积分”,是对连续变化的数学研究,就像几何学是对形状的研究,而代数是对算术运算的概括研究一样。
它有两个主要分支,微分和积分;微分涉及瞬时变化率和曲线的斜率,而积分涉及数量的累积,以及曲线下或曲线之间的面积。这两个分支通过微积分的基本定理相互联系,它们利用了无限序列和无限级数收敛到一个明确定义的极限的基本概念 。
计量经济学代写
什么是计量经济学?
计量经济学是统计学和数学模型的定量应用,使用数据来发展理论或测试经济学中的现有假设,并根据历史数据预测未来趋势。它对现实世界的数据进行统计试验,然后将结果与被测试的理论进行比较和对比。
根据你是对测试现有理论感兴趣,还是对利用现有数据在这些观察的基础上提出新的假设感兴趣,计量经济学可以细分为两大类:理论和应用。那些经常从事这种实践的人通常被称为计量经济学家。
Matlab代写
MATLAB 是一种用于技术计算的高性能语言。它将计算、可视化和编程集成在一个易于使用的环境中,其中问题和解决方案以熟悉的数学符号表示。典型用途包括:数学和计算算法开发建模、仿真和原型制作数据分析、探索和可视化科学和工程图形应用程序开发,包括图形用户界面构建MATLAB 是一个交互式系统,其基本数据元素是一个不需要维度的数组。这使您可以解决许多技术计算问题,尤其是那些具有矩阵和向量公式的问题,而只需用 C 或 Fortran 等标量非交互式语言编写程序所需的时间的一小部分。MATLAB 名称代表矩阵实验室。MATLAB 最初的编写目的是提供对由 LINPACK 和 EISPACK 项目开发的矩阵软件的轻松访问,这两个项目共同代表了矩阵计算软件的最新技术。MATLAB 经过多年的发展,得到了许多用户的投入。在大学环境中,它是数学、工程和科学入门和高级课程的标准教学工具。在工业领域,MATLAB 是高效研究、开发和分析的首选工具。MATLAB 具有一系列称为工具箱的特定于应用程序的解决方案。对于大多数 MATLAB 用户来说非常重要,工具箱允许您学习和应用专业技术。工具箱是 MATLAB 函数(M 文件)的综合集合,可扩展 MATLAB 环境以解决特定类别的问题。可用工具箱的领域包括信号处理、控制系统、神经网络、模糊逻辑、小波、仿真等。