MY-ASSIGNMENTEXPERT™可以为您提供sites.google MA307 Riemann surface黎曼曲面课程的代写代考和辅导服务!
这是印度科學理工學院黎曼曲面课程的代写成功案例。
MA307课程简介
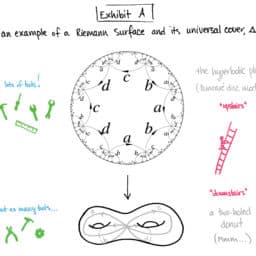
Course description: Riemann surfaces are one-dimensional complex manifolds, obtained by gluing together pieces of the complex plane by holomorphic maps. This course will be an introduction to the the theory of Riemann surfaces, with an emphasis on analytical and topological aspects. After describing examples and constructions of Riemann surfaces, the topics covered would include branched coverings and the Riemann-Hurwitz formula, holomorphic 1-forms and periods, the Weyl’s Lemma and existence theorems, the Hodge decomposition theorem, Riemann’s bilinear relations, Divisors, the Riemann-Roch theorem, theorems of Abel and Jacobi, the Uniformization theorem, Fuchsian groups and hyperbolic surfaces.
Prerequisites
There will be no class on January 5th (Tuesday).
There will be no class on December 17th (Thursday).
There will be no class on November 19th (Thursday). Also, no lectures would be held in the midterm week of Nov 23 -27.
A new Teams code has been emailed to the mailing list.
There will be weekly homework also.
There will be no lecture on Tuesday, October 6th.
Lectures will be held on Tuesdays and Thursdays, 3:30-5pm.
Our first meeting will be held on Thursday October 1st , 3:30-5pm.
Classes will be online, at the beginning over Microsoft Teams. The first meeting link will be put up here, and on the IISc Intranet Bulletin board, before the meeting time.
MA307 Riemann surface HELP(EXAM HELP, ONLINE TUTOR)
Let $f$ be a harmonic function on $\mathbb{C}$ that is square-integrable (i.e. $f \in$ $\left.\mathrm{L}^2(\mathbb{C})\right)$. Then $f$ is identically zero.
Let $M$ be a compact Riemann surface. There is a non-zero harmonic differential on $M$ which is exact.
Suppose $\alpha$ is a real-valued harmonic 1 -form on a Riemann surface $M$. Then there is a unique harmonic 1-form $\beta$ such that $\omega=\alpha+i \beta$ is a holomorphic differential on $M$.
If $f, g$ are smooth and compactly-supported functions on $M$, then $\langle d f, * d g\rangle=0$
Let $\omega \in \Omega^1(M ; \mathbb{R})$ be a closed 1 -form such that $\langle\omega, \beta\rangle=0$ for any co-closed form $\beta$. Then $\omega$ is exact.
Show that if $g=0$, then the only holomorphic differential on $M$ is the zero differential. (This is a case we skipped in class. Note that the

MY-ASSIGNMENTEXPERT™可以为您提供SITES.GOOGLE MA307 RIEMANN SURFACE交换代数课程的代写代考和辅导服务!