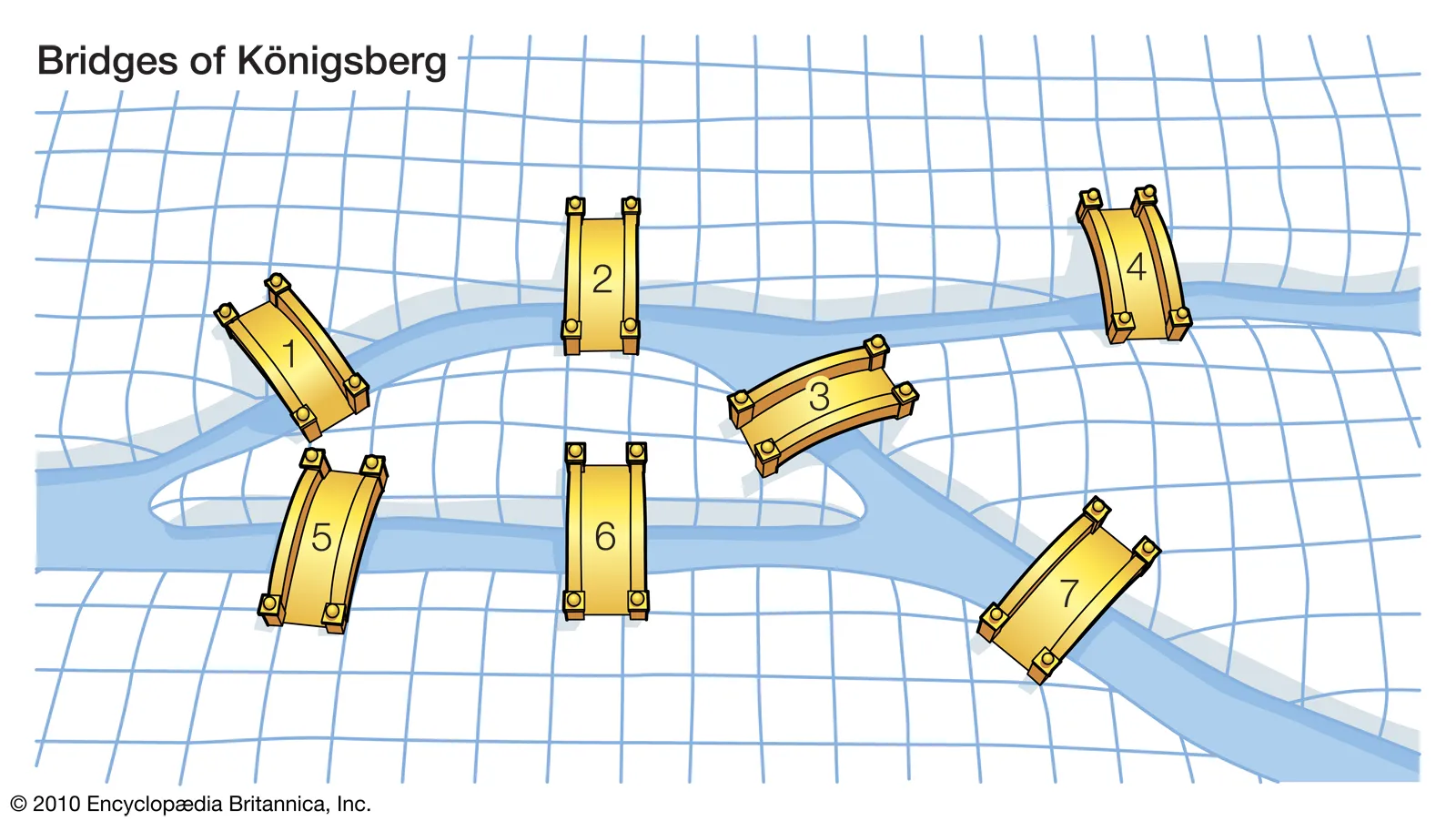
MY-ASSIGNMENTEXPERT™可以为您提供 suny MATH3020 Graph Theory图论的代写代考和辅导服务!
这是帝国州大学 图论课程的代写成功案例。
MATH3020课程简介
You will learn the same curriculum as our on-campus students
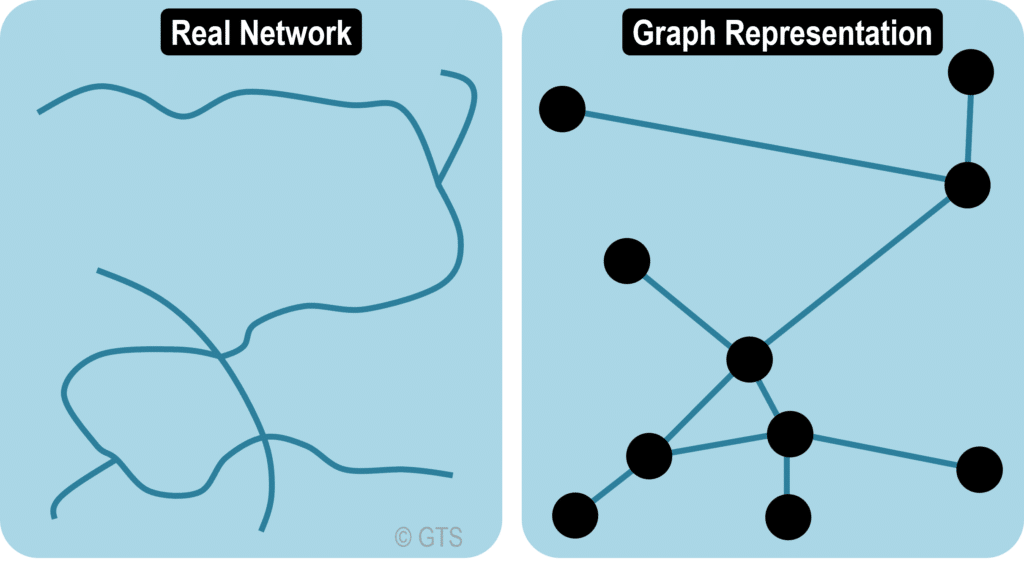
Topics covered in this course include: graphs as models, paths, cycles, directed graphs, trees, spanning trees, matchings (including stable matchings, the stable marriage problem and the medical school residency matching program), network flows, and graph coloring (including scheduling applications). Students will explore theoretical network models, such as random graphs, small world models and scale-free networks, as well as networked datasets from social, infrastructure and information networks, the role of strong and weak ties, triadic closure, and centrality measures, as well as the fragility of networked systems and contagious process on networks of various topologies. Prerequisites: Discrete Math Foundations of mathematics and mathematical proof: logic, methods of proof (both inductive and deductive), sets, relations and functions. This knowledge may be obtained from a course such as Discrete Mathematics, for example. This course was previously SMT-273244.
Prerequisites
About our University College
Our university college is a leader in undergraduate instruction. We provide bachelor’s and master’s degrees in liberal arts and sciences and professional disciplines. Student success is our primary focus as evidenced by faculty and staff interactions through honors programs, independent study opportunities, research and study abroad.
MATH3020 Graph Theory HELP(EXAM HELP, ONLINE TUTOR)
Prove that every tree with maximum degree $\Delta>1$ has at least $\Delta$ leaves. Show that this is best possible by constructing an $n$ vertex tree with exactly $\Delta$ leaves, for every choice of $n, \Delta$ with $n>\Delta \geq 2$.
Let $e$ be an edge in a connected graph $G$. Prove that $e$ is a cut-edge of $G$ if and only if $e$ belongs to any spanning-tree of $G$.
(i) Prove that if $G$ is a simple graph with $n$ vertices and
$$
\sum_{v \in V(G)}\left(\begin{array}{c}
d(v) \
2
\end{array}\right)>(m-1)\left(\begin{array}{l}
n \
2
\end{array}\right)
$$
then $G$ contains $K_{2, m}$.
(ii) Use (i) to prove that for $n \geq 4$, every $n$-vertex graph with more than $2 n^{3 / 2}$ edges has girth at most 4 .
For $n \geq 4$ let $G$ be a simple $n$-vertex graph with $e(G) \geq 2 n-3$. Prove that $G$ has two cycles of equal length.
Let $T$ be a tree. Prove that the vertices of $T$ all have odd degree if and only if for all $e \in E(T)$, both components of $T-e$ have odd order (where the order of a component is the number of its vertices).
MY-ASSIGNMENTEXPERT™可以为您提供 suny MATH3020 Graph Theory图论的代写代考和辅导服务!