如果你也在 怎样代写传感器Sensor TFE4202这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。传感器Sensor是一种产生输出信号的设备,其目的是感知一种物理现象。在最广泛的定义中,传感器是一个设备、模块、机器或子系统,它检测其环境中的事件或变化,并将信息发送到其他电子产品,通常是计算机处理器。传感器总是与其他电子产品一起使用。
传感器Sensor被用于日常用品,如触摸式电梯按钮(触觉传感器)和通过触摸底座而变暗或变亮的灯,以及大多数人从未意识到的无数应用中。随着微型机械和易于使用的微控制器平台的进步,传感器的用途已经超出了温度、压力和流量测量的传统领域,例如,扩展到MARG传感器。模拟传感器,如电位计和力敏电阻仍然被广泛使用。它们的应用包括制造业和机械、飞机和航空航天、汽车、医学、机器人和我们日常生活的许多其他方面。还有其他广泛的测量材料化学和物理特性的传感器,包括用于测量折射率的光学传感器,用于测量流体粘度的振动传感器,以及用于监测流体pH值的电-化学传感器。
传感器Sensor代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。最高质量的传感器Sensor作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此传感器Sensor作业代写的价格不固定。通常在专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
同学们在留学期间,都对各式各样的作业考试很是头疼,如果你无从下手,不如考虑my-assignmentexpert™!
my-assignmentexpert™提供最专业的一站式服务:Essay代写,Dissertation代写,Assignment代写,Paper代写,Proposal代写,Proposal代写,Literature Review代写,Online Course,Exam代考等等。my-assignmentexpert™专注为留学生提供Essay代写服务,拥有各个专业的博硕教师团队帮您代写,免费修改及辅导,保证成果完成的效率和质量。同时有多家检测平台帐号,包括Turnitin高级账户,检测论文不会留痕,写好后检测修改,放心可靠,经得起任何考验!
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
我们在物理Physical代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的物理Physical代写服务。我们的专家在声学Acoustics代写方面经验极为丰富,各种声学Acoustics相关的作业也就用不着说。
物理代写|传感器代写Sensor代考|The Role of Errors
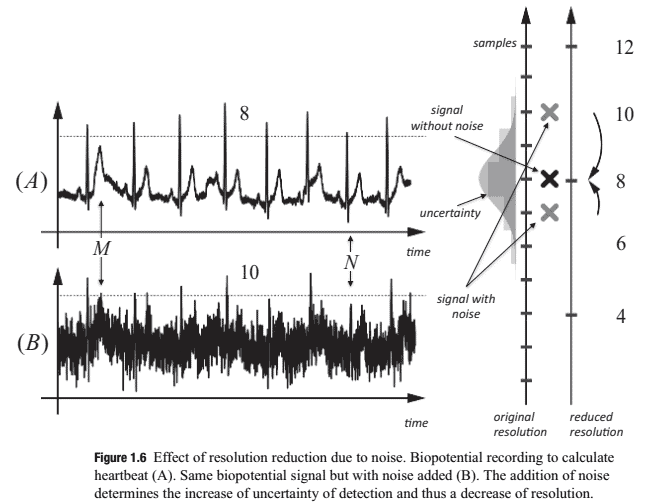
Unfortunately, the sensing process’s physical implementation is necessarily affected by errors due to the stochastic nature of random processes and nonidealities. Errors arise from either the environment or the sensing system itself and its nonperfect detection capabilities. Let us look at Fig. 1.6, where a biopotential is used to detect heartbeats utilizing a threshold as in Fig. 1.4A. In the absence of noise, during the timelapse, we measure 8 beats, as shown in Fig. 1.6A. Now, assume that the sensing process is noisy and the same waveform as in Fig. 1.6A with added noise is shown in Fig. 1.6B. If we use the same detection approach, the count is no longer 8 but rather 10 . The random process of noise changes the threshold crossing cases: there are some points (e.g., point M) that were not crossing the threshold before (without noise), while now they do, thanks to noise contribution. Conversely, there are other points (e.g., point $\mathrm{N}$ ) that were crossing the threshold in the previous case, and now they do not pass the level because of the perturbation of noise. If we repeat the same procedure on a signal containing 8 beats in the presence of the noise, we may at one time count 7 , another time 9, another time again 8 , and so on. This means that we cannot say that the count is certain but, in the presence of noise, we can say that the “estimation of the count is given by $8 \pm 2$.” Therefore, the presence of noise determines an uncertainty (Chapter 2) of the measure of $\pm 2$ counts. The uncertainty due to noise could be visualized in the gray area across the tick equal to 8 in Fig. 1.6.
In this example, the presence of errors (or noise) changes the information space situation. If we could have counted single beats without noise before, now we have an uncertainty of $\pm 2$ counts. Therefore, previous levels are no longer truly distinguishable from each other because the same signal could give counts in the interval $8 \pm 2$ due to noise. This fact reveals that the information space subdivision is not appropriate because the sentence “count $=8$ ” has information similar to that one of “count $=10$.” This results in a misclassification because we classify 8 counts, although, in reality, they might be 10 or vice versa. This means that we have a high probability that the affirmations mentioned above reflect the same signal condition due to errors. This could be seen pictorially showing that the same uncertainty area covers samples. Therefore, it could be better to reduce the number of subdivisions (e.g., by grouping 4 levels) so that “count is between 6 and 10” and “count is between 2 and 6 ” has more significance from the information point of view because there is a lower probability that the two sentences correspond to the same signal. In this case, any sample giving a value in the uncertainty zone, identified by $8 \pm 2$, will be associated with the center of the interval, whose value is 8 . In other words, by enlarging the classification zones by considering uncertainty, we reduce possible misclassification errors.
物理代写|传感器代写Sensor代考|Essential Building Blocks of Electronic Sensors
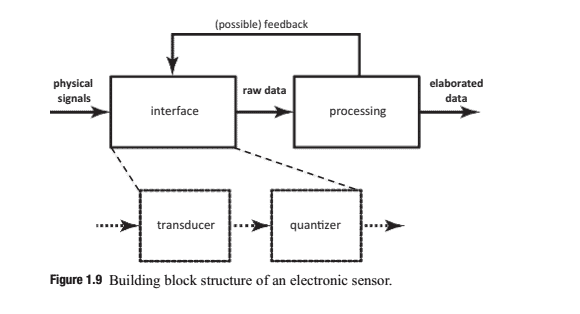
A preliminary and necessary step of the electronic sensing process is the transduction of the physical stimulus into electronic signals. This is performed by a transducer interface, which converts the energy of physical signals into electronic states. Then, data should be organized and elaborated to extract the information depending on the specific task. This process could be performed by a system that follows the transducer and computes elaborations on the previous stage’s raw data. The higher the complexity of information, the higher the “intelligence” (computational complexity) required by this block to achieve the result.
We can draw a general scheme of an electronic sensor structure illustrated in Fig. 1.9. Two parts could describe a generic sensor: the interface and the processing machine. The first one is devoted to the transduction and digitization of the signal, composed of two subblocks: the transducer and the quantizer. The transducer is the block that directly connects with the physical environment. It could be a simple amplifier or a more complex structure operating in the time or frequency domain. The quantizer is a block that is necessary to operate in the binary domain on which the elaboration block will work. A typical quantizer is an A/D converter, even if other digitizers might be used (Chapter 8 ).
A processing machine implements the second part to gain the maximum information from raw data. The main differences between the two parts can be summarized. The transducer converts the energy/power levels of physical signal states into raw data. Therefore, it acts as an energy detector, and the design should be mainly focused on optimization with respect to the energetic content of signals. One of the references of this optimization is the signal-to-noise ratio. Conversely, the processor searches the raw data’s information to achieve the final task required by the sensor application. It makes complex elaborations of raw data that earlier stages cannot treat. For example, we can implement analog filters in the first blocks to optimize the signal-to-noise ratio, but we need data elaboration to implement complex algorithms, for example, Kalman’s filters or machine learning classifiers.
传感器代写
物理代写|传感器代写SENSOR代考|THE ROLE OF ERRORS
不幸的是,由于随机过程的随机性和非理想性,传崗过程的物理实现必然会受到误差的影响。错误来自环境或传感系统本身及其不完善的检测能力。让我们看一下 图 1.6,其中生物电势用于利用图 1.4A中的呵值检测心跳。在没有噪音的情况下,在游戏中时光倒流期间,我们测量了 8 个节拍,如图 $1.6 \mathrm{~A}$ 所示。现在,假设传感 过程有噪声,图 1.6B 显示了与图 1.6A 相同的波形,添加了噪声。如果我们使用相同的检测方法,计数不再是 8 而是 10 。噪声的随机过程改变了呵值交叉的情况: 有一些点e. g., pointM之前没有跨过门槛withoutnoise,而现在他们这样做了,这要归功于噪音的贡献。反之,还有其他点e. $g .$, point $\$ N \$$ 在前一种情况下越过 阈值,现在由于噪声的扰动而没有通过水平。如果我们在存在璪声的情况下对包含 8 拍的信号重复相同的过程,我们可能会一次数 7 ,另一次数 9 ,再数一次 8 , 依此类推。这意味着我们不能说计数是确定的,但是在存在噪声的情况下,我们可以说“计数的估计由下式给出 $8 \pm 2$ 。”因此,噪声的存在决定了不确定性 Chapter 2 的措施 $\pm 2$ 计数。噪声引起的不确定性可以在图 $1.6$ 中等于 8 的刻度的灰色区域中可视化。
在这个例子中,错误的存在ornoise改变信息空间状况。如果我们以前可以在没有噪音的情况下计算单个节拍,那么现在我们有一个不确定性 $\pm 2$ 计数。因此,以前 的级别不再能够真正区分彼此,因为相同的信号可以在区间内给出计数 $8 \pm 2$ 由于噪音。这一事实表明,信息空间细分是不合适的,因为句子”count $=8$ “具有与 “count””类似的信息 $=10$ 。 这会导致错误分类,因为我们对 8 个计数进行了分类,尽管实际上它们可能是 10 个,反之亦然。这意味着我们很有可能上面提到的确 认由于错误而反映了相同的信号条件。这可以从图形上看出,相同的不确定性区域䙲盖了样本。因此,最好减少细分的数量e. g., bygrouping4levels因此”count is between 6 and 10″和“count is between 2 and 6″从信息的角度来看具有更大的意义,因为这两个句子对应相同信号的概率较低。在这种情况下,任何在不确定区域中 给出值的样本,由 $8 \pm 2$, 将与区间的中心相关联,其值为 8 。换句话说,通过考虑不确定性来扩大分类区域,我们减少了可能的错误分类错误。
物理代写|传感器代写SENSOR代考|ESSENTIAL BUILDING BLOCKS OF ELECTRONIC SENSORS
电子传感过程的一个初步和必要的步骤是将物理刺激转换为电子信号。这是由传感器接口执行的,该接口将物理信号的能量转换为电子状态。然后,应根据具体任 务组织和详细说明数据以提取信息。这个过程可以由一个跟随换能器并计算前一阶段原始数据的详细信息的系統来执行。信息的复杂程度越高,“智能”就越高 computationalcomplexity此块所需的以实现絡果。
我们可以画出图 $1.9$ 所示的电子传感器结构的一般方室。两个部分可以描述通用传感器:接口和处理机器。第一个专门用于信号的转换和数字化,由两个子模块组 成:换能器和量化器。换能器是直接与物理环境相连的块。它可以是一个简单的放大器,也可以是在时域或频域中运行的更复杂的结构。量化器是在精化块将在其 上工作的二进制域中操作所必需的块。典型的量化器是 $\mathrm{A} / \mathrm{D}$ 转换器,即使可能使用其他数字化器Chapter 8 .
处理机碞执行第二部分以从原始数据中获取最大信息。可以总结出这两部分的主要区别。传感器将物理信号状态的能量/功率水平转换为原始数据。因此,它充当 能量检测器,设计应主要集中在信号能量含量的优化上。这种优化的参考之一是信噪比。相反,处理器搜索原始数据的信息以完成传感器应用程序所需的最終任 务。它对早期阶段无法处理的原始数据进行复杂的阐述。例如,我们可以在第一个块中实现模拟滤波器以优化信噪比,但我们需要数据细化来实现复杂的算法,例 如,

物理代写|传感器代写Sensor代考 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。
微观经济学代写
微观经济学是主流经济学的一个分支,研究个人和企业在做出有关稀缺资源分配的决策时的行为以及这些个人和企业之间的相互作用。my-assignmentexpert™ 为您的留学生涯保驾护航 在数学Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在图论代写Graph Theory代写方面经验极为丰富,各种图论代写Graph Theory相关的作业也就用不着 说。
线性代数代写
线性代数是数学的一个分支,涉及线性方程,如:线性图,如:以及它们在向量空间和通过矩阵的表示。线性代数是几乎所有数学领域的核心。
博弈论代写
现代博弈论始于约翰-冯-诺伊曼(John von Neumann)提出的两人零和博弈中的混合策略均衡的观点及其证明。冯-诺依曼的原始证明使用了关于连续映射到紧凑凸集的布劳威尔定点定理,这成为博弈论和数学经济学的标准方法。在他的论文之后,1944年,他与奥斯卡-莫根斯特恩(Oskar Morgenstern)共同撰写了《游戏和经济行为理论》一书,该书考虑了几个参与者的合作游戏。这本书的第二版提供了预期效用的公理理论,使数理统计学家和经济学家能够处理不确定性下的决策。
微积分代写
微积分,最初被称为无穷小微积分或 “无穷小的微积分”,是对连续变化的数学研究,就像几何学是对形状的研究,而代数是对算术运算的概括研究一样。
它有两个主要分支,微分和积分;微分涉及瞬时变化率和曲线的斜率,而积分涉及数量的累积,以及曲线下或曲线之间的面积。这两个分支通过微积分的基本定理相互联系,它们利用了无限序列和无限级数收敛到一个明确定义的极限的基本概念 。
计量经济学代写
什么是计量经济学?
计量经济学是统计学和数学模型的定量应用,使用数据来发展理论或测试经济学中的现有假设,并根据历史数据预测未来趋势。它对现实世界的数据进行统计试验,然后将结果与被测试的理论进行比较和对比。
根据你是对测试现有理论感兴趣,还是对利用现有数据在这些观察的基础上提出新的假设感兴趣,计量经济学可以细分为两大类:理论和应用。那些经常从事这种实践的人通常被称为计量经济学家。
Matlab代写
MATLAB 是一种用于技术计算的高性能语言。它将计算、可视化和编程集成在一个易于使用的环境中,其中问题和解决方案以熟悉的数学符号表示。典型用途包括:数学和计算算法开发建模、仿真和原型制作数据分析、探索和可视化科学和工程图形应用程序开发,包括图形用户界面构建MATLAB 是一个交互式系统,其基本数据元素是一个不需要维度的数组。这使您可以解决许多技术计算问题,尤其是那些具有矩阵和向量公式的问题,而只需用 C 或 Fortran 等标量非交互式语言编写程序所需的时间的一小部分。MATLAB 名称代表矩阵实验室。MATLAB 最初的编写目的是提供对由 LINPACK 和 EISPACK 项目开发的矩阵软件的轻松访问,这两个项目共同代表了矩阵计算软件的最新技术。MATLAB 经过多年的发展,得到了许多用户的投入。在大学环境中,它是数学、工程和科学入门和高级课程的标准教学工具。在工业领域,MATLAB 是高效研究、开发和分析的首选工具。MATLAB 具有一系列称为工具箱的特定于应用程序的解决方案。对于大多数 MATLAB 用户来说非常重要,工具箱允许您学习和应用专业技术。工具箱是 MATLAB 函数(M 文件)的综合集合,可扩展 MATLAB 环境以解决特定类别的问题。可用工具箱的领域包括信号处理、控制系统、神经网络、模糊逻辑、小波、仿真等。

