数学代写|Math1030 Discrete Mathematics

MY-ASSIGNMENTEXPERT™可以为您提供courses.math Math1030 Discrete Mathematics 离散数学的代写代考和辅导服务！

Math1030课程简介

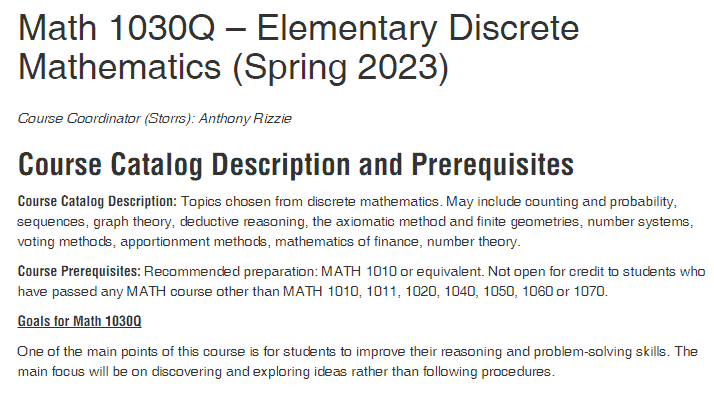
Course Catalog Description and Prerequisites
Course Catalog Description: Topics chosen from discrete mathematics. May include counting and probability, sequences, graph theory, deductive reasoning, the axiomatic method and finite geometries, number systems, voting methods, apportionment methods, mathematics of finance, number theory.

Course Prerequisites: Recommended preparation: MATH 1010 or equivalent. Not open for credit to students who have passed any MATH course other than MATH 1010, 1011, 1020,1040, 1050, 1060 or 1070.
Goals for Math 10300
One of the main points of this course is for stuclents to improve their reasoning and problem-solving skills. The main focus will be on discovering and exploring ideas rather than following procedures.

Prerequisites 

Some Advice for How to Succeed in Math 10300

Become an active participant in the class. Ask questions. Participate during class activities. Do your best to keep up with the material. Come to office hours. Work on assignments as soon as they are assigned.

Spend some time reading and engaging with the textbook. It is better to use a notebook to keep track of your progress. For instance, one could write down questions that come up and, so, it would be easy to come back to them at some point. It is also a good idea to write down important concepts in order to involve all types of memory during the studying process. In

Work with other students in this class during in-class activities and on assigned problems. Also, you should try
to build a little community to discuss the issues and questions you have in and outside of class

Math1030 Discrete Mathematics HELP（EXAM HELP， ONLINE TUTOR）

Proof:

(1) Let $n = p_1^{k_1}p_2^{k_2}\cdots p_r^{k_r}$ be the prime factorization of $n$, where $p_i$’s are distinct primes and $k_i \geq 1$ for all $i$. Then, we have $n^2 = p_1^{2k_1}p_2^{2k_2}\cdots p_r^{2k_r}$.

If $p$ does not divide $n$, then $p$ does not appear in the prime factorization of $n$, and hence $p$ does not appear in the prime factorization of $n^2$. Therefore, $p$ appears an even number of times (i.e., zero times) in the prime factorization of $n^2$.

If $p$ divides $n$, then we can write $n = p^k\cdot n’$, where $n’$ is not divisible by $p$. Then, $n^2 = p^{2k}\cdot (n’)^2$. Since $p$ appears with an exponent of $2k$ in the prime factorization of $n^2$, $p$ appears an even number of times in the prime factorization of $n^2$.

Therefore, in either case, $p$ appears an even number of times (or zero times) in the prime factorization of $n^2$.

(2) Let $m = p\cdot n^2$. Using the prime factorization of $n$ as before, we can write $n = p_1^{k_1}p_2^{k_2}\cdots p_r^{k_r}$. Then, we have $m = p\cdot p_1^{2k_1}p_2^{2k_2}\cdots p_r^{2k_r}$.

If $p$ does not divide $n$, then $p$ appears only once in the prime factorization of $m$, namely with an exponent of $1$.

If $p$ divides $n$, then we can write $

Proof:

(1) Assume, for the sake of contradiction, that there exist numbers $a$ and $b$ such that $\frac{a}{b} = \sqrt{7}$. Then we have $a = b \cdot \sqrt{7}$. Squaring both sides, we get $a^2 = 7b^2$. This implies that $a^2$ is a multiple of $7$, which in turn implies that $a$ is a multiple of $7$. Let $a = 7k$, where $k$ is an integer. Then we have $49k^2 = 7b^2$, which simplifies to $b^2 = 7k^2$. This means that $b^2$ is a multiple of $7$, and hence $b$ is also a multiple of $7$. But this contradicts the assumption that $a$ and $b$ have no common factors. Therefore, there are no numbers $a$ and $b$ such that $\frac{a}{b} = \sqrt{7}$.

(2) Assume, for the sake of contradiction, that there exist numbers $a$ and $b$ such that $\frac{a}{b} = \sqrt{23}$. Then we have $a = b \cdot \sqrt{23}$. Squaring both sides, we get $a^2 = 23b^2$. This implies that $a^2$ is a multiple of $23$, which in turn implies that $a$ is a multiple of $23$. Let $a = 23k$, where $k$ is an integer. Then we have $529k^2 = 23b^2$, which simplifies to $b^2 = 23k^2$. This means that $b^2$ is a multiple of $23$, and hence $b$ is also a multiple of $23$. But this contradicts the assumption that $a$ and $b$ have no common factors. Therefore, there are no numbers $a$ and $b$ such that $\frac{a}{b} = \sqrt{23}$.

(3) Assume, for the sake of contradiction, that there exist numbers $a$ and $b$ such that $\frac{a}{b} = \sqrt{p}$, where $p$ is a prime. Then we have $a = b \cdot \sqrt{p}$. Squaring both sides, we get $a^2 = pb^2$. This implies that $a^2$ is a multiple of $p$, which in turn implies that $a$ is a multiple of $p$. Let $a = pk$, where $k$ is an integer. Then we have $p^2k^2 = pb^2$, which simplifies to $b^2 = pk^2$. This means that $b^2$ is a multiple of $p$, and hence $b$ is also a multiple of $p$. But this contradicts the assumption that $a$ and $b$ have no common factors. Therefore, there are no numbers $a$ and $b$ such that $\frac{a}{b} = \sqrt{p}$, where $p$ is a prime.

MY-ASSIGNMENTEXPERT™可以为您提供UNIVERSITY OF ILLINOIS URBANA-CHAMPAIGN MATH2940 linear algebra线性代数课程的代写代考和辅导服务！ 请认准MY-ASSIGNMENTEXPERT™. MY-ASSIGNMENTEXPERT™为您的留学生涯保驾护航。