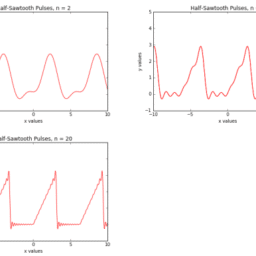
MY-ASSIGNMENTEXPERT™可以为您提供Stanford EE261 Fourier analysis傅里叶分析的代写代考和辅导服务!
这是斯坦福大学 傅里叶分析课程的代写成功案例。
EE261课程简介
The goals for the course are to gain a facility with using the Fourier transform, both specific techniques and general principles, and learning to recognize when, why, and how it is used. Together with a great variety, the subject also has a great coherence, and the hope is students come to appreciate both.
Topics include: The Fourier transform as a tool for solving physical problems. Fourier series, the Fourier transform of continuous and discrete signals and its properties. The Dirac delta, distributions, and generalized transforms. Convolutions and correlations and applications; probability distributions, sampling theory, filters, and analysis of linear systems. The discrete Fourier transform and the FFT algorithm. Multidimensional Fourier transform and use in imaging. Further applications to optics, crystallography. Emphasis is on relating the theoretical principles to solving practical engineering and science problems.
Prerequisites
e goals for the course are to gain a facility with using the Fourier transform, both specific techniques and general principles, and learning to recognize when, why, and how it is used. Together with a great variety, the subject also has a great coherence, and the hope is students come to appreciate both.
Topics include:
- The Fourier transform as a tool for solving physical problems.
- Fourier series, the Fourier transform of continuous and discrete signals and its properties.
- The Dirac delta, distributions, and generalized transforms.
- Convolutions and correlations and applications; probability distributions, sampling theory, filters, and analysis of linear systems.
- The discrete Fourier transform and the FFT algorithm.
- Multidimensional Fourier transform and use in imaging.
- Further applications to optics, crystallography.
- Emphasis is on relating the theoretical principles to solving practical engineering and science problems.
EE261 Fourier analysis HELP(EXAM HELP, ONLINE TUTOR)
Let $\varphi(x)=|x|$ for $x \in[0,1]$. Extend $\varphi$ to $\mathbb{R}$ as a 2-periodic function. Prove that the formula
$$
f(x)=\sum_{n=0}^{\infty}\left(\frac{3}{4}\right)^n \varphi\left(4^n x\right)
$$
defines a continuous function on $\mathbb{R}$.
Solution. Since $|\varphi(x)| \leqslant 1$ for all $x \in \mathbb{R}$, the series defining $f$ may be compared to the absolutely converging geometric series $\sum_{n=0}^{\infty}\left(\frac{3}{4}\right)^n$, thereby establishing that the series defining $f$ converges uniformly.
We continue to study properties of $\varphi$ defined in the first exercise. Fix $x_0 \in \mathbb{R}$. For every non-negative integer $m$ define a real number $\delta_m$ by the conditions $\left|\delta_m\right|=4^{-m} / 2$ and the sign is fixed by the condition that there are no integers between $4^m x_0$ and $4^m\left(x_0+\delta_m\right)$. Consider the quotient
$$
\gamma_n=\frac{\varphi\left(4^n\left(x_0+\delta_m\right)\right)-\varphi\left(4^n x_0\right)}{\delta_m}
$$
Prove that
a) If $n>m$ then $\gamma_n=0$.
b) If $0 \leqslant n \leqslant m$, then $\left|\gamma_n\right| \leqslant 4^n$ and $\left|\gamma_m\right|=4^m$.
Solution. a) This follows from the fact that $\varphi$ is 2-periodic and the subsequent observation that when $n>m$, we have
$$
\varphi\left(4^n\left(x+\delta_m\right)\right)=\varphi\left(4^n x \pm 2^{2(n-m)-1}\right)=\varphi\left(4^n x\right) .
$$
b) For $0 \leqslant n<m$ the inequalities follow directly from the fact that $\varphi$ is Lipschitz and in fact
$$
|\varphi(x)-\varphi(y)| \leqslant|x-y|
$$
for all $x, y \in \mathbb{R}$. Finally, the equality $\left|\gamma_m\right|=4^m$ follows from the observation that since the sign of $\delta_m$ was chosen in such a way that there are no integers between $4^m\left(x_0+\delta_m\right)$ and $4^m x_0$, the part of the graph of $\varphi$ between those values looks like a line segment of slope \pm 1 , and thus
$$
\left|\gamma_m\right|=\left|\frac{\varphi\left(4^n\left(x_0+\delta_m\right)\right)-\varphi\left(4^n x_0\right)}{\delta_m}\right|=\left|\frac{4^m\left(x_0+\delta_m\right)-4^m x_0}{\delta_m}\right|=4^m .
$$
This continues the study of $f$ and $\varphi$ defined in the previous exercises. Prove that
$$
\left|\frac{f\left(x_0+\delta_m\right)-f\left(x_0\right)}{\delta_m}\right| \geqslant \frac{1}{2}\left(3^m+1\right) .
$$
Conclude that $f$ is not differentiable at $x_0$.
Solution. We use the symbols $\gamma_0, \gamma_1, \ldots$ defined in the previous exercise.
Since $\left|\gamma_0\right| \leqslant 4^0=1$ and $\left|\gamma_m\right|=4^m$, we have
$$
\left|\left(\frac{3}{4}\right)^m \gamma_m+\gamma_0\right| \geqslant\left|\left(\frac{3}{4}\right)^m\right| \gamma_m|-| \gamma_0|| \geqslant 3^m-1 .
$$
Similarly, we may estimate
$$
\left|\sum_{n=1}^{m-1}\left(\frac{3}{4}\right)^n \gamma_n\right| \leqslant \sum_{n=1}^{m-1}\left(\frac{3}{4}\right)^n\left|\gamma_n\right| \leqslant \sum_{n=1}^{m-1} 3^n=3 \cdot \frac{3^{m-1}-1}{3-1}=\frac{3^m-3}{2} .
$$
Combining the above estimates with the part a) of the previous exercise gives the inequality
$$
\begin{aligned}
& \left|\frac{f\left(x_0+\delta_m\right)-f\left(x_0\right)}{\delta_m}\right|=\left|\sum_{n=0}^{\infty}\left(\frac{3}{4}\right)^n \gamma_n\right|=\left|\sum_{n=0}^m\left(\frac{3}{4}\right)^n \gamma_n\right| \
\geqslant & ||\left(\frac{3}{4}\right)^m \gamma_m+\gamma_0|-| \sum_{n=1}^{m-1}\left(\frac{3}{4}\right)^n \gamma_n|| \geqslant|| 3^m-1|-| \frac{3^m-3}{2}||=\frac{3^m+1}{2} .
\end{aligned}
$$
Finally, we conclude that the function $f$ can not be differentiable at $x_0$ since the difference quotient $\frac{f\left(x_0+h\right)-f\left(x_0\right)}{h}$ can not tend to a finite limit when $h$ tends to zero.

MY-ASSIGNMENTEXPERT™可以为您提供STANFORD EE261 FOURIER ANALYSIS傅里叶分析的代写代考和辅导服务!