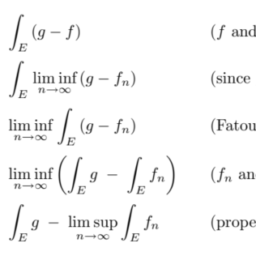如果你也在 怎样代写实分析real analysis这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。实分析real analysis是数学分析的一个分支,研究实数、实数序列和实数函数的行为。 实分析研究的实值序列和函数的一些特殊性质包括收敛性、极限、连续性、平稳性、可微分性和可整定性。实分析有别于复分析,后者涉及复数及其函数的研究。
实分析real analysis是数学中的一个经典分支,它的发展是为了使数和函数的研究正规化,并研究重要的概念,如极限和连续性。这些概念是微积分及其应用的基础。实物分析已经成为许多应用领域中不可或缺的工具。
实分析real analysis的基础知识:序列和数列的收敛性、连续性、可分性、黎曼积分、函数的序列和数列、均匀性以及极限操作的互换。
my-assignmentexpert™ 实分析real analysis作业代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。my-assignmentexpert™, 最高质量的实分析real analysis作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于统计Statistics作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此复分析complex analysis作业代写的价格不固定。通常在经济学专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
my-assignmentexpert™ 为您的留学生涯保驾护航 在数学mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学mathematics代写服务。我们的专家在实分析real analysis代写方面经验极为丰富,各种实分析real analysis相关的作业也就用不着 说。
我们提供的实分析real analysis及其相关学科的代写,服务范围广, 其中包括但不限于:
数学代写|实分析代写real analysis代考|Dense Subsets of Lp(E)
When trying to prove that some particular fact holds for all functions in $L^{p}(E)$, it is not unusual to find that it is easy to establish that the fact holds for some special subclass of functions, but it is not so clear how to prove it for arbitrary functions in $L^{p}(E)$. A standard technique in this situation is to try to extend the result from the “easy” class to the entire space by applying some type of approximation argument. Specifically, if every function in a class $S$ has a certain property $\mathbf{P}$, if $S$ is dense in $L^{p}(E)$, and if we can show that property $\mathbf{P}$ is preserved under limits in $L^{p}$-norm, then we can conclude that every function in $L^{p}(E)$ has property $\mathbf{P}$. We used this technique to prove several results about $L^{1}(E)$ in Section $4.5$; now we consider $L^{p}(E)$.
The abstract definition of density was given in Definition 1.1.5. For convenience, we restate some equivalent formulations of density for the specific case of the $L^{p}$-norm as the following result.
数学代写|实分析代写REAL ANALYSIS代考|Dense Subsets of Lp(E)).
Let $E \subseteq \mathbb{R}^{d}$ be measurable, and fix $1 \leq p \leq \infty$. If $S$ is a subset of $L^{p}(E)$, then the following three statements are equivalent.
(a) $S$ is dense in $L^{p}(E)$, i.e., the closure of $S$ equals $L^{p}(E)$.
(b) If $f$ is any element of $L^{p}(E)$, then there exist functions $f_{n} \in S$ such that $f_{n} \rightarrow f$ in $L^{p}$-norm.
(c) If $f$ is any element of $L^{p}(E)$, then for each $\varepsilon>0$ there exists a function $g \in S$ such that $|f-g|_{p}<\varepsilon$.
To illustrate, we will prove that the set of functions in $L^{p}(E)$ that are compactly supported is dense in $L^{p}(E)$ when $p$ is finite. We do need to be careful about the meaning of “support” in this context. The support of a continuous function is the closure of the set where $f$ is nonzero. This definition cannot literally be applied to elements of $L^{p}(E)$ because it depends on the choice of representative. For example, $\chi_{\mathbb{Q}}$ and the zero function are representatives of the same element of $L^{p}(\mathbb{R})$, but the closure of the set where $\chi_{\mathbb{Q}}$ is nonzero is $\mathbb{R}$, whereas the closure of the set where 0 is nonzero is the empty set. The precise definition of the support of an element of $L^{p}(E)$ is laid out in Problem $7.3 .24$, but for most purposes it is sufficient to declare, as we do next, that an element of $L^{p}(E)$ is compactly supported if it is zero a.e. outside of some compact set.
实分析代写
数学代写|实分析代写REAL ANALYSIS代考|DENSE SUBSETS OF LP和
当试图证明某些特定事实适用于所有函数时大号p(和), 很容易发现这一事实适用于某些特殊的函数子类,但对于大号p(和). 这种情况下的标准技术是尝试通过应用某种类型的近似参数将结果从“简单”类扩展到整个空间。具体来说,如果类中的每个函数小号有一定的属性磷, 如果小号密集在大号p(和), 如果我们能证明这个性质磷被保存在限制下大号p-norm,那么我们可以得出结论,大号p(和)有财产磷. 我们用这种技术证明了几个关于大号1(和)在部分4.5; 现在我们考虑大号p(和).
密度的抽象定义在定义 1.1.5 中给出。为方便起见,我们针对特定情况重述了一些等效的密度公式大号p-norm 如下结果。
数学代写|实分析代写REAL ANALYSIS代考|DENSE SUBSETS OF LP和).
让和⊆Rd可测量并修复1≤p≤∞. 如果小号是的一个子集大号p(和), 那么下面三个语句是等价的。
一种 小号密集在大号p(和),即关闭小号等于大号p(和).
b如果F是任何元素大号p(和), 那么存在函数Fn∈小号这样Fn→F在大号p-规范。
C如果F是任何元素大号p(和),那么对于每个e>0存在一个函数G∈小号这样|F−G|p<e.
为了说明,我们将证明大号p(和)紧密支撑的密集于大号p(和)什么时候p是有限的。在这种情况下,我们确实需要小心“支持”的含义。连续函数的支持是集合的闭包,其中F是非零的。这个定义不能从字面上应用到元素大号p(和)因为这取决于代表的选择。例如,χ问和零函数代表相同的元素大号p(R), 但是集合的闭包 whereχ问是非零是R,而 0 为非零的集合的闭包是空集。支持元素的精确定义大号p(和)在问题中列出7.3.24,但对于大多数目的来说,就像我们接下来要做的那样,声明一个元素就足够了大号p(和)如果它在某个紧集之外为零 ae,则得到紧支持。

数学代写|实分析代写real analysis代考 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。
离散数学代写
Partial Differential Equations代写可以参考一份偏微分方程midterm答案解析