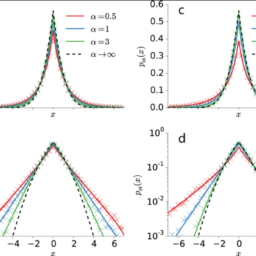
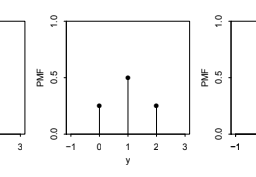
统计代写| Expectations and variances stat代写
统计代考
4.12 Exercises
Exercises marked with (S) have detailed solutions at http://stat110.net.
Expectations and variances
- Bobo, the amoeba from Chapter 2, currently lives alone in a pond. After one minute Bobo will either die, split into two amoebas, or stay the same, with equal probability. Find the expectation and variance for the number of amoebas in the pond after one
- In the Gregorian calendar, each year has either 365 days (a normal year) or 366 days (a leap year). A year is randomly chosen, with probability $3 / 4$ of being a normal year and $1 / 4$ of beingen year. chosen
- (a) A fair die is rolled. Find the expected value of the roll.
(b) Four fair dice are rolled. Find the expected total of the rolls. - A fair die is rolled some number of times. You can choose whether to stop after 1,2 , or. 3 rolls, and your decision can be based on the values that have appeared so far. You receive the value shown on the last roll of the die, in dollars. What is your optimal strategy (to maximize your expected winnings)? Find the expected winnings for this
Hint: Start by considering a simpler version of this problem, where there are at most 2 rolls. For what values of the first roll should you continue for a seoond roll? rolls. For what values of the first roll should you continue for a seoond roll?
Find the mean and variance of a Discrete Uniform r.v. on $1,2, \ldots, 7$
Hint: See the math appendix for some useful facts about sums.
3 of of 6. Two teams are going to play a best-of-7 match (the match will end as soon as either team. Assume that each team is equally likely to win each game, and that the games played are independent. Find the mean and variance of the number of games played. - A certain small town, whose population consists of 100 families, has 30 families with 1 . of these children is 1 if the child is the firstborn, 2 if the child is the secondborn, and 3 if the child is the thirdborn.
(a) A random family is chosen (with equal probabilities), and then a random child within that family is chosen (with equal probabilities). Find the PMF, mean, and variance of the child’s birth rank.
(a) Walk away with $\$ 16,000 .$ (b) Apply his lifeline to the $10 t h$ question, and then answer it. If he gets it wrong. he will leave with $\$ 1,000$. If he gets it right, he moves on to the 11 th question. He then
leaves with $\$ 32,000$ if he gets the 11 th question wrong, and $\$ 64,000$ if he gets the 11 th question right. (c) Hanestion right.
(c) Same as the previous option, except not using his lifeline on the $10 t h$ question, and instead applying it to the 11 th question (if he gets the 10 th question right). Whe applying it to the 11 th question (if he gets the 10 th question right).
Find the expected value of each of these options. Which option has the highest expected value? Which option has the lowest variance?
Consider the St. Petersburg paradox (Example 4.3.14), except that you reoeive Sn rather than $\$ 2^{n}$ if the game lasts for $n$ rounds. What is the fair value of this game? What if the payoff is $\$ n^{2} ?$
the payof is $\$ n^{2}$. - Martin has just heard about the following exciting gambling strategy: bet $\$ 1$ that a toss, now betting $\$ 2$ on Heads. If it does, stop. Otherwise, double the bet for the next toss to $\$ 4$. Continue in this way, doubling the bet each time and then stopping right after winning a bet. Assume that each individual bet is fair, i.e., has an expected net winter winning a bet. Assume that winnings of 0 . The idea is that
$1+2+2^{2}+2^{3}+\cdots+2^{n}=2^{n+1}-1$
so the gambler will be $\$ 1$ ahead after
profit. Martin decides to try out this strategy. However, he only has $\$ 31$, so he may end up
walking away bankrupt rather than continuing to double his bet. On average, how much money will Martin win?
统计代考
4.12 练习
标有(S)的练习在http://stat110.net上有详细的解答。
期望和差异
- 第 2 章中的变形虫 Bobo 目前独自生活在池塘中。一分钟后,波波要么死,要么分裂成两个变形虫,要么保持不变,概率相同。求一个后池塘中变形虫数量的期望值和方差
- 在公历中,每年有 365 天(平年)或 366 天(闰年)。一年是随机选择的,正常年份的概率为 $3 / 4$,而正常年份的概率为 $1 / 4$。选择
- (a) 掷出一个公平的骰子。找到滚动的期望值。
(b) 掷出四个公平的骰子。找到预期的总卷数。 - 一个公平的骰子被掷了若干次。您可以选择是否在 1,2 之后停止,或者。 3 卷,您的决定可以基于迄今为止出现的值。您会收到最后一卷骰子上显示的价值,以美元为单位。您的最佳策略是什么(最大化您的预期收益)?找到预期的奖金
提示:首先考虑这个问题的一个更简单的版本,最多有 2 个卷。对于第一个滚动的值,您应该继续进行第二个滚动?劳斯莱斯。对于第一个滚动的值,您应该继续进行第二个滚动?
求离散均匀 r.v. 的均值和方差。在 $1,2, \ldots, 7$
提示:有关求和的一些有用事实,请参见数学附录。
6 场比赛中的 3 场。两支球队将进行七局两胜的比赛(比赛将在任何一支球队结束时立即结束。假设每支球队赢得每场比赛的可能性相同,并且所进行的比赛是独立的。找到游戏次数的均值和方差。 - 某小镇,人口100户,有30户,1户。如果孩子是第一个孩子,则这些孩子中的 1 个,如果孩子是第二个孩子,则为 2 个,如果孩子是第三个孩子,则为 3 个。
(a) 选择一个随机家庭(概率相等),然后选择该家庭中的一个随机孩子(概率相等)。找出孩子出生等级的 PMF、均值和方差。
(a) 拿着$\$ 16,000 .$ 离开。 (b) 将他的生命线应用到$10 t h$ 的问题上,然后回答它。如果他弄错了。他将带着$\$ 1,000$离开。如果他做对了,他会继续第 11 个问题。他当时
如果他第 11 题答错了,则剩下 $\$ 32,000$,如果第 11 题答对,则剩下 $\$ 64,000$。 (c) Hanestion 权利。
(c) 与前一个选项相同,除了在第 10 美元问题上不使用他的生命线,而是将其应用于第 11 道问题(如果他答对了第 10 道问题)。将其应用于第 11 个问题(如果他答对了第 10 个问题)。
找出每个选项的期望值。哪个选项的期望值最高?哪个选项的方差最小?
考虑圣彼得堡悖论(示例 4.3.14),除了如果游戏持续 $n$ 轮,你得到的是 Sn 而不是 $\$ 2^{n}$。这个游戏的公允价值是多少?如果回报是 $\$ n^{2} 怎么办?$
报酬是 $\$ n^{2}$。 - Martin 刚刚听说了以下令人兴奋的赌博策略:下注 $\$ 1$ 那是一掷千金,现在下注 $\$ 2$ 在正面。如果是这样,请停止。否则,将下一次投掷的赌注加倍至 $\$ 4$。以这种方式继续,每次加倍下注,然后在赢得下注后立即停止。假设每个单独的赌注都是公平的,即有一个预期的净冬季赢得赌注。假设奖金为 0 。这个想法是
$1+2+2^{2}+2^{3}+\cdots+2^{n}=2^{n+1}-1$
所以赌徒会领先$\$ 1$
利润。马丁决定尝试这种策略。然而,他只有$\$ 31$,所以他可能最终
破产离开而不是继续加倍赌注。平均而言,马丁会赢多少钱?
。
R语言代写

统计代写|SAMPLE SPACES AND PEBBLE WORLD stat 代写 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。