数学代写| Decidability 离散代考
离散数学在计算领域有广泛的应用,例如密码学、编码理论、 形式方法, 语言理论, 可计算性, 人工智能, 理论 数据库和软件的可靠性。 离散数学的重点是理论和应用,而不是为了数学本身而研究数学。 一切算法的基础都是离散数学一切加密的理论基础都是离散数学
编程时候很多奇怪的小技巧(特别是所有和位计算相关的东西)核心也是离散数学
其他相关科目课程代写:组合学Combinatorics集合论Set Theory概率论Probability组合生物学Combinatorial Biology组合化学Combinatorial Chemistry组合数据分析Combinatorial Data Analysis
my-assignmentexpert愿做同学们坚强的后盾,助同学们顺利完成学业,同学们如果在学业上遇到任何问题,请联系my-assignmentexpert™,我们随时为您服务!
离散数学代写
Mathematics is decidable if the truth or falisty of any mathematical proposition may be determined by an algorithm.
Church and Turing independently showed this to be impossible in 1936. Church developed the lambda calculus in the 1930 s as a tool to study computability, ${ }^{3}$ and he showed that anything that is computable is computable by the lambda calculus. Turing showed that decidability was related to the halting problem for Turing machines, and that therefore if first-order logic were decidable then the halting problem for Turing machines could be solved. However, he had already proved that there was no general algorithm to determine whether a given Turing machine halts or not. Therefore, first-order logic is undecidable.
The question as to whether a given Turing machine halts or not can be formulated as a first-order statement. If a general decision procedure exists for first-order logic, then the statement of whether a given Turing machine halts or not is within the scope of the decision algorithm. However, Turing had already proved that the halting problem for Turing machines is not computable: i.e. it is not possible algorithmically to decide whether or not any given Turing machine will halt or not. Therefore, since there is no general algorithm that can decide whether any given Turing machine halts, there is no general decision procedure for first-order logic. The only way to determine whether a statement is true or false is to try to solve it. However, if one tries but does not succeed this does not prove that an answer does not exist.
There are first-order theories that are decidable. However, first-order logic that includes Peano’s axioms of arithmetic (or any formal system that includes addition and multiplication) cannot be decided by an algorithm. That is, there is no algorithm to determine whether an arbitrary mathematical proposition is true or false. Propositional logic is decidable as there is a procedure (e.g. using a truth table) to determine whether an arbitrary formula is valid ${ }^{4}$ in the calculus.
Gödel (Fig. 13.2) proved that first-order predicate calculus is complete. i.e. all truths in the predicate calculus can be proved in the language of the calculus.
Definition 13.2 (Completeness)
A formal system is complete if all the truths in the system can be derived from the axioms and rules of inference.
Gödel’s first incompleteness theorem showed that first-order arithmetic is incomplete; i.e. there are truths in first-order arithmetic that cannot be proved in the language of the axiomatization of first-order arithmetic. Gödel’s second incompleteness theorem showed that that any formal system extending basic arithmetic cannot prove its own consistency within the formal system.
Definition $13.3$
(Consistency) A formal system is consistent if there is no formula A such that A and $\neg \mathrm{A}$ are provable in the system (i.e. there are no contradictions in the system).

图论代考
Mathematics is decidable if the truth or falisty of any mathematical proposition may be determined by an algorithm.
Church and Turing independently showed this to be impossible in 1936. Church developed the lambda calculus in the 1930 s as a tool to study computability, ${ }^{3}$ and he showed that anything that is computable is computable by the lambda calculus. Turing showed that decidability was related to the halting problem for Turing machines, and that therefore if first-order logic were decidable then the halting problem for Turing machines could be solved. However, he had already proved that there was no general algorithm to determine whether a given Turing machine halts or not. Therefore, first-order logic is undecidable.
The question as to whether a given Turing machine halts or not can be formulated as a first-order statement. If a general decision procedure exists for first-order logic, then the statement of whether a given Turing machine halts or not is within the scope of the decision algorithm. However, Turing had already proved that the halting problem for Turing machines is not computable: i.e. it is not possible algorithmically to decide whether or not any given Turing machine will halt or not. Therefore, since there is no general algorithm that can decide whether any given Turing machine halts, there is no general decision procedure for first-order logic. The only way to determine whether a statement is true or false is to try to solve it. However, if one tries but does not succeed this does not prove that an answer does not exist.
There are first-order theories that are decidable. However, first-order logic that includes Peano’s axioms of arithmetic (or any formal system that includes addition and multiplication) cannot be decided by an algorithm. That is, there is no algorithm to determine whether an arbitrary mathematical proposition is true or false. Propositional logic is decidable as there is a procedure (e.g. using a truth table) to determine whether an arbitrary formula is valid ${ }^{4}$ in the calculus.
Gödel (Fig. 13.2) proved that first-order predicate calculus is complete. i.e. all truths in the predicate calculus can be proved in the language of the calculus.
Definition 13.2 (Completeness)
A formal system is complete if all the truths in the system can be derived from the axioms and rules of inference.
Gödel’s first incompleteness theorem showed that first-order arithmetic is incomplete; i.e. there are truths in first-order arithmetic that cannot be proved in the language of the axiomatization of first-order arithmetic. Gödel’s second incompleteness theorem showed that that any formal system extending basic arithmetic cannot prove its own consistency within the formal system.
Definition $13.3$
(Consistency) A formal system is consistent if there is no formula A such that A and $\neg \mathrm{A}$ are provable in the system (i.e. there are no contradictions in the system).

数学代写| DISCRETE MATHEMATICS代考 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。
抽象代数代考
抽象代数就是一门概念繁杂的学科,我们最重要的一点我想并不是掌握多少例子。即便是数学工作者也不会刻意记住Jacobson环、正则环这类东西,重要的是你要知道这门学科的基本工具和基本手法,对概念理解了没有,而这一点不需要用例子来验证,只需要看看你的理解和后续概念是否相容即可。
矩阵论代考matrix theory
数学,矩阵理论是一门研究矩阵在数学上的应用的科目。矩阵理论本来是线性代数的一个小分支,但其后由于陆续在图论、代数、组合数学和统计上得到应用,渐渐发展成为一门独立的学科。
密码学代考
密码学是研究编制密码和破译密码的技术科学。 研究密码变化的客观规律,应用于编制密码以保守通信秘密的,称为编码学;应用于破译密码以获取通信情报的,称为破译学,总称密码学。 电报最早是由美国的摩尔斯在1844年发明的,故也被叫做摩尔斯电码。
- Cryptosystem
- A system that describes how to encrypt or decrypt messages
- Plaintext
- Message in its original form
- Ciphertext
- Message in its encrypted form
- Cryptographer
- Invents encryption algorithms
- Cryptanalyst
- Breaks encryption algorithms or implementations
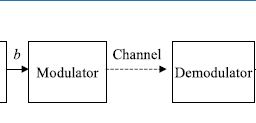
编码理论代写
编码理论(英语:Coding theory)是研究编码的性质以及它们在具体应用中的性能的理论。编码用于数据压缩、加密、纠错,最近也用于网络编码中。不同学科(如信息论、电机工程学、数学、语言学以及计算机科学)都研究编码是为了设计出高效、可靠的数据传输方法。这通常需要去除冗余并校正(或检测)数据传输中的错误。
编码共分四类:[1]
数据压缩和前向错误更正可以一起考虑。