数学代考| Propositional Logic 离散数学代写代写 - 代考代写：100%准时可靠您的作业代写专家

Propositional logic is the study of propositions where a proposition is a statement that is either true or false. There are many examples of propositions such as ‘ $1+1=2$ ‘ which is a true proposition, and the statement that ‘Today is Wednesday” which is true if today is Wednesday and false otherwise. The statement $x>0$ is not a proposition as it contains a variable $x$, and it is only meaningful to consider its truth or falsity only when a value is assigned to $x$. Once the variable $x$ is assigned a value, it becomes a proposition. The statement “This sentence is false” is not a proposition as it contains a self-reference that contradicts itself. Clearly, if the statement is true, it is false and if it is false, it is true.

A propositional variable may be used to stand for a proposition (e.g. let the variable $P$ stand for the proposition ‘ $2+2=4$ ‘ which is a true proposition). A propositional variable takes the value true or false. The negation of a proposition $\mathrm{P}($ denoted $\neg P)$ is the proposition that is true if and only if $P$ is false, and is false if and only if $P$ is true.

A well-formed formula (wff) in propositional logic is a syntactically correct formula created according to the syntactic rules of the underlying calculus. A well-formed formula is built up from variables, constants, terms and logical connectives such as conjunction (and), disjunction (or), implication (if.. then..), equivalence (if and only if) and negation. A distinguished subset of these well-formed formulae are the axioms of the calculus, and there are rules of inference that allow the truth of new formulae to be derived from the axioms
A formula in propositional calculus may contain several propositional variables, and the truth or falsity of the individual variables needs to be known prior to determine the truth or falsity of the logical formula.

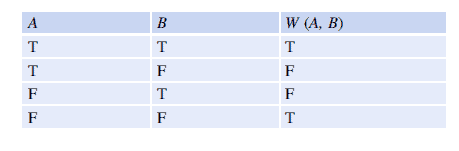
Each propositional variable has two possible values and a formula with $n$ propositional variables has $2^{n}$ values associated with the $n$-propositional variables. The set of values associated with the $n$ variables may be used to derive a truth table with $2^{n}$ rows and $n+1$ columns. Each row gives each of the $2^{n}$ truth-values that the $n$ variables may take, and column $n+1$ gives the result of the logical expression for that set of values of the propositional variables. For example, the propositional formula $W$ defined in the truth table above has two propositional variables $A$ and $B$, with $2^{2}=4$ rows for each of the values that the two propositional variables may
take. There are $2+1=3$ columns with $W$ defined in the third column (Table 15.1). and to build up well-formed formulae. This includes the conjunction of two propositions $(A \wedge B)$; the disjunction of two propositions $(A \vee B)$; and the implication of two propositions $(A \rightarrow B)$. These connectives allow compound propositions to be formed, and the truth of the compound propositions is determined from the truth-values of its constituent propositions and the rules associated with the logical connective. The meaning of the logical connectives is given by truth tables. ${ }^{1}$
${ }^{1}$ Basic truth tables were first used by Frege, and developed further by Post and Wittgenstein.

命题逻辑是对命题的研究，其中命题是对或错的陈述。命题的例子有很多，例如“$1+1=2$”是一个真命题，而“今天是星期三”的陈述如果今天是星期三则为真，否则为假。陈述 $x>0$ 是不是命题，因为它包含了一个变量$x$，只有给$x$赋值时才考虑它的真假才有意义。一旦给变量$x$赋值，它就变成了一个命题。 “这句话是假的”这句话不是一个命题，因为它包含一个自相矛盾的自我指涉。显然，如果该陈述为真，则为假，如果为假，则为真。

命题变量可用于代表命题（例如，让变量 $P$ 代表命题“$2+2=4$”，这是一个真命题）。命题变量取值真或假。命题 $\mathrm{P}($ 表示为 $\neg P)$ 的否定是当且仅当 $P$ 为假时为真的命题，当且仅当 $P$ 为真时为假。

命题逻辑中的格式良好的公式 (wff) 是根据基础演算的句法规则创建的句法正确的公式。一个格式良好的公式是由变量、常数、术语和逻辑连接词组成的，例如合取（and）、析取（or）、蕴涵（if.. then..）、等价（if and only if）和否定。这些格式良好的公式的一个显着子集是微积分的公理，并且有推理规则允许从公理中推导出新公式的真实性
命题演算中的一个公式可能包含多个命题变量，在确定逻辑公式的真假之前，需要知道各个变量的真假。

每个命题变量都有两个可能的值，一个具有 $n$ 个命题变量的公式具有与 $n$-命题变量相关联的 $2^{n}$ 个值。与 $n$ 变量相关的一组值可用于导出具有 $2^{n}$ 行和 $n+1$ 列的真值表。每行给出$n$ 变量可能采用的$2^{n}$ 个真值，$n+1$ 列给出命题变量的该组值的逻辑表达式的结果。例如，上面真值表中定义的命题公式 $W$ 有两个命题变量 $A$ 和 $B$，对于这两个命题变量可能的值，每一个都有 $2^{2}=4$ 行
拿。有 $2+1=3$ 列，在第三列中定义了 $W$（表 15.1）。并建立格式良好的公式。这包括两个命题 $(A \wedge B)$ 的合取；两个命题 $(A \vee B)$ 的析取；以及两个命题 $(A \rightarrow B)$ 的蕴涵。这些连接词可以形成复合命题，复合命题的真值由其组成命题的真值和与逻辑连接词相关的规则确定。逻辑连接词的含义由真值表给出。 ${ }^{1}$
${ }^{1}$ 基本真值表由弗雷格首先使用，并由波斯特和维特根斯坦进一步发展。

图论代考

本章简要介绍了逻辑，它涉及推理和确定论证的有效性。它允许根据逻辑规则从前提推导出结论，并且只要前提为真，逻辑论证就可以确定结论的真实性。

逻辑起源于对真理的本质感兴趣的希腊人。苏格拉底以破坏对手的立场而闻名（这意味着他在辩论中没有赢得任何朋友），而苏格拉底的调查由问答组成，其中对手将被引导得出与他原来的立场不符的结论。他的做法表明他的对手的立场是不连贯的和站不住脚的。

亚里士多德在逻辑方面做了重要的工作，他开发了一套逻辑系统，即三段论逻辑，一直使用到 19 世纪。三段论逻辑是一种“术语逻辑”，字母用来代表单个术语。三段论由两个前提和一个结论组成，其中结论是对两个前提的有效演绎。斯多葛派发展了一种早期形式的命题逻辑，其中可断言（命题）具有真值，它们要么是真要么是假。
George Boole 在 1800 年代中期发展了他的符号逻辑，它后来形成了数字计算的基础。布尔认为，逻辑应该被视为数学的一个独立分支，而不是哲学的一部分。他认为存在数学定律来表达人类思维中的推理操作，并且他展示了亚里士多德的三段论逻辑如何可以简化为一组代数方程。

Gottlob Frege 对逻辑和数学基础做出了重要贡献。他试图证明数学（或至少算术）的所有基本真理都可以从一组有限的逻辑公理中推导出来（这种方法被称为逻辑主义）。他发明了谓词逻辑以及全称和存在量词，谓词逻辑是亚里士多德三段论逻辑的重大进步。
参考文献
239

Ackrill JL (1994) 哲学家亚里士多德。克拉伦登出版社牛津
Boole G (1848) 逻辑演算。 Camb Dublin Math J. III:183-98
Boole G (1854) 对思想规律的调查。多佛出版物。 1854 年首次出版
麦克海尔 D (1985) 布尔。科克大学出版社
O’ Regan G (2013) 计算巨人。施普林格
Shannon C (1937) 继电器和开关电路的符号分析。硕士论文。麻省理工学院

数学代写| DISCRETE MATHEMATICS代考请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。

Cryptosystem
A system that describes how to encrypt or decrypt messages
Plaintext
Message in its original form
Ciphertext
Message in its encrypted form
Cryptographer
Invents encryption algorithms
Cryptanalyst
Breaks encryption algorithms or implementations

编码理论代写

编码理论（英语：Coding theory）是研究编码的性质以及它们在具体应用中的性能的理论。编码用于数据压缩、加密、纠错，最近也用于网络编码中。不同学科（如信息论、电机工程学、数学、语言学以及计算机科学）都研究编码是为了设计出高效、可靠的数据传输方法。这通常需要去除冗余并校正（或检测）数据传输中的错误。

编码共分四类：^[1]