博弈论代写代考| Sequential Games: The Theory of Moves 数学代写
博弈论代考
In this section, we engage in a little sensitivity analysis with ordinal games. Our starting point is that while the chicken dilemma modeled the essential dynamics of the Cuban Missile Crisis, it is not necessarily a good model to use for predictive purposes. Indeed, if each player tries for the Nash equilibrium that most benefits him or her, the prediction is the disastrous ( 1,1 ) outcome. As we know, that did not happen in 1962. As noted before, in Brams (1985a and 1985b), Brams considered the model obtained by allowing communication between the players, and he considered the sequential nature of the game when the players do not move simultaneously. Furthermore, we noted earlier that in the chicken dilemma model of the US federal government shutdown, while the $(1,1)$ outcome was indeed obtained, it did not last because players were
8.3 Sequential Games: The Theory of Moves
389
allowed to change their strategies. We suggested that a model that allows changes of strategies in a sequential game might be more realistic. Also, if players are allowed to switch strategies, that implies there is some starting point from which initial changes might be made.
When one modifies $2 \times 2$ ordinal game models to incorporate sequential moves, a starting position, and the ability to switch strategies, one arrives at the theory of moves, a technique first proposed in Brams (1994) and slightly modified in Taylor and Pacelli (2008). We use the version in Taylor and Pacelli $(2008)$ here.
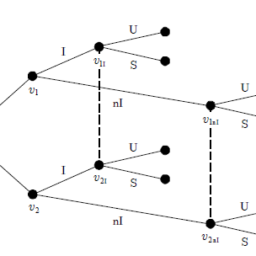
The idea is that each $2 \times 2$ ordinal payoff matrix can be used to create eight different sequential games, four when the row player has the first option to switch strategies (depending on which of the four outcomes is the initial position) and four when the column player has the first move option. Each such sequential game is modeled in extensive form, and, assuming the tree diagram is finite, one can analyze it via reverse induction to see what would actually happen in each of the eight cases. Note that the assumption that it can be analyzed by reverse induction implies we do not relax the intelligence assumption of game theory – we cont and agree on all the preference rankings in the payoff matrix. Here are the rules for creating the tree diagram. We give the rules for when the row player moves first. If the column player moves first, the rules are similar:
ALGORITHM $8.9$ Suppose you are given a $2 \times 2$ ordinal payoff matrix, and a sequential game is to be played with the row player moving first. The steps taken to set up the tree diagram for the Theory of Moves are as follows: .
- Each player makes a simultaneous and independent choice of a strategy. The resulting outcome on the payoff matrix is the starting position.
- The row player makes a choice either to switch to the other strategy or to stay with her Current strategy. In each case, draw a branch of the tree, and label it with the resulting outcome. For any branch that is not a terminal node, go to step 3. If every node is terminal, then stop-the tree is complete.
- The column player then has a choice to either switch to the other strategy or to stay with his current strategy. For any branch that is not a terminal node, go to step $2 .$ If every node is terminal, then stop-the tree is complete.
The conditions for a terminal node are as follows: - If it is the row player’s turn to move, and the current outcome is $(4,-)$, except on the row player’s first move.
- If it is the column player’s turn to move, and the current outcome is $(-, 4)$.
- If either player elects to stay, except a stay on the row player’s first move.
The rules ensure that both players have at least one chance to switch strategies if they want, which is why an initial move by the row player (or whoever goes first) never ends the game. Furthermore, if one player places the other in an outcome, then presumably, he or she is happy with that outcome, but if it happens to be the first choice of the other player (the 4 preference), then – since both players are satisfied – there should be no further switches. The same holds if either player elects to stay with their current choice, as long as both players have had a chance to switch. Once the game tree is set up, the outcome of the game is determined using backwards induction.
390
Sensitivity Analysis, Ordinal Games, and n-Person Games
When a game is played under simultaneous moves, the “stable points” are called Nash equilibria. For the theory of moves, we have the following definition:
DEFINITION 8.10 If an outcome of the game has the property that whenever it is the starting position, it is also the final position when the row player moves first, it is said to be a nonmyopic equilibrium when row goes first. Similarly, if it has the property that whenever it is the starting position, it is also the funal position when the column player moves first, it is said to be a non-myopic equilibrium when column goes first. If an outcome
The name stems from the idea that by analyzing the tree diagram for the sequential moves, one is looking ahead, or being “far-sighted”by anticipating what the other would do in each outcome. we denote by $C$ the cooperation strategy (swerve out of the way) and by $D$ the defection strategy (drive straight on).

在本节中,我们对序数博弈进行了一些敏感性分析。我们的出发点是,虽然鸡困境模拟了古巴导弹危机的基本动态,但它不一定是用于预测目的的好模型。事实上,如果每个参与者都尝试对他或她最有利的纳什均衡,那么预测就是灾难性的 (1,1) 结果。正如我们所知,这在 1962 年并没有发生。如前所述,在 Brams(1985a 和 1985b)中,Brams 考虑了通过允许玩家之间进行通信而获得的模型,并且他考虑了玩家不移动时游戏的顺序性质同时地。此外,我们之前注意到,在美国联邦政府关门的鸡两难模型中,虽然确实得到了 $(1,1)$ 的结果,但它并没有持续下去,因为玩家被
8.3 顺序博弈:移动理论
389
允许改变他们的策略。我们建议允许在顺序博弈中改变策略的模型可能更现实。此外,如果允许玩家切换策略,这意味着可能会从某个起点进行初始更改。
当一个人修改 $2\times 2$ 序数博弈模型以结合顺序移动、起始位置和切换策略的能力时,就会得出移动理论,这是一种在 Brams (1994) 中首次提出并在 Taylor 和帕切利 (2008)。我们在这里使用 Taylor 和 Pacelli $(2008)$ 中的版本。
这个想法是每个 $2\times 2$ 的序数收益矩阵可以用来创建八种不同的顺序游戏,四个当行玩家有第一个切换策略的选项时(取决于四个结果中的哪个是初始位置)和四个当纵队玩家有先手选项时。每个这样的顺序博弈都以扩展的形式建模,假设树图是有限的,可以通过反向归纳分析它,看看在八种情况下实际会发生什么。请注意,可以通过反向归纳分析的假设意味着我们不会放松博弈论的智力假设——我们继续并同意支付矩阵中的所有偏好排名。以下是创建树形图的规则。我们给出了排玩家首先移动的规则。如果纵队玩家先走,规则类似:
算法 $8.9$ 假设给定一个 $2\times 2$ 的序数收益矩阵,并且要进行一个顺序游戏,其中行玩家首先移动。为移动理论设置树形图所采取的步骤如下:
- 每个玩家同时独立地选择策略。收益矩阵的结果是起始位置。
- 排玩家选择切换到其他策略或保持当前策略。在每种情况下,绘制树的一个分支,并用结果标记它。对于不是终端节点的任何分支,转到步骤 3。如果每个节点都是终端,则停止树完成。
- 纵队玩家可以选择切换到其他策略或保持当前策略。对于不是终端节点的任何分支,转到步骤 $2。$ 如果每个节点都是终端,则停止树完成。
终端节点的条件如下: - 如果轮到排位玩家移动,并且当前结果是$(4,-)$,排位玩家的第一个动作除外。
2.如果轮到列玩家移动,当前的结果是$(-, 4)$。 - 如果任一玩家选择留下,除了停留在排玩家的第一步。
规则确保双方玩家至少有一次机会根据需要切换策略,这就是为什么排玩家(或先走的人)的初始移动永远不会结束游戏的原因。此外,如果一个玩家将另一个放在一个结果中,那么他或她可能对该结果感到满意,但如果它恰好是另一个玩家的首选(4 偏好),那么 – 因为两个玩家都满意- 不应该有更多的开关。如果任何一个玩家选择保留他们当前的选择,只要两个玩家都有机会切换,这同样适用。一旦建立了博弈树,博弈的结果就使用反向归纳来确定。
390
敏感性分析、序数博弈和 n 人博弈
当游戏同时进行时,“稳定点”称为纳什均衡。对于移动理论,我们有以下定义:
定义 8.10 如果游戏的结果具有这样的性质,即无论何时它是起始位置,当排玩家首先移动时它也是最终位置,则当排先行时,它被称为非近视平衡。类似地,如果它具有无论何时是起始位置,也是纵队玩家先行时的最终位置的性质,则称纵队先行时非近视平衡。如果一个结果

其他相关科目课程代写:组合学Combinatorics集合论Set Theory概率论Probability组合生物学Combinatorial Biology组合化学Combinatorial Chemistry组合数据分析Combinatorial Data Analysis
my-assignmentexpert愿做同学们坚强的后盾,助同学们顺利完成学业,同学们如果在学业上遇到任何问题,请联系my-assignmentexpert™,我们随时为您服务!
博弈论,又称为对策论(Game Theory)、赛局理论等,既是现代数学的一个新分支,也是运筹学的一个重要学科。 博弈论主要研究公式化了的激励结构间的相互作用,是研究具有斗争或竞争性质现象的数学理论和方法。 博弈论考虑游戏中的个体的预测行为和实际行为,并研究它们的优化策略。
计量经济学代考
计量经济学是以一定的经济理论和统计资料为基础,运用数学、统计学方法与电脑技术,以建立经济计量模型为主要手段,定量分析研究具有随机性特性的经济变量关系的一门经济学学科。 主要内容包括理论计量经济学和应用经济计量学。 理论经济计量学主要研究如何运用、改造和发展数理统计的方法,使之成为经济关系测定的特殊方法。
相对论代考
相对论(英語:Theory of relativity)是关于时空和引力的理论,主要由愛因斯坦创立,依其研究对象的不同可分为狭义相对论和广义相对论。 相对论和量子力学的提出给物理学带来了革命性的变化,它们共同奠定了现代物理学的基础。
编码理论代写
编码理论(英语:Coding theory)是研究编码的性质以及它们在具体应用中的性能的理论。编码用于数据压缩、加密、纠错,最近也用于网络编码中。不同学科(如信息论、电机工程学、数学、语言学以及计算机科学)都研究编码是为了设计出高效、可靠的数据传输方法。这通常需要去除冗余并校正(或检测)数据传输中的错误。
编码共分四类:[1]
数据压缩和前向错误更正可以一起考虑。
复分析代考
学习易分析也已经很冬年了,七七八人的也续了圧少的书籍和论文。略作总结工作,方便后来人学 Đ参考。
复分析是一门历史悠久的学科,主要是研究解析函数,亚纯函数在复球面的性质。下面一昭这 些基本内容。
(1) 提到复变函数 ,首先需要了解复数的基本性左和四则运算规则。怎么样计算复数的平方根, 极坐标与 $x y$ 坐标的转换,复数的模之类的。这些在高中的时候囸本上都会学过。
(2) 复变函数自然是在复平面上来研究问题,此时数学分析里面的求导数之尖的运算就会很自然的 引入到复平面里面,从而引出解析函数的定义。那/研究解析函数的性贡就是关楗所在。最关键的 地方就是所谓的Cauchy一Riemann公式,这个是判断一个函数是否是解析函数的关键所在。
(3) 明白解析函数的定义以及性质之后,就会把数学分析里面的曲线积分 $a$ 的概念引入复分析中, 定义几乎是一致的。在引入了闭曲线和曲线积分之后,就会有出现复分析中的重要的定理: Cauchy 积分公式。 这个是易分析的第一个重要定理。