数学代写|实分析代写real analysis代考|Integrable Functions and L1 (E)

如果你也在 怎样代写实分析real analysis这个学科遇到相关的难题，请随时右上角联系我们的24/7代写客服。实分析real analysis是数学分析的一个分支，研究实数、实数序列和实数函数的行为。 实分析研究的实值序列和函数的一些特殊性质包括收敛性、极限、连续性、平稳性、可微分性和可整定性。实分析有别于复分析，后者涉及复数及其函数的研究。

实分析real analysis是数学中的一个经典分支，它的发展是为了使数和函数的研究正规化，并研究重要的概念，如极限和连续性。这些概念是微积分及其应用的基础。实物分析已经成为许多应用领域中不可或缺的工具。

实分析real analysis的基础知识：序列和数列的收敛性、连续性、可分性、黎曼积分、函数的序列和数列、均匀性以及极限操作的互换。

my-assignmentexpert™ 实分析real analysis作业代写，免费提交作业要求， 满意后付款，成绩80\%以下全额退款，安全省心无顾虑。专业硕 博写手团队，所有订单可靠准时，保证 100% 原创。my-assignmentexpert™， 最高质量的实分析real analysis作业代写，服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面，考虑到同学们的经济条件，在保障代写质量的前提下，我们为客户提供最合理的价格。 由于统计Statistics作业种类很多，同时其中的大部分作业在字数上都没有具体要求，因此复分析complex analysis作业代写的价格不固定。通常在经济学专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。

想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。

my-assignmentexpert™ 为您的留学生涯保驾护航 在数学mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学mathematics代写服务。我们的专家在实分析real analysis代写方面经验极为丰富，各种实分析real analysis相关的作业也就用不着 说。

我们提供的实分析real analysis及其相关学科的代写，服务范围广, 其中包括但不限于:

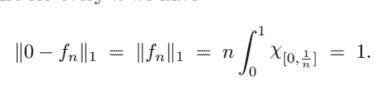
数学代写|实分析代写real analysis代考|The Lebesgue Space L1(E)

The Lebesgue space $L^{\infty}(E)$ introduced in Definition 3.3.3 consists of the essentially bounded functions on $E$. We similarly collect the integrable func-tions to form a space that we call $L^{1}(E)$. Technically, there are two versions of $L^{1}(E)$, one consisting of complex-valued functions and one consisting of extended real-valued functions (which must be finite a.e., since they are integrable). Both cases are important, and in practice it is usually clear from context whether we are working with extended real-valued functions or complex-valued functions. As usual, we combine the two possibilities into a single definition by letting $\overline{\mathbf{F}}$ denote either $[-\infty, \infty]$ or $\mathbb{C}$. Implicitly, the word scalar denotes a real number $c \in \mathbb{R}$ if $\overline{\mathbf{F}}=[-\infty, \infty]$, and a complex number $c \in \mathbb{C}$ if $\overline{\mathbf{F}}=\mathbb{C}$.

Definition 4.4.3 (The Lebesgue Space $L^{1}(E)$ ). If $E$ is a measurable subset of $\mathbb{R}^{d}$, then the Lebesgue space of integrable functions on $E$ is
$L^{1}(E)=\left{f: E \rightarrow \overline{\mathbf{F}}: f\right.$ is measurable and $\left.|f|_{1}=\int_{E}|f|<\infty\right}$
Suppose that $f$ and $g$ are integrable functions on $E$ and $a$ and $b$ are scalars. Regardless of whether we are considering extended real-valued or complexvalued functions, $|a f+b g|$ is an extended real-valued function. Therefore we can apply Theorem $4.2 .3$ and compute that
$$
\int_{E}|a f+b g| \leq \int_{E}(|a||f|+|b||g|)=|a| \int_{E}|f|+|b| \int_{E}|g|<\infty
$$
This shows that $a f+b g$ is integrable. Consequently $L^{1}(E)$ is closed under the operations of addition of functions and multiplication of a function by a scalar, so it is a vector space with respect to these operations.

数学代写|实分析代写REAL ANALYSIS代考|Convergence in L1-Norm

The distance between two functions $f$ and $g$ with respect to the $L^{1}$-norm is $|f-g|_{1}$. Once we have a notion of distance, we also have a corresponding notion of convergence, made precise in the following definition.

Definition 4.4.6 (Convergence in $L^{1}$-Norm). Let $E$ be a measurable subset of $\mathbb{R}^{d}$. A sequence of integrable functions $\left{f_{n}\right}_{n \in \mathbb{N}}$ on $E$ (either extended real-valued or complex-valued) is said to converge to $f$ in $L^{1}$-norm if
$$
\lim {n \rightarrow \infty}\left|f-f{n}\right|_{1}=\lim {n \rightarrow \infty} \int{E}\left|f-f_{n}\right|=0 .
$$
In this case we write $f_{n} \rightarrow f$ in $L^{1}$-norm.
The following examples compare $L^{1}$-norm convergence to pointwise a.e. convergence.
Example 4.4.7. The domain for this example is $E=[0,1]$.
(a) The Shrinking Boxes $f_{n}=\chi_{\left[0, \frac{1}{n}\right]}$ from Example 3.5.2 converge pointwise a.e. to the zero function, and they also converge to the zero function in $L^{1}$-norm, because
$$
\left|0-f_{n}\right|_{1}=\left|f_{n}\right|_{1}=\int_{0}^{1} \chi_{\left[0, \frac{1}{n}\right]}=\frac{1}{n} \rightarrow 0 .
$$
(b) The Shrinking Boxes $f_{n}=n \chi_{\left[0, \frac{1}{n}\right]}$ from Example 4.2.6 converge pointwise a.e. to the zero function, but they do not converge in $L^{1}$-norm to the zero function because for every $n$ we have
$$
\left|0-f_{n}\right|_{1}=\left|f_{n}\right|_{1}=n \int_{0}^{1} \chi_{\left[0, \frac{1}{n}\right]}=1
$$
Hence pointwise a.e. convergence does not imply $L^{1}$-norm convergence in general.

(c) Let $\left{f_{n}\right}_{n \in \mathbb{N}}$ be the sequence of Boxes Marching in Circles defined in Example 3.5.5. The values of $\left|f_{n}\right|_{1}$ for $n=1, \ldots, 10$ are
$$
1, \frac{1}{2}, \frac{1}{2}, \frac{1}{3}, \frac{1}{3}, \frac{1}{3}, \frac{1}{4}, \frac{1}{4}, \frac{1}{4}, \frac{1}{4} .
$$
Continuing this sequence, we see that the functions $f_{n}$ converge in $L^{1}$ norm to the zero function (slowly, to be sure, but they do converge). However, $f_{n}$ does not converge pointwise a.e., so convergence in $L^{1}$-norm does not imply pointwise a.e. convergence.

Although $L^{1}$-norm convergence does not imply pointwise a.e. convergence, we will use Tchebyshev’s Inequality to prove that $L^{1}$-norm convergence implies convergence in measure, and consequently there must exist a subsequence that converges pointwise a.e.

数学代写|实分析代写REAL ANALYSIS代考|Linearity of the Integral for Integrable Functions

By Theorem 4.2.3, $\int_{E}(f+g)=\int_{E} f+\int_{E} g$ for all nonnegative functions $f$ and $g$. We will enlarge the class of functions for which this conclusion holds, but we must impose some restrictions in order to exclude indeterminate forms. The following result achieves this by focusing on integrable functions.
Theorem 4.4.10 (Linearity of the Integral). Let $E \subseteq \mathbb{R}^{d}$ be a measurable set. If $f, g: E \rightarrow \overline{\mathbf{F}}$ are integrable functions and $a$ and $b$ are scalars, then
$$
\int_{E}(a f+b g)=a \int_{E} f+b \int_{E} g .
$$
Proof. Case 1: $\overline{\mathbf{F}}=[-\infty, \infty]$. Assume that $f, g: E \rightarrow[-\infty, \infty]$ are integrable functions on $E$. By equation (4.15), their sum $f+g$ is also integrable. Define the measurable sets
$$
\begin{array}{ll}
E_{1}={f \geq 0, g \geq 0}, & E_{4}={f<0, g \geq 0, f+g \geq 0}, \
E_{2}={f \geq 0, g<0, f+g \geq 0}, & E_{5}={f<0, g \geq 0, f+g<0} \
E_{3}={f \geq 0, g<0, f+g<0}, & E_{6}={f<0, g<0}
\end{array}
$$
Consider the integral of $f+g$ on the set $E_{2}$. Since $f+g$ and $-g$ are each nonnegative on $E_{2}$, we compute that
$\begin{array}{rlr}\int_{E_{2}}(f+g)-\int_{E_{2}} g & =\int_{E_{2}}(f+g)+\int_{E_{2}}(-g) \quad(\text { by Exercise } 4.3 .6(\mathrm{e})) \ & =\int_{E_{2}}(f+g)+(-g) \quad \text { (by Theorem 4.2.3) } \ & =\int_{E_{2}} f\end{array}$

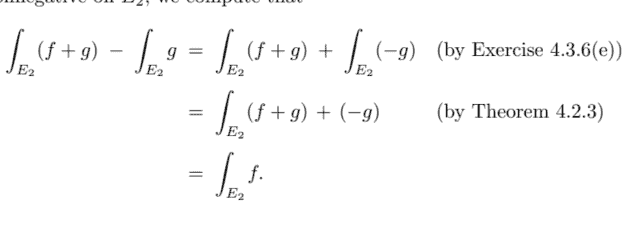
实分析代写

数学代写|实分析代写REAL ANALYSIS代考|THE LEBESGUE SPACE L1和

勒贝格空间大号∞(和)定义 3.3.3 中介绍的基本有界函数和. 我们类似地收集可积函数以形成我们称之为空间大号1(和). 从技术上讲，有两个版本大号1(和), 一种由复值函数组成，一种由扩展实值函数组成在H一世CH米你s吨b和F一世n一世吨和一种.和.,s一世nC和吨H和是一种r和一世n吨和Gr一种b一世和. 这两种情况都很重要，在实践中，通常可以从上下文中清楚我们是在使用扩展实值函数还是复值函数。像往常一样，我们通过让F¯表示任一[−∞,∞]或者C. 隐含地，标量这个词表示一个实数C∈R如果F¯=[−∞,∞], 和一个复数C∈C如果F¯=C.

定义 4.4.3吨H和大号和b和sG你和小号
$L^{1}(E)=\left{f: E \rightarrow \overline{\mathbf{F}}: f\right.$ is measurable and $\left.|f|_{1}=\int_{E}|f|<\infty\right}$
Suppose that $f$ and $g$ are integrable functions on $E$ and $a$ and $b$ are scalars. Regardless of whether we are considering extended real-valued or complexvalued functions, $|a f+b g|$ is an extended real-valued function. Therefore we can apply Theorem $4.2 .3$ and compute that
$$
\int_{E}|a f+b g| \leq \int_{E}(|a||f|+|b||g|)=|a| \int_{E}|f|+|b| \int_{E}|g|<\infty
$$和$在函数的加法和函数乘以标量的操作下是闭合的，因此它是关于这些操作的向量空间。

数学代写|实分析代写REAL ANALYSIS代考|CONVERGENCE IN L1-NORM

两个函数之间的距离F和G相对于该大号1-规范是|F−G|1. 一旦我们有了距离的概念，我们也有一个相应的收敛概念，在下面的定义中得到了精确的定义。

定义 4.4.6C这nv和rG和nC和一世n$大号1$−ñ这r米. 让和是可测量的子集Rd. 一系列可积函数\left{f_{n}\right}_{n \in \mathbb{N}}\left{f_{n}\right}_{n \in \mathbb{N}}在和 和一世吨H和r和X吨和nd和dr和一种一世−v一种一世你和d这rC这米p一世和X−v一种一世你和d据说收敛到F在大号1-norm if
$$
\int_{E}(a f+b g)=a \int_{E} f+b \int_{E} g .
$$
Proof. Case 1: $\overline{\mathbf{F}}=[-\infty, \infty]$. Assume that $f, g: E \rightarrow[-\infty, \infty]$ are integrable functions on $E$. By equation (4.15), their sum $f+g$ is also integrable. Define the measurable sets
$$
\begin{array}{ll}
E_{1}={f \geq 0, g \geq 0}, & E_{4}={f<0, g \geq 0, f+g \geq 0}, \
E_{2}={f \geq 0, g<0, f+g \geq 0}, & E_{5}={f<0, g \geq 0, f+g<0} \
E_{3}={f \geq 0, g<0, f+g<0}, & E_{6}={f<0, g<0}
\end{array}
$$
因此逐点 ae 收敛并不意味着大号1- 范数收敛一般。

C让\left{f_{n}\right}_{n \in \mathbb{N}}\left{f_{n}\right}_{n \in \mathbb{N}}是示例 3.5.5 中定义的圆中行进的框的序列。的价值观|Fn|1为了n=1,…,10是
1,12,12,13,13,13,14,14,14,14.
继续这个序列，我们看到函数Fn收敛于大号1零函数的范数s一世这在一世是,吨这b和s你r和,b你吨吨H和是d这C这nv和rG和. 然而，Fn不逐点收敛 ae，所以收敛于大号1-norm 并不意味着逐点 ae 收敛。

虽然大号1-范数收敛并不意味着逐点ae收敛，我们将使用切比雪夫不等式来证明大号1-范数收敛意味着度量收敛，因此必须存在一个逐点收敛的子序列 ae

数学代写|实分析代写REAL ANALYSIS代考|LINEARITY OF THE INTEGRAL FOR INTEGRABLE FUNCTIONS

根据定理 4.2.3，∫和(F+G)=∫和F+∫和G对于所有非负函数F和G. 我们将扩大这一结论所适用的函数类别，但我们必须施加一些限制以排除不定形式。以下结果通过关注可积函数实现了这一点。
定理 4.4.10大号一世n和一种r一世吨是这F吨H和一世n吨和Gr一种一世. 让和⊆Rd是一个可测量的集合。如果F,G:和→F¯是可积函数和一种和b是标量，那么
∫和(一种F+bG)=一种∫和F+b∫和G.
证明。情况1：F¯=[−∞,∞]. 假使，假设F,G:和→[−∞,∞]是可积函数和. 通过方程4.15, 他们的总和F+G也是可积的。定义可测量集
和1=F≥0,G≥0,和4=F<0,G≥0,F+G≥0, 和2=F≥0,G<0,F+G≥0,和5=F<0,G≥0,F+G<0 和3=F≥0,G<0,F+G<0,和6=F<0,G<0
考虑积分F+G在片场和2. 自从F+G和−G都是非负的和2, 我们计算
∫和2(F+G)−∫和2G=∫和2(F+G)+∫和2(−G)( 通过运动 4.3.6(和)) =∫和2(F+G)+(−G) （由定理 4.2.3）  =∫和2F

数学代写|实分析代写real analysis代考 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。

复分析

纠错码代写

离散数学代写

Partial Differential Equations代写可以参考一份偏微分方程midterm答案解析

时间序列分析代写