如果你也在 怎样代写抽象代数abstract algebra这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。抽象代数abstract algebra是代数的一组高级课题,涉及抽象代数结构而不是通常的数系。这些结构中最重要的是群、环和域。
抽象代数abstract algebra如果你在街上问别人这个问题,最可能的回答是。”一些与X、Y和Z有关的可怕的事情”。如果你足够幸运,碰到了一个数学家,那么你可能会得到这样的回答。”代数是对我们的组成直觉的抽象封装。我们对组合的直觉”。我们所说的构成,是指两个物体在一起形成一个新物体的概念,一起形成一个新的对象。例如,将两个数字相加,或将实值的单变量函数。我们将发现,看似简单的组合概念中隐藏着巨大的隐藏深度。代数渗透到我们所有的数学直觉中。事实上,概念是这个学科的基础。
my-assignmentexpert™ 抽象代数abstract algebra作业代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。my-assignmentexpert™, 最高质量的抽象代数abstract algebra作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于统计Statistics作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此抽象代数abstract algebra作业代写的价格不固定。通常在经济学专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
my-assignmentexpert™ 为您的留学生涯保驾护航 在数学mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的抽象代数abstract algebra代写服务。我们的专家在数学mathematics代写方面经验极为丰富,各种抽象代数abstract algebra相关的作业也就用不着 说。
我们提供的抽象代数abstract algebra及其相关学科的代写,服务范围广, 其中包括但不限于:
数学代写|抽象代数代写abstract algebra代考|algebraic properties
In studying groups we are interested in their algebraic properties, and not in the particular form in which they are presented. For example, if we construct the multiplication tables for two finite groups and find that they have the same patterns, although the elements might have different forms, then we would say that the groups have exactly the same algebraic properties.
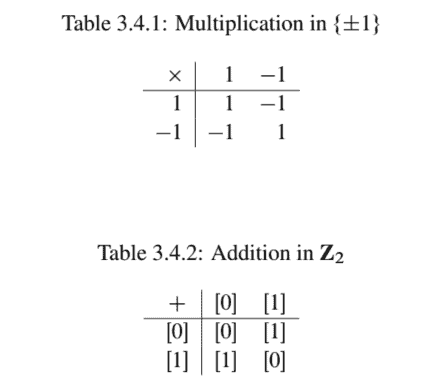
Consider the subgroup ${\pm 1}$ of $\mathbf{Q}^{\times}$and the group $\mathbf{Z}{2}$. If you write out the group tables for these groups you will find precisely the same pattern, as shown in Tables 3.4.1 and 3.4.2. Table 3.4.1: Multiplication in ${\pm 1}$ \begin{tabular}{r|rr} $\times$ & 1 & $-1$ \ \hline 1 & 1 & $-1$ \ $-1$ & $-1$ & 1 \end{tabular} Table 3.4.2: Addition in $\mathbf{Z}{2}$
\begin{tabular}{c|cc}
$+$ & {$[0]$} & {$[1]$} \
\hline$[0]$ & {$[0]$} & {$[1]$} \
{$[1]$} & {$[1]$} & {$[0]$}
\end{tabular}
Actually, if we have any group $G$ with two elements, say the identity element $e$ and one other element $a$, then there is only one possibility for the multiplication table for $G$. We have already observed that Propositions 3.1.7 and 3.1.8 imply that in each row and column of a group table, each element of the group must occur exactly once. Since $e$ is the identity element, $e \cdot e=e, e \cdot a=a$, and $a \cdot e=a$. Since $a$ cannot be repeated in the last row of the table, we must have $a \cdot a=e$.
数学代写|抽象代数代写abstract algebra代考|isomorphic
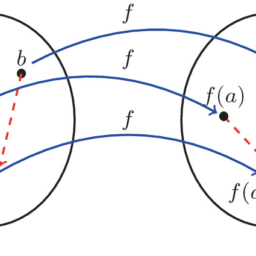
We need a formal definition to describe when two groups have the same algebraic properties. To begin with, there should be a one-to-one correspondence between the elements of the groups. This means in essence that elements of one group could be renamed to correspond exactly to the elements of the second group. Furthermore, products of corresponding elements should correspond. If $G_{1}$ is a group with operation $*$ and $G_{2}$ is a group with operation $\star$, then any function $\phi: G_{1} \rightarrow G_{2}$ that preserves products must have the property that $\phi(a * b)=\phi(a) \star \phi(b)$ for all $a, b \in G_{1}$. This expresses in a formula the fact that if we first multiply $a$ and $b$ to exactly the same answer as if we find the corresponding elements $\phi(a)$ and $\phi(b)$ in exactly the same answer as if we find the corresponding elements $\phi(a)$ and $\phi(b)$ in $G_{2}$ and then compute their product $\phi(a) \star \phi(b)$ in $G_{2}$. It is rather cumbersome to write the two operations, so in our definition we will omit them, since it should be clear from the context which operation is to be used. A one-to-one correspondence words isos meaning “equal” and morphe meaning “form.”
3.4.1 Definition. Let $G_{1}$ and $G_{2}$ be groups, and let $\phi: G_{1} \rightarrow G_{2}$ be a function. Then $\phi$ is said to be a group isomorphism if $\phi$ is one-to-one and onto and
$$
\phi(a b)=\phi(a) \phi(b)
$$
for all $a, b \in G_{1}$. In this case, $G_{1}$ is said to be isomorphic to $G_{2}$, and this is denoted by $G_{1} \cong G_{2}$.
抽象代数代写
数学代写|抽象代数代写ABSTRACT ALGEBRA代考|ALGEBRAIC PROPERTIES
在研究小组中,我们对它们的代数性质感兴趣,而不是对它们呈现的特定形式感兴趣。例如,如果我们为两个有限群构建乘法表并发现它们具有相同的模式,尽管元素可能具有不同的形式,那么我们可以说这些群具有完全相同的代数性质。
考虑子 ${\pm 1}$ of $\mathbf{Q}^{\times}$and the group $\mathbf{Z}{2}$. If you write out the group tables for these groups you will find precisely the same pattern, as shown in Tables 3.4.1 and 3.4.2. Table 3.4.1: Multiplication in ${\pm 1}$ \begin{tabular}{r|rr} $\times$ & 1 & $-1$ \ \hline 1 & 1 & $-1$ \ $-1$ & $-1$ & 1 \end{tabular} Table 3.4.2: Addition in $\mathbf{Z}{2}$
\begin{tabular}{c|cc}
$+$ & {$[0]$} & {$[1]$} \
\hline$[0]$ & {$[0]$} & {$[1]$} \
{$[1]$} & {$[1]$} & {$[0]$}
\end{tabular}
Actually, if we have any group $G$ with two elements, say the identity element $e$ and one other element $a$, then there is only one possibility for the multiplication table for $G$. We have already observed that Propositions 3.1.7 and 3.1.8 imply that in each row and column of a group table, each element of the group must occur exactly once. Since $e$ is the identity element, $e \cdot e=e, e \cdot a=a$, and $a \cdot e=a$. Since $a$ cannot be repeated in the last row of the table, we must have $a \cdot a=e$.
数学代写|抽象代数代写ABSTRACT ALGEBRA代考|ISOMORPHIC
我们需要一个正式的定义来描述两个群何时具有相同的代数性质。首先,组的元素之间应该存在一一对应的关系。这实质上意味着一个组的元素可以重命名为与第二组的元素完全对应。此外,相应元素的产品应该对应。如果G1是一个有操作的组∗和G2是一个有操作的组⋆, 那么任何函数φ:G1→G2保存产品必须具有以下属性φ(一种∗b)=φ(一种)⋆φ(b)对全部一种,b∈G1. 这在一个公式中表达了这样一个事实,即如果我们先相乘一种和b得到完全相同的答案,就像我们找到相应的元素一样φ(一种)和φ(b)在完全相同的答案中,就好像我们找到了相应的元素φ(一种)和φ(b)在G2然后计算他们的产品φ(一种)⋆φ(b)在G2. 编写这两个操作相当麻烦,因此在我们的定义中我们将省略它们,因为从上下文中应该清楚要使用哪个操作。一对一的对应词 isos 意思是“相等”,morphe 意思是“形式”。
3.4.1 定义。让G1和G2成为组,并让φ:G1→G2成为一个函数。然后φ被称为群同构如果φ是一对一的,并且
φ(一种b)=φ(一种)φ(b)
对全部一种,b∈G1. 在这种情况下,G1据说是同构的G2,这表示为G1≅G2.

数学代写|抽象代数代写abstract algebra代考 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。