如果你也在 怎样代写拓扑学topology这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。拓扑学topology在数学中,拓扑学(来自希腊语中的τόπος,”地方、位置”,和λόγος,”研究”)关注的是几何对象在连续变形下保持的属性,如拉伸、扭曲、皱缩和弯曲;也就是说,在不关闭孔、打开孔、撕裂、粘连或穿过自身的情况下。
拓扑学topology拓扑空间是一个被赋予了结构的集合,称为拓扑,它允许定义子空间的连续变形,以及更广泛地定义所有种类的连续性。欧几里得空间,以及更一般的,公制空间都是拓扑空间的例子,因为任何距离或公制都定义了一个拓扑。拓扑学中所考虑的变形是同构和同形。在这种变形下不变的属性是一种拓扑属性。拓扑属性的基本例子有:维度,它可以区分线和面;紧凑性,它可以区分线和圆;连接性,它可以区分一个圆和两个不相交的圆。
my-assignmentexpert™ 拓扑学topology作业代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。my-assignmentexpert™, 最高质量的拓扑学topology作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于统计Statistics作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此拓扑学topology作业代写的价格不固定。通常在经济学专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
my-assignmentexpert™ 为您的留学生涯保驾护航 在数学Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的拓扑学topology代写服务。我们的专家在数学Mathematics代写方面经验极为丰富,各种拓扑学topology相关的作业也就用不着 说。
我们提供的拓扑学topology及其相关学科的代写,服务范围广, 其中包括但不限于:
数学代写|拓扑学作业代写topology代考|Basic transversality
We consider the following setting:
- $M$ is a smooth $m$-manifold with (possibly empty) boundary $\partial M$.
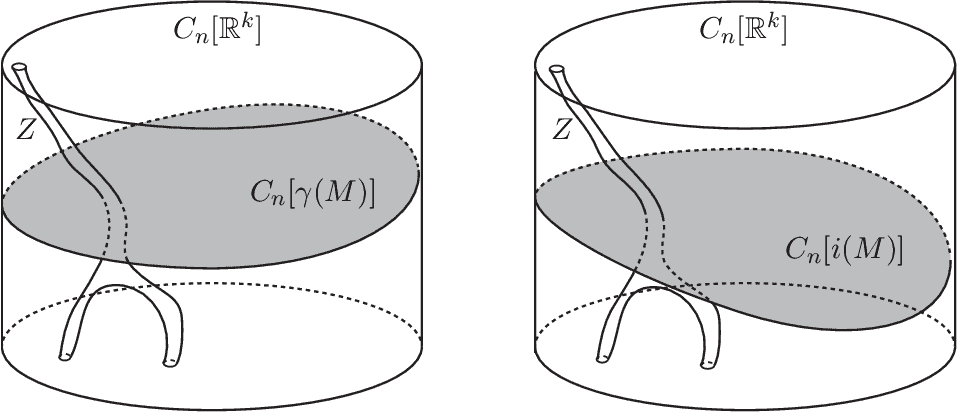
- $N$ is a smooth boundaryless $n$-manifold and $Z \subset N$ is a proper $r$ submanifold of $N$; hence $Z$ is both boundaryless and a closed subset of $N$.
- $f: M \rightarrow N$ is a smooth map. If the boundary is nonempty, then $\partial f$ denotes the restriction of $f$ to $\partial M$.
Definition 8.1. We say that $f$ is transverse to $Z$ (and we write $f \pitchfork Z$ ) if:
(1) For every $x \in M$ such that $y=f(x) \in Z$, we have
$$
T_{y} N=T_{y} Z+d_{x} f\left(T_{x} M\right) .
$$
(2) For every $x \in \partial M$ such that $\partial f(x) \in Z$, we have
$$
T_{y} N=T_{y} Z+d_{x} \partial f\left(T_{x} \partial M\right)
$$
(in other words, $\partial f \pitchfork Z$ by itself). If $\partial M=\emptyset$, this second requirement is empty.
We denote by $\pitchfork(M, N ; Z)$ the subspace of $\mathcal{E}(M, N)$ formed by the maps transverse to $Z$. If $A$ is a subset of $M$, we denote by $\pitchfork_{A}(M, N ; Z)$ the space of maps which satisfy the transversality conditions for every $x \in A$ or $x \in A \cap \partial M$, so that $\pitchfork(M, N ; Z)=\pitchfork_{M}(M, N ; Z)$.
数学代写|拓扑学作业代写topology代考|Miscellaneous transversalities
Transversality is a profound, potent, and pervasive paradigm beyond the basic results stated in the previous section. Without any pretension of completeness, we collect here a few examples of further applications.
8.2.1. Jet trasversality. First, we perform some constructions within the smooth category of open sets considered in Chapter 1 . In particular, we refer to the Taylor polynomials defined in Section 1.2. Recall that a homogeneous polynomial map of degree $k \geq 1$
$$
\mathfrak{p}: \mathbb{R}^{m} \rightarrow \mathbb{R}^{n}
$$
is of the form $\mathfrak{p}(x)=\phi(x, \ldots, x)$, where $\phi:\left(\mathbb{R}^{m}\right)^{k} \rightarrow \mathbb{R}^{n}$ is a (necessarily unique) symmetric $k$-linear map. The set $\mathcal{P}{k}(m, n)$ of these homogeneous polynomial maps has the natural structure of a finite-dimensional real vector space endowed with a standard basis, so that it is identified with $\mathbb{R}^{\operatorname{dim} \mathcal{P}{k}(m, n)}$. A polynomial map of degree $\leq r, p: \mathbb{R}^{m} \rightarrow \mathbb{R}^{n}$, is of the form
$$
p=p_{0}+p_{1}+\cdots+p_{r}
$$
where $p_{0} \in \mathbb{R}^{n}$ and for $k \geq 1, p_{k}$ is a homogeneous polynomial map of degree $k$. Denote by $J^{r}(m, n)$ the set of these polynomial maps. We can use the natural identification
$$
J^{r}(m, n)=\prod_{k=0}^{r} \mathcal{P}^{k}(m, n)
$$
to give it the structure of a finite-dimensional real vector space; $J^{r}(m, n)$ is identified with $\mathbb{R}^{\operatorname{dim} J^{r}(m, n)}$.
拓扑学代写
数学代写|拓扑学作业代写TOPOLOGY代考|BASIC TRANSVERSALITY
我们考虑以下设置:
- 米是光滑的米-歧管与p这ss一世bl是和米p吨是边界∂米.
- ñ是一个光滑的无边界n-歧管和从⊂ñ是适当的r的子流形ñ; 因此从既是无边界的又是ñ.
- F:米→ñ是一个光滑的地图。如果边界非空,则∂F表示限制F到∂米.
定义 8.1。我们说F是横向的从 一种nd在和在r一世吨和$F⋔从$如果:
1对于每一个X∈米这样是=F(X)∈从, 我们有
吨是ñ=吨是从+dXF(吨X米).
2对于每一个X∈∂米这样∂F(X)∈从, 我们有
吨是ñ=吨是从+dX∂F(吨X∂米)
一世n这吨H和r在这rds,$∂F⋔从$b是一世吨s和lF. 如果∂米=∅,这第二个要求是空的。
我们表示⋔(米,ñ;从)的子空间和(米,ñ)由横向的地图形成从. 如果一种是的一个子集米,我们表示为⋔一种(米,ñ;从)满足每个横断条件的映射空间X∈一种或者X∈一种∩∂米, 以便⋔(米,ñ;从)=⋔米(米,ñ;从).
数学代写|拓扑学作业代写TOPOLOGY代考|MISCELLANEOUS TRANSVERSALITIES
超越上一节所述的基本结果,横向性是一种深刻、有力和普遍的范式。不假装完整,我们在这里收集一些进一步应用的例子。
8.2.1。射流穿越。首先,我们在第 1 章考虑的开集的光滑范畴内进行一些构造。特别是,我们参考了 1.2 节中定义的泰勒多项式。回想一下次数的齐次多项式映射ķ≥1
$$
\mathfrak{p}: \mathbb{R}^{m} \rightarrow \mathbb{R}^{n}
$$
is of the form $\mathfrak{p}(x)=\phi(x, \ldots, x)$, where $\phi:\left(\mathbb{R}^{m}\right)^{k} \rightarrow \mathbb{R}^{n}$ is a (necessarily unique) symmetric $k$-linear map. The set $\mathcal{P}{k}(m, n)$ of these homogeneous polynomial maps has the natural structure of a finite-dimensional real vector space endowed with a standard basis, so that it is identified with $\mathbb{R}^{\operatorname{dim} \mathcal{P}{k}(m, n)}$. A polynomial map of degree $\leq r, p: \mathbb{R}^{m} \rightarrow \mathbb{R}^{n}$, is of the form
$$
p=p_{0}+p_{1}+\cdots+p_{r}
$$
where $p_{0} \in \mathbb{R}^{n}$ and for $k \geq 1, p_{k}$ is a homogeneous polynomial map of degree $k$. Denote by $J^{r}(m, n)$ the set of these polynomial maps. We can use the natural identification
$$
J^{r}(m, n)=\prod_{k=0}^{r} \mathcal{P}^{k}(m, n)
$$
to give it the structure of a finite-dimensional real vector space; $J^{r}(m, n)$ is identified with $\mathbb{R}^{\operatorname{dim} J^{r}(m, n)}$.

数学代写|拓扑学作业代写TOPOLOGY代考 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。