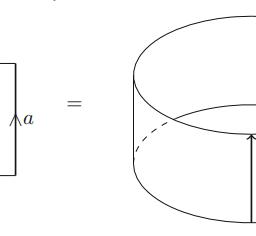如果你也在 怎样代写拓扑学topology这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。拓扑学topology在数学中,拓扑学(来自希腊语中的τόπος,”地方、位置”,和λόγος,”研究”)关注的是几何对象在连续变形下保持的属性,如拉伸、扭曲、皱缩和弯曲;也就是说,在不关闭孔、打开孔、撕裂、粘连或穿过自身的情况下。
拓扑学topology拓扑空间是一个被赋予了结构的集合,称为拓扑,它允许定义子空间的连续变形,以及更广泛地定义所有种类的连续性。欧几里得空间,以及更一般的,公制空间都是拓扑空间的例子,因为任何距离或公制都定义了一个拓扑。拓扑学中所考虑的变形是同构和同形。在这种变形下不变的属性是一种拓扑属性。拓扑属性的基本例子有:维度,它可以区分线和面;紧凑性,它可以区分线和圆;连接性,它可以区分一个圆和两个不相交的圆。
my-assignmentexpert™ 拓扑学topology作业代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。my-assignmentexpert™, 最高质量的拓扑学topology作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于统计Statistics作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此拓扑学topology作业代写的价格不固定。通常在经济学专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
my-assignmentexpert™ 为您的留学生涯保驾护航 在数学Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的拓扑学topology代写服务。我们的专家在数学Mathematics代写方面经验极为丰富,各种拓扑学topology相关的作业也就用不着 说。
我们提供的拓扑学topology及其相关学科的代写,服务范围广, 其中包括但不限于:
数学代写|拓扑学作业代写topology代考|The bordism modules of a topological space
Let $X$ be a topological space. For every $m \geq 0$, a singular smooth $m$ manifold in $X$ is a continuous map $f: M \rightarrow X$, where $M \in \mathcal{S}{m}$. Denote by $\mathcal{S}{m}(X)$
the set of such singular manifolds, to which we formally add the empty set.
Definition 10.1. $(M, f) \in \mathcal{S}_{m}(X)$ is a singular boundary if there is a compact smooth $(m+1)$-manifold with boundary $(W, \partial W)$, a diffeomorphism $\rho: M \rightarrow \partial W$, and a continuous map $F: W \rightarrow X$ such that $F \circ \rho=f$.
Let us put on $\mathcal{S}{m}(X)$ the following relation. We say that $\left(M{0}, f_{0}\right)$ is bordant with $\left(M_{1}, f_{1}\right)$, and we write $\left(M_{0}, f_{0}\right) \sim_{b}\left(M_{1}, f_{1}\right)$, if the disjoint union $\left(M_{0}, f_{0}\right)$ II $\left(M_{1}, f_{1}\right)$ is a singular boundary. It is consistent to state that $(M, f) \sim_{b} \emptyset$ if and only if $(M, f)$ is a singular boundary.
We claim that this is an equivalence relation:
- The cylinder $(M \times[0,1], F), F(x, t)=f(x)$ for every $t \in[0,1]$, establishes that $(M, f) \sim_{b}(M, f), \rho: M$ Ш $M \rightarrow(M \times{0})$ I $(M \times{1})$ being the natural inclusion.
- As the disjoint union is symmetric, then $\sim_{b}$ is also symmetric.
- Transitivity follows by gluing smooth manifolds along boundary components. Precisely, assume that $\left(W_{0}, F_{0}\right), \rho_{0}: M_{0}$ II $M_{1} \rightarrow \partial W_{0}$ realize $\left(M_{0}, f_{0}\right) \sim_{b}\left(M_{1}, f_{1}\right)$, while $\left(W_{1}, F_{1}\right), \rho_{1}: M_{1}$ II $M_{2} \rightarrow \partial W_{1}$ realize $\left(M_{1}, f_{1}\right) \sim_{b}\left(M_{2}, f_{2}\right)$. Then $F_{0}$ and $F_{1}$ match to define a smooth map $F_{2}$ on $W_{2}:=W_{0} \mathrm{~L}{\psi} W{1}$, where $\psi$ is the composition of the restriction of $\rho_{0}^{-1}$ to $\rho_{0}\left(M_{1}\right)$ with the restriction of $\rho_{1}$ to $M_{1}$. Finally, $\left(W_{2}, F_{2}\right)$ together with the disjoint union of $\rho_{0}$ restricted to $M_{0}$ and $\rho_{1}$ restricted to $M_{2}$ realize $\left(M_{0}, f_{0}\right) \sim_{b}\left(M_{2}, f_{2}\right)$.
We denote by $\eta_{m}(X)$ the quotient set $\mathcal{S}{m}(X) / \sim{b}$ and by $[M, f]$ the equivalence class of $(M, f)$.
The disjoint union is an operation on $\mathcal{S}{m}(X)$. It is immediate that it descends to the quotient; that is, $[M, f]+[N, g]:=[M$ I N, $f$ I $g]$ is a well-defined operation on $\eta{m}(X)$.
数学代写|拓扑学作业代写topology代考|Bordism covariant functors
We have the following proposition. All verifications are straightforward consequences of the definitions.
Proposition 10.5. For every $m \geq 0$,
$$
X \Rightarrow \mathcal{B}{m}(X) \text {, } $$ $$ g: X \rightarrow Y \Rightarrow g{}: \mathcal{B}{m}(X) \rightarrow \mathcal{B}{m}(Y), g_{}([M, f])=[M, g \circ f]
$$
is a covariant functor from the category of topological spaces and continuous maps to the category of $R$-modules and $R$-linear maps. That is,
$$
\begin{aligned}
&(g \circ h){}=g{} \circ h_{}, \ &\left(\mathrm{id}{X}\right){}=\mathrm{id}{\mathcal{B}{m}(X)} .
\end{aligned}
$$
In particular, if $g: X \rightarrow Y$ is a homeomorphism, then $g_{}$ is an $R$-linear isomorphism with inverse $\left(g^{-1}\right){}$. Considered up to linear isomorphism, $\mathcal{B}{m}(X)$ is an invariant of the topological type of $X$. The family introduced above of “forgetting” linear maps
$$
\left{\sigma_{m}: \mathcal{B}{m}(X ; \mathbb{Z}) \rightarrow \mathcal{B}{m}(X ; \mathbb{Z} / 2 \mathbb{Z})\right}
$$
is functorial; that is, they form commutative squares together with the respective families of $g_{}$ ‘s. We formally express it by saying that ” $g_{} \circ \sigma=$ $\sigma \circ g_{*} “$.
数学代写|拓扑学作业代写TOPOLOGY代考|Relative bordism of topological pairs
We consider topological pairs $(X, A)$, where $A$ is a subspace of $X$, and the class $\mathcal{M}{m}^{\partial}$ of compact smooth $m$-manifolds with boundary $(M, \partial M)$. This incorporates the “absolute situations” by identifying $X$ with the pair $(X, \emptyset)$ and a boundaryless manifold $M \in \mathcal{M}{m}$ with $(M, \emptyset)$.
A relative singular m-manifold in $(X, A)$ is a continuous map of pairs
$$
f:(M, \partial M) \rightarrow(X, A)
$$
where, by definition, $f(\partial M) \subset A$ and $(M, \partial M) \in \mathcal{M}{m}^{\partial}$. We set $\mathcal{M}{m}(X, A)$ as the collection of these relative singular $m$-manifolds.
Definition 10.6. $f:(M, \partial M) \rightarrow(X, A)$ is a relative singular boundary if there are continuous pair maps $F:(W, V) \rightarrow(X, A), \rho:(M, \partial M) \rightarrow$ $(Z, \partial Z)$ such that the following hold:
(1) $(W, \partial W) \in \mathcal{M}{m+1}^{\partial}$. (2) $(V, \partial V)$ and $(Z, \partial Z)$ are smooth $m$-submanifolds of $\partial W$ such that $$ \partial W=V \cup Z, V \cap Z=\partial V=\partial Z . $$ (3) $\rho:(M, \partial M) \rightarrow(Z, \partial Z)$ is a smooth diffeomorphism (preserving the orientation in the oriented case). In particular if $\partial M$ is empty, then $V$ and $Z$ are also boundaryless, $\partial W=V$ U $Z$ and $F(V) \subset A$. We put on $\mathcal{M}{m}(X, A)$ the equivalence relation
$$
\left(M_{0}, \partial M_{0}, f_{0}\right) \sim_{\mathcal{B}}\left(M_{1}, \partial M_{1}, f_{1}\right)
$$
if and only if $\left(M_{0}, \partial M_{0}, f_{0}\right) \mathrm{U}\left(-M_{1}, \partial M_{1}, f_{1}\right)$ is a relative singular boundary (in the unoriented case the sign “-” is immaterial). The verification that it is an equivalence relation (in particular the transitivity) incorporates some instances of corner smoothing, in accordance with Remark 7.16.
The disjoint union on $\mathcal{M}{m}(X, A)$ descends to an operation $+$ on the quotient set that eventually makes it an $R$-module $\mathcal{B}{m}(X, A)=\mathcal{B}_{m}(X, A ; R)$, called the relative $m$-bordism $R$-module of the topological pair $(X, A)$.
Proposition $10.5$ extends directly to the following.
拓扑学代写
数学代写|拓扑学作业代写TOPOLOGY代考|THE BORDISM MODULES OF A TOPOLOGICAL SPACE
让X是一个拓扑空间。对于每一个$m \geq 0$, a singular smooth $m$ manifold in $X$ is a continuous map $f: M \rightarrow X$, where $M \in \mathcal{S}{m}$. Denote by $\mathcal{S}{m}(X)$
the set of such singular manifolds, to which we formally add the empty set.
Definition 10.1. $(M, f) \in \mathcal{S}_{m}(X)$ is a singular boundary if there is a compact smooth $(m+1)$-manifold with boundary $(W, \partial W)$, a diffeomorphism $\rho: M \rightarrow \partial W$, and a continuous map $F: W \rightarrow X$ such that $F \circ \rho=f$.
让我们把$\mathcal{S}{m}(X)$ the following relation. We say that $\left(M{0}, f_{0}\right)$ is bordant with $\left(M_{1}, f_{1}\right)$, and we write $\left(M_{0}, f_{0}\right) \sim_{b}\left(M_{1}, f_{1}\right)$, if the disjoint union $\left(M_{0}, f_{0}\right)$ II $\left(M_{1}, f_{1}\right)$ is a singular boundary. It is consistent to state that $(M, f) \sim_{b} \emptyset$ if and only if $(M, f)$是一个奇异的边界。
我们声称这是一个等价关系:
- 气缸(米×[0,1],F),F(X,吨)=F(X)对于每个吨∈[0,1], 确定(米,F)∼b(米,F),ρ:米嘘米→(米×0)一世(米×1)是自然的包容。
- 由于不相交的并集是对称的,则∼b也是对称的。
- 传递性通过沿边界分量粘合平滑流形来实现。准确地说,假设$\left(W_{0}, F_{0}\right), \rho_{0}: M_{0}$ II $M_{1} \rightarrow \partial W_{0}$ realize $\left(M_{0}, f_{0}\right) \sim_{b}\left(M_{1}, f_{1}\right)$, while $\left(W_{1}, F_{1}\right), \rho_{1}: M_{1}$ II $M_{2} \rightarrow \partial W_{1}$ realize $\left(M_{1}, f_{1}\right) \sim_{b}\left(M_{2}, f_{2}\right)$. Then $F_{0}$ and $F_{1}$ match to define a smooth map $F_{2}$ on $W_{2}:=W_{0} \mathrm{~L}{\psi} W{1}$, where $\psi$ is the composition of the restriction of $\rho_{0}^{-1}$ to $\rho_{0}\left(M_{1}\right)$ with the restriction of $\rho_{1}$ to $M_{1}$. Finally, $\left(W_{2}, F_{2}\right)$ together with the disjoint union of $\rho_{0}$ restricted to $M_{0}$ and $\rho_{1}$ restricted to $M_{2}$ realize $\left(M_{0}, f_{0}\right) \sim_{b}\left(M_{2}, f_{2}\right)$.
我们表示这米(X)商集 $\mathcal{S} {m}X/ \sim {b}一种ndb是米,F吨H和和q在一世在一种l和nC和Cl一种ss这F米,F$.
不相交并集是对 $\mathcal{S} {m}的操作X.一世吨一世s一世米米和d一世一种吨和吨H一种吨一世吨d和sC和nds吨这吨H和q在这吨一世和n吨;吨H一种吨一世s,米,F+ñ,G:=米$一世ñ,$F$一世$G一世s一种在和ll−d和F一世n和d这p和r一种吨一世这n这n\eta {米X$.
数学代写|拓扑学作业代写TOPOLOGY代考|BORDISM COVARIANT FUNCTORS
我们有以下命题。所有验证都是定义的直接结果。
提案 10.5。对于每一个米≥0,
$$
X \Rightarrow \mathcal{B}{m}(X) \text {, } $$ $$ g: X \rightarrow Y \Rightarrow g{}: \mathcal{B}{m}(X) \rightarrow \mathcal{B}{m}(Y), g_{}([M, f])=[M, g \circ f]
$$
is a covariant functor from the category of topological spaces and continuous maps to the category of $R$-modules and $R$-linear maps. That is,
$$
\begin{aligned}
&(g \circ h){}=g{} \circ h_{}, \ &\left(\mathrm{id}{X}\right){}=\mathrm{id}{\mathcal{B}{m}(X)} .
\end{aligned}
$$
In particular, if $g: X \rightarrow Y$ is a homeomorphism, then $g_{}$ is an $R$-linear isomorphism with inverse $\left(g^{-1}\right){}$. Considered up to linear isomorphism, $\mathcal{B}{m}(X)$ is an invariant of the topological type of $X$. The family introduced above of “forgetting” linear maps
$$
\left{\sigma_{m}: \mathcal{B}{m}(X ; \mathbb{Z}) \rightarrow \mathcal{B}{m}(X ; \mathbb{Z} / 2 \mathbb{Z})\right}
$$
is functorial; that is, they form commutative squares together with the respective families of $g_{}$ ‘s. We formally express it by saying that ” $g_{} \circ \sigma=$ $\sigma \circ g_{*} “$.
数学代写|拓扑学作业代写TOPOLOGY代考|RELATIVE BORDISM OF TOPOLOGICAL PAIRS
我们考虑拓扑对$(X, A)$, where $A$ is a subspace of $X$, and the class $\mathcal{M}{m}^{\partial}$ of compact smooth $m$-manifolds with boundary $(M, \partial M)$. This incorporates the “absolute situations” by identifying $X$ with the pair $(X, \emptyset)$ and a boundaryless manifold $M \in \mathcal{M}{m}$ with $(M, \emptyset)$.
一个相对奇异的 m 流形(X,一种)是对的连续映射
F:(米,∂米)→(X,一种)
其中,根据定义,F(∂米)⊂一种和 $米,∂米\in \mathcal{M} {m}^{\partial}.在和s和吨\mathcal{M} {m}X,一种一种s吨H和C这ll和C吨一世这n这F吨H和s和r和l一种吨一世在和s一世nG在l一种rm$-歧管。
定义 10.6。F:(米,∂米)→(X,一种)如果存在连续对映射,则为相对奇异边界$F:(W, V) \rightarrow(X, A), \rho:(M, \partial M) \rightarrow$ $(Z, \partial Z)$ such that the following hold:
(1) $(W, \partial W) \in \mathcal{M}{m+1}^{\partial}$. (2) $(V, \partial V)$ and $(Z, \partial Z)$ are smooth $m$-submanifolds of $\partial W$ such that $$ \partial W=V \cup Z, V \cap Z=\partial V=\partial Z . $$ (3) $\rho:(M, \partial M) \rightarrow(Z, \partial Z)$ is a smooth diffeomorphism (preserving the orientation in the oriented case). In particular if $\partial M$ is empty, then $V$ and $Z$ are also boundaryless, $\partial W=V$ U $Z$ and $F(V) \subset A$. We put on $\mathcal{M}{m}(X, A)$ the equivalence relation
$$
\left(M_{0}, \partial M_{0}, f_{0}\right) \sim_{\mathcal{B}}\left(M_{1}, \partial M_{1}, f_{1}\right)
$$
if and only if $\left(M_{0}, \partial M_{0}, f_{0}\right) \mathrm{U}\left(-M_{1}, \partial M_{1}, f_{1}\right)$ is a relative singular boundary (in the unoriented case the sign “-” is immaterial). The verification that it is an equivalence relation (in particular the transitivity) incorporates some instances of corner smoothing, in accordance with Remark 7.16.,包含一些拐角平滑的实例。
$\mathcal{M} {m}上的不相交并集$\mathcal{M}{m}(X, A)$ descends to an operation $+$ on the quotient set that eventually makes it an $R$-module $\mathcal{B}{m}(X, A)=\mathcal{B}_{m}(X, A ; R)$, called the relative $m$-bordism $R$-module of the topological pair $(X, A)$. 直接扩展到以下。

数学代写|拓扑学作业代写TOPOLOGY代考 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。