如果你也在 怎样代写优化和运筹学Operations Research MAST30013这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。优化和运筹学Operations Research通常简称为OR,是一门研究开发和应用先进的分析方法来改善决策的学科。它有时被认为是数学科学的一个子领域。管理科学一词有时被用作同义词。
优化和运筹学Operations Research包括开发和使用广泛的解决问题的技术和方法,以追求改善决策和效率,如模拟、数学优化、排队理论和其他随机过程模型、马尔科夫决策过程、计量经济学方法、数据包络分析、神经网络、专家系统、决策分析和分析层次过程。由于这些领域的大部分都具有计算和统计的性质,因此操作学也与计算机科学和分析学有着密切的联系。面临新问题的运筹学研究人员必须根据系统的性质、改进的目标以及时间和计算能力的限制,来确定这些技术中哪种技术是最合适的,或者针对手头的问题(以及之后的那类问题)开发一种新的技术。
my-assignmentexpert™优化和运筹学Operations Research代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。my-assignmentexpert™, 最高质量的优化和运筹学Operations Research作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于统计Statistics作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此优化和运筹学Operations Research作业代写的价格不固定。通常在经济学专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
my-assignmentexpert™ 为您的留学生涯保驾护航 在澳洲代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的澳洲代写服务。我们的专家在优化和运筹学Operations Research代写方面经验极为丰富,各种优化和运筹学Operations Research相关的作业也就用不着 说。
我们提供的优化和运筹学Operations Research MAST30013及其相关学科的代写,服务范围广, 其中包括但不限于:

澳洲代考|优化和运筹学代考Operations Research代考|MODEL DEVELOPMENT
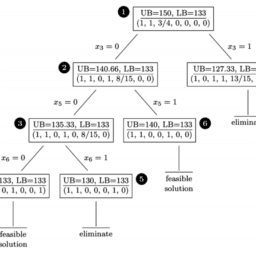
Development of model is important for executing problem analysis. The main purpose is to evaluate different alternatives. It has been emphasized that OR discipline focusses on the application of quantitative tools to help in decision-making by adopting a systematic approach to problem solving. This approach uses mathematical models that, through mathematical expressions, equations and symbols, represent real problem or situations. Models simulate real situation. They are used to examine an optimal solution in as real environment as possible. Models are a very effective way to analyse and make inferences about real problems as they are constructed as an archetype of problem at hand. For instance, to examine the effect of variation in total units to be supplied on cost of transportation, a model could be constructed involving cause-and-effect variables. In this case, units that are to be transported would be causal variables, and cost of transportation is the outcome or effect. By varying or experimenting with causal variables, we can analyse the effect on cost. This process involves less time and cost, and also is less risky than doing experimentation practically. From centre A to $B$, if cost of transporting one unit is $\$ 10$ per unit, then a mathematical model of situation can be represented by equation: Cost $(C)=10 x$; where $\mathrm{x}$ is the number of units. For 1 unit, cost would be $\$ 10$; for 2 units, it would be $\$ 20$; and so on. Decision maker has to decide which conclusion suits best.
However, model conclusion provides the best results only if they represent the real situation accurately. That is why problem identification and formulation are so important. In the above example, cost could be the function of other variables and not only the number of units such as the size of truck or the number of possible routes, etc. Thus, more closely a model represents the problem, more conclusive results would be.
The following case explains the development of a model by highlighting various elements used in its formulation.
澳洲代考|优化和运筹学代考Operations Research代考|CASE: ADIDAS AG
Adidas $\mathrm{AG}$ is the second largest sportswear manufacturer in the world. It is primarily a male brand catering to the needs of burgeoning young population with disposable income. The company is looking to open retail stores in a major capital city. The retailer has shortlisted two locations: a famous shopping mall (location A) located at the outskirts of a city and a popular shopping plaza (location B) located at the heart of the city. Adidas wanted to estimate the number of units that could be sold from two geographic areas, which would maximize sales. Profit generated per unit from location A was estimated to be $\$ 80$, whereas from location B was $\$ 40$.
Profit was found to be a direct function of the number of units sold, which in turn depended on the accessibility of store measured in terms of distance, population density of each geographic area and lastly median household income. From location analysis, a retailer considered his shop to be attractive in the range of 14 miles. Location A customers needed to travel an average distance of 9 miles, whereas customers of location B travelled 4 miles to access offerings of the retail shop. Second, population density in terms of the number of households was estimated to be 2,000 and 7,000 for $\mathrm{A}$ and B, respectively. Population density represents prospective clients or potential demand from a stores’ trade area. A household can buy more than one unit, so the retailer decided to cater to maximum demand of 10,000 households. Finally, demographic data indicated that the maximum average household income of two demand areas was $\$ 20,000$. Median household income would decide the extent to which customers would be willing to spend on products. It was found that the mall (location A) population had a median income of $\$ 5,000$ and the shopping plaza (location B) population had $\$ 10,000$. Under these circumstances, the retailer wants to estimate the maximum profit that could be earned by selling specific units to customers of $A$ and $B$.
优化和运筹学代写
澳洲代考|优化和运筹学代考OPERATIONS RESEARCH代考|MODEL DEVELOPMENT
模型的开发对于执行问题分析很重要。主要目的是评估不同的备选方案。有人强调,OR 学科侧重于应用定量工具,通过采用系统的方法解决问题来帮助决策。这种方法使用数学模型,通过数学表达式、方程式和符号,代表实际问题或情况。模型模拟真实情况。它们用于在尽可能真实的环境中检查最佳解决方案。模型是分析和推断实际问题的一种非常有效的方法,因为它们被构建为手头问题的原型。例如,要检查要供应的总单位的变化对运输成本的影响,可以构建一个包含因果变量的模型。在这种情况下,要运输的单位将是因果变量,运输成本是结果或效果。通过改变或试验因果变量,我们可以分析对成本的影响。这个过程需要更少的时间和成本,并且比实际进行实验的风险更小。从中心 A 到乙, 如果运输一个单位的成本是$10每单位,那么情况的数学模型可以用等式表示: 成本(C)=10X; 在哪里X是单位数。对于 1 个单位,成本为$10; 对于 2 个单位,它将是$20; 等等。决策者必须决定哪个结论最适合。
然而,模型结论只有在准确地代表真实情况时才能提供最佳结果。这就是为什么问题识别和制定如此重要的原因。在上面的例子中,成本可能是其他变量的函数,而不仅仅是单位的数量,例如卡车的大小或可能的路线的数量等。因此,一个模型更能代表问题,更确凿的结果将是.
以下案例通过突出模型中使用的各种元素来解释模型的开发。
澳洲代考|优化和运筹学代考OPERATIONS RESEARCH代考|CASE: ADIDAS AG
阿迪达斯一个G是世界第二大运动服装制造商。它主要是一个男性品牌,以满足具有可支配收入的新兴年轻人口的需求。该公司正寻求在主要首都城市开设零售店。该零售商入围了两个地点:一个著名的购物中心l○C一个吨一世○n一个位于城市郊区和热门购物广场l○C一个吨一世○n乙位于市中心。阿迪达斯想要估计可以从两个地理区域销售的单位数量,这将最大限度地提高销售额。地点 A 的每单位产生的利润估计为$80,而从位置 B 是$40.
利润被发现是售出单位数量的直接函数,而这又取决于商店的可达性,以距离、每个地理区域的人口密度和最后的家庭收入中位数来衡量。根据位置分析,零售商认为他的商店在 14 英里范围内具有吸引力。位置 A 的客户需要平均行驶 9 英里,而位置 B 的客户需要行驶 4 英里才能访问零售店的产品。其次,以家庭数量计算的人口密度估计为 2,000 户,而 7,000 户一个和 B,分别。人口密度代表潜在客户或商店贸易区域的潜在需求。一个家庭可以购买多个单元,因此零售商决定满足 10,000 个家庭的最大需求。最后,人口统计数据表明,两个需求地区的最大平均家庭收入是$20,000. 家庭收入中位数将决定客户愿意在产品上花费的程度。才发现商场l○C一个吨一世○n一个人口平均收入为$5,000和购物广场l○C一个吨一世○n乙人口有$10,000. 在这种情况下,零售商想要估计通过向特定客户销售特定产品可以获得的最大利润。一个和乙.

澳洲代考|优化和运筹学代考Operations Research代考 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。
微观经济学代写
微观经济学是主流经济学的一个分支,研究个人和企业在做出有关稀缺资源分配的决策时的行为以及这些个人和企业之间的相互作用。my-assignmentexpert™ 为您的留学生涯保驾护航 在数学Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在图论代写Graph Theory代写方面经验极为丰富,各种图论代写Graph Theory相关的作业也就用不着 说。
线性代数代写
线性代数是数学的一个分支,涉及线性方程,如:线性图,如:以及它们在向量空间和通过矩阵的表示。线性代数是几乎所有数学领域的核心。
博弈论代写
现代博弈论始于约翰-冯-诺伊曼(John von Neumann)提出的两人零和博弈中的混合策略均衡的观点及其证明。冯-诺依曼的原始证明使用了关于连续映射到紧凑凸集的布劳威尔定点定理,这成为博弈论和数学经济学的标准方法。在他的论文之后,1944年,他与奥斯卡-莫根斯特恩(Oskar Morgenstern)共同撰写了《游戏和经济行为理论》一书,该书考虑了几个参与者的合作游戏。这本书的第二版提供了预期效用的公理理论,使数理统计学家和经济学家能够处理不确定性下的决策。
微积分代写
微积分,最初被称为无穷小微积分或 “无穷小的微积分”,是对连续变化的数学研究,就像几何学是对形状的研究,而代数是对算术运算的概括研究一样。
它有两个主要分支,微分和积分;微分涉及瞬时变化率和曲线的斜率,而积分涉及数量的累积,以及曲线下或曲线之间的面积。这两个分支通过微积分的基本定理相互联系,它们利用了无限序列和无限级数收敛到一个明确定义的极限的基本概念 。
计量经济学代写
什么是计量经济学?
计量经济学是统计学和数学模型的定量应用,使用数据来发展理论或测试经济学中的现有假设,并根据历史数据预测未来趋势。它对现实世界的数据进行统计试验,然后将结果与被测试的理论进行比较和对比。
根据你是对测试现有理论感兴趣,还是对利用现有数据在这些观察的基础上提出新的假设感兴趣,计量经济学可以细分为两大类:理论和应用。那些经常从事这种实践的人通常被称为计量经济学家。
Matlab代写
MATLAB 是一种用于技术计算的高性能语言。它将计算、可视化和编程集成在一个易于使用的环境中,其中问题和解决方案以熟悉的数学符号表示。典型用途包括:数学和计算算法开发建模、仿真和原型制作数据分析、探索和可视化科学和工程图形应用程序开发,包括图形用户界面构建MATLAB 是一个交互式系统,其基本数据元素是一个不需要维度的数组。这使您可以解决许多技术计算问题,尤其是那些具有矩阵和向量公式的问题,而只需用 C 或 Fortran 等标量非交互式语言编写程序所需的时间的一小部分。MATLAB 名称代表矩阵实验室。MATLAB 最初的编写目的是提供对由 LINPACK 和 EISPACK 项目开发的矩阵软件的轻松访问,这两个项目共同代表了矩阵计算软件的最新技术。MATLAB 经过多年的发展,得到了许多用户的投入。在大学环境中,它是数学、工程和科学入门和高级课程的标准教学工具。在工业领域,MATLAB 是高效研究、开发和分析的首选工具。MATLAB 具有一系列称为工具箱的特定于应用程序的解决方案。对于大多数 MATLAB 用户来说非常重要,工具箱允许您学习和应用专业技术。工具箱是 MATLAB 函数(M 文件)的综合集合,可扩展 MATLAB 环境以解决特定类别的问题。可用工具箱的领域包括信号处理、控制系统、神经网络、模糊逻辑、小波、仿真等。