如果你也在 怎样代写概率论Probability Theory 这个学科遇到相关的难题，请随时右上角联系我们的24/7代写客服。概率论Probability Theory作为统计学的数学基础，对许多涉及数据定量分析的人类活动至关重要。概率论的方法也适用于对复杂系统的描述，只对其状态有部分了解，如在统计力学或顺序估计。二十世纪物理学的一个伟大发现是量子力学中描述的原子尺度的物理现象的概率性质。

概率论Probability Theory STAT131的核心课题包括离散和连续随机变量、概率分布和随机过程（为非决定性或不确定的过程或测量量提供数学抽象，这些过程或测量量可能是单一发生的，或以随机方式随时间演变）。尽管不可能完美地预测随机事件，但对它们的行为可以有很多说法。概率论中描述这种行为的两个主要结果是大数法则和中心极限定理。概率论是与概率有关的数学分支。虽然有几种不同的概率解释，但概率论以严格的数学方式处理这一概念，通过一组公理来表达它。

同学们在留学期间，都对各式各样的作业考试很是头疼，如果你无从下手，不如考虑my-assignmentexpert™！

my-assignmentexpert™提供最专业的一站式服务：Essay代写，Dissertation代写，Assignment代写，Paper代写，Proposal代写，Proposal代写，Literature Review代写，Online Course，Exam代考等等。my-assignmentexpert™专注为留学生提供Essay代写服务，拥有各个专业的博硕教师团队帮您代写，免费修改及辅导，保证成果完成的效率和质量。同时有多家检测平台帐号，包括Turnitin高级账户，检测论文不会留痕，写好后检测修改，放心可靠，经得起任何考验！

数学代写|概率论代考Probability Theory代写|An application in genetics, Hardy’s law

Mendel’s theory of heredity has seen its full flowering with the discovery of how hereditary information is carried by genes. The passing of genetic characteristics to offspring is a chance-driven process and provides fertile ground for illustration of probabilistic methods. Our discussion is necessarily simplisticthe specialised nature of the subject inhibits a detailed study here, no less the author’s own very limited knowledge of the subject-but the ample scope for probabilistic models should be apparent.

The blueprint of hereditary features characteristic of an organism is carried within its genome, an identical copy of which is maintained within each cell. The genome consists of a complete set of genes, each of which is a specific grouping of nucleic acids, with the genes organised structurally into chromosomes. Heritable characteristics are governed by one or more genes which are located at specific points (or loci) on a chromosome. As shown in Figure 2, it may be helpful to form an image of a chromosome as a string on which are clustered a large number of beads (the genes) in a specific order.

Individual genes themselves may exist in two or more forms called alleles which are small variations in the basic molecular structure of the gene. The salient point for our purposes is that in diploid organisms (which include most higher life forms) each gene exists in pairs (the genotype) at the chromosome locus. The specific genotype of the organism ultimately informs observed variations in physical traits such as albinism, eye colour, or haemophilia that are controlled by the gene. Genotypes are classified as homozygous (meaning that the alleles forming the gene pair are identical) or heterozygous (meaning that the alleles forming the gene pair are different). We consider the simplest setting where a gene can exist as one of two alleles $A$ and $a$. The organism can then belong to one of the three genotypes $A A, A a$, and $a a$ of which the first and third are homozygous and the second is heterozygous (the order is irrelevant in the arrangement $A a)$

Reproductive cells (or gametes) are special in that they contain only one of the genes in the genotype. Thus, the gametes of a type $A A$ or type $a a$ organism contain genes of only one type, $A$ or $a$, respectively, but the gametes of a type $A a$ organism contain $A$-gametes and $a$-gametes in equal numbers.

数学代写|概率论代考Probability Theory代写|Independent trials

The independence of a sequence of events entails the checking of a formidable number of product conditions. In the most important situation in practice, however, the validity of the conditions will be almost obvious and will entail no necessity for checks. This is the situation corresponding to independent trials.
The setting is already familiar to us in examples such as repeated coin tosses or throws of a die. We will only be concerned here with situations where individual experiments (or trials) result in a discrete set of outcomes.

ExAmplE 1) Craps. In the popular dice game of craps a player rolls two dice and sums the face values obtained. She wins immediately if she rolls 7 or 11; if, on the other hand, she rolls 2,3, or 12 then she loses immediately. If she rolls any of the six remaining numbers she then proceeds to roll the dice repeatedly, the game terminating at the first roll in which she either repeats her initial roll or rolls a 7; she wins if the game terminates with her initial roll, loses if it terminates with a 7. What constitutes fair odds in this setting?

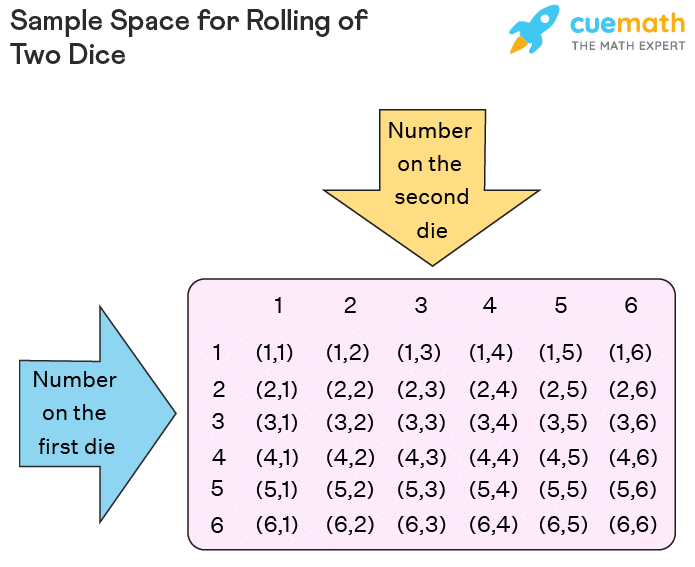
It is natural to allocate equal probability $1 / 36$ to each pair of face values $(1,1),(1,2), \ldots,(6,6)$ for the two dice. Let $E_i$ denote the event that any given roll results in the value $i$ for the sum of face values and let $p_i=\mathbf{P}\left(E_i\right)$. By summing over the probabilities of the outcomes engendering each of the $E_i$ we obtain the probabilities listed in Table I.2.1, redisplayed here for convenience:
\begin{tabular}{c|ccccccccccc}
$\mathrm{i}$ & 2 & 3 & 4 & 5 & 6 & 7 & 8 & 9 & 10 & 11 & 12 \
\hline $\mathrm{p}i$ & $\frac{1}{36}$ & $\frac{2}{36}$ & $\frac{3}{36}$ & $\frac{4}{36}$ & $\frac{5}{36}$ & $\frac{6}{36}$ & $\frac{5}{36}$ & $\frac{4}{36}$ & $\frac{3}{36}$ & $\frac{2}{36}$ & $\frac{1}{36}$ \end{tabular} Let $W$ denote the event that the player eventually wins the game. By conditioning on the outcome of the first roll, we have $\mathbf{P}(W)=\sum{i=2}^{12} \mathbf{P}\left(W \mid E_i\right) \mathbf{P}\left(E_i\right)$

by total probability. Now $\mathbf{P}\left(W \mid E_2\right)=\mathbf{P}\left(W \mid E_3\right)=\mathbf{P}\left(W \mid E_{12}\right)=0$ and $\mathbf{P}\left(W \mid E_7\right)=\mathbf{P}\left(W \mid E_{11}\right)=1$. It only remains to evaluate $\mathbf{P}\left(W \mid E_i\right)$ for $i \in{4,5,6,8,9,10}$.

Suppose now that the game proceeds past the first roll. Let $W_n$ denote the event that the game terminates in the $n$th roll. By total probability, for each $i \in{4,5,6,8,9,10}$, we then have $\mathbf{P}\left(W \mid E_i\right)=\sum_{n=2}^{\infty} \mathbf{P}\left(W_n \mid E_i\right)$. Given that the player rolls $i$ initially, she wins on the nth roll if, and only if, rolls 2 through $n-1$ are neither $i$ nor 7 and roll $n$ is $i$. By independence of the rolls it follows that $\mathbf{P}\left(W_n \mid E_i\right)=\left(1-p_i-p_7\right)^{n-2} p_i$ and so, by summing a geometric series, we obtain
$$
\mathbf{P}\left(W \mid E_i\right)=\sum_{n=2}^{\infty}\left(1-p_i-p_7\right)^{n-2} p_i=\frac{p_i}{p_i+p_7}
$$

概率论代写

数学代写|概率论代考PROBABILITY THEORY代写|AN APPLICATION IN GENETICS, HARDY’S LAW

孟德尔的遗传理论随着发现基因如何携带遗传信息而全面开花。将遗传特征传递给后代是一个机会驱动的过程，为说明概率方法提供了肥沃的土壤。我们的讨论必 然是简单化的一一该主题的专业性质阻碍了此处的详细研究，至少作者自己对该主题的知识非常有限一一但概率模型的广泛范围应该是显而易见的。
生物体的遗传特征监图在其基因组中携带，每个细胞中都保存着相同的副本。基因组由一整套基因组成，每个基因都是一组特定的核酸，基因在结构上组织成染色 体。可遗传的特征由位于特定点的一个或多个基因控制orloci一条染色体上。如图 2 所示，将染色体图像形成为串有大量珠子的串可能会有所帮助thegenes按特定 顺序。
单个基因本身可能以两种或多种形式存在，称为等位基因，它们是基因基本分子结构的微小变化。我们目的的重点是在二倍体生物中 whichincludemosthigherlifeforms 每个基因成对存在thegenotype在染色体位点。生物体的特定基因型最终会告知观察到的身体特征的变化，例如白化病、 眼睛颜色或由该基因控制的血友病。基因型被归类为纯合子meaningthattheallelesformingthegenepairareidentical或杂合子
meaningthattheallelesformingthegenepairaredifferent. 我们考虑最简单的设置，其中一个基因可以作为两个等位基因之一存在 $A$ 和 $a$. 然后该生物体可以 属于三种基因型之一 $A A, A a$ ，和 $a a$ 其中第一个和第三个是纯合子，第二个是杂合子theorderisirrelevantinthearrangement $\$ A a$ \$
生殖细胞 orgametes特殊之处在于它们仅包含基因型中的一个基因。因此，一种类型的配子 $A A$ 或输入 $a a$ 生物体只包含一种类型的基因， $A$ 或者 $a ，$ 分别是一种类 型的配子 $A a$ 有机体含有 $A$-配子和 $a$-配子数量相等。

数学代写|概率论代考PROBABILITY THEORY代写|INDPENDENT TRIALS

一系列事件的独立性需要检音大量的产品条件。然而，在实践中最重要的情况下，条件的有效性几乎是显而易见的，不需要检变。这是对应独立试验的情况。 在重复抛硬市或掷骰子等示例中，我们已经很熟悉这种设置。我们在这里只关心个别实验的情况ortrials导致一组离散的结果。
示例 1) 掷骰子。在流行的掷骰子骰子游戏中，玩家掷两个骰子并对获得的面值求和。如果她掷出 7 或 11 ，她立即获胜；另一方面，如果她㧷出 2,3 或 12 ，那么她立 即输了。如果她掷出剩下的六个数字中的任何一个，她就会继续重复掷骰子，游戏在第一次掷骰时终止，她要么重垣她最初的掷骰，要么掷出 7；如果游戏以她的 初始掷哿结束，她就嬴了，如果以 7 结束，她就输了。在这种情况下，什么构成公平赔率?
分配等概率是很自然的 $1 / 36$ 每对面值 $(1,1),(1,2), \ldots,(6,6)$ 对于两个骰子。让 $E_i$ 表示任何给定掷骰结果的事件 $i$ 对于面值的总和，让 $p_i=\mathbf{P}\left(E_i\right)$. 通过对产生每 个结果的概率求和 $E_i$ 我们获得表 1.2 .1 中列出的概率，为方便起见在此处重新显示:
让 $W$ 表示玩家最终赢得游戏的事件。通过以第一卷的结果为条件，我们有 $\mathbf{P}(W)=\sum i=2^{12} \mathbf{P}\left(W \mid E_i\right) \mathbf{P}\left(E_i\right)$
按总概率。现在 $\mathbf{P}\left(W \mid E_2\right)=\mathbf{P}\left(W \mid E_3\right)=\mathbf{P}\left(W \mid E_{12}\right)=0$ 和 $\mathbf{P}\left(W \mid E_7\right)=\mathbf{P}\left(W \mid E_{11}\right)=1$. 只剩下评价了 $\mathbf{P}\left(W \mid E_i\right)$ 为了 $i \in 4,5,6,8,9,10$.
现在假设游戏进行到第一次掷羿之后。让 $W_n$ 表示游戏在 $n$ 第卷。按总概率，对于每个 $i \in 4,5,6,8,9,10$, 那么我们有 $\mathbf{P}\left(W \mid E_i\right)=\sum \sum_{n=2}^{\infty} \mathbf{P}\left(W_n \mid E_i\right)$. 鉴于玩家 滚动 $i$ 最初，当且仅当第 2 次通过时，她在第 $\mathrm{n}$ 次掷軗中获胜 $n-1$ 两者都不是 $i$ 也不是 7 和滚动 $n$ 是 $i$. 通过卷的独立性，它荁楿 $\mathrm{P}\left(W_n \mid E_i\right)=\left(1-p_i-p_7\right)^{n-2} p_i$ 因此，通过对几何级数求和，我们得到
$$
\mathbf{P}\left(W \mid E_i\right)=\sum_{n=2}^{\infty}\left(1-p_i-p_7\right)^{n-2} p_i=\frac{p_i}{p_i+p_7}
$$

数学代写|概率论代考Probability Theory代写 请认准exambang™. exambang™为您的留学生涯保驾护航。

微观经济学代写

微观经济学是主流经济学的一个分支，研究个人和企业在做出有关稀缺资源分配的决策时的行为以及这些个人和企业之间的相互作用。my-assignmentexpert™ 为您的留学生涯保驾护航 在数学Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在图论代写Graph Theory代写方面经验极为丰富，各种图论代写Graph Theory相关的作业也就用不着 说。

线性代数代写

线性代数是数学的一个分支，涉及线性方程，如：线性图，如：以及它们在向量空间和通过矩阵的表示。线性代数是几乎所有数学领域的核心。

博弈论代写

现代博弈论始于约翰-冯-诺伊曼（John von Neumann）提出的两人零和博弈中的混合策略均衡的观点及其证明。冯-诺依曼的原始证明使用了关于连续映射到紧凑凸集的布劳威尔定点定理，这成为博弈论和数学经济学的标准方法。在他的论文之后，1944年，他与奥斯卡-莫根斯特恩（Oskar Morgenstern）共同撰写了《游戏和经济行为理论》一书，该书考虑了几个参与者的合作游戏。这本书的第二版提供了预期效用的公理理论，使数理统计学家和经济学家能够处理不确定性下的决策。

微积分代写

微积分，最初被称为无穷小微积分或 “无穷小的微积分”，是对连续变化的数学研究，就像几何学是对形状的研究，而代数是对算术运算的概括研究一样。

它有两个主要分支，微分和积分；微分涉及瞬时变化率和曲线的斜率，而积分涉及数量的累积，以及曲线下或曲线之间的面积。这两个分支通过微积分的基本定理相互联系，它们利用了无限序列和无限级数收敛到一个明确定义的极限的基本概念 。

计量经济学代写

什么是计量经济学？
计量经济学是统计学和数学模型的定量应用，使用数据来发展理论或测试经济学中的现有假设，并根据历史数据预测未来趋势。它对现实世界的数据进行统计试验，然后将结果与被测试的理论进行比较和对比。

根据你是对测试现有理论感兴趣，还是对利用现有数据在这些观察的基础上提出新的假设感兴趣，计量经济学可以细分为两大类：理论和应用。那些经常从事这种实践的人通常被称为计量经济学家。

Matlab代写

MATLAB 是一种用于技术计算的高性能语言。它将计算、可视化和编程集成在一个易于使用的环境中，其中问题和解决方案以熟悉的数学符号表示。典型用途包括：数学和计算算法开发建模、仿真和原型制作数据分析、探索和可视化科学和工程图形应用程序开发，包括图形用户界面构建MATLAB 是一个交互式系统，其基本数据元素是一个不需要维度的数组。这使您可以解决许多技术计算问题，尤其是那些具有矩阵和向量公式的问题，而只需用 C 或 Fortran 等标量非交互式语言编写程序所需的时间的一小部分。MATLAB 名称代表矩阵实验室。MATLAB 最初的编写目的是提供对由 LINPACK 和 EISPACK 项目开发的矩阵软件的轻松访问，这两个项目共同代表了矩阵计算软件的最新技术。MATLAB 经过多年的发展，得到了许多用户的投入。在大学环境中，它是数学、工程和科学入门和高级课程的标准教学工具。在工业领域，MATLAB 是高效研究、开发和分析的首选工具。MATLAB 具有一系列称为工具箱的特定于应用程序的解决方案。对于大多数 MATLAB 用户来说非常重要，工具箱允许您学习和应用专业技术。工具箱是 MATLAB 函数（M 文件）的综合集合，可扩展 MATLAB 环境以解决特定类别的问题。可用工具箱的领域包括信号处理、控制系统、神经网络、模糊逻辑、小波、仿真等。