如果你也在 怎样代写组合学Combinatorics 这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。组合学Combinatorics是数学的一个领域,主要涉及计数(作为获得结果的手段和目的)以及有限结构的某些属性。主要涉及计数,作为获得结果的手段和目的,以及有限结构的某些属性。它与数学的许多其他领域密切相关,有许多应用,从逻辑学到统计物理学,从进化生物学到计算机科学。
组合学Combinatorics因其解决的问题的广泛性而闻名。组合问题出现在纯数学的许多领域,特别是在代数、概率论、拓扑学和几何学中,以及在其许多应用领域。许多组合问题在历史上被孤立地考虑,对某个数学背景下出现的问题给出一个临时性的解决方案。然而,在二十世纪后期,强大而普遍的理论方法被开发出来,使组合学本身成为一个独立的数学分支。组合学最古老和最容易理解的部分之一是图论,它本身与其他领域有许多自然联系。在计算机科学中,组合学经常被用来获得算法分析中的公式和估计。
组合学Combinatorics代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。 最高质量的组合学Combinatorics作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此组合学Combinatorics作业代写的价格不固定。通常在专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
同学们在留学期间,都对各式各样的作业考试很是头疼,如果你无从下手,不如考虑my-assignmentexpert™!
my-assignmentexpert™提供最专业的一站式服务:Essay代写,Dissertation代写,Assignment代写,Paper代写,Proposal代写,Proposal代写,Literature Review代写,Online Course,Exam代考等等。my-assignmentexpert™专注为留学生提供Essay代写服务,拥有各个专业的博硕教师团队帮您代写,免费修改及辅导,保证成果完成的效率和质量。同时有多家检测平台帐号,包括Turnitin高级账户,检测论文不会留痕,写好后检测修改,放心可靠,经得起任何考验!
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
我们在数学Mathematics代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在组合学Combinatorics代写方面经验极为丰富,各种组合学Combinatorics相关的作业也就用不着 说。
数学代写|组合学代写Combinatorics代考|Listing Structures with Symmetries
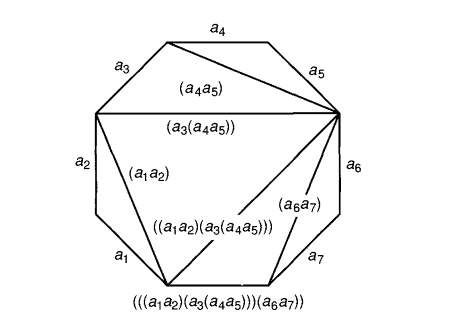
By using decision trees, introduced in Chapter 3 , we can produce our list $C$ of canonical representatives. There are many ways to go about it. We’ll illustrate this by some examples. Many of the examples are based on the Ferris wheel problem of Example 1.12 (p. 13): How many distinct six long circular sequences of ones and twos are there?
Example 4.7 A straightforward method One approach to the Ferris wheel problem is to simply generate all sequences and reject those that are equivalent to an earlier one in the lex order. For example, we would reject both 121121 and 211211 because they are equivalent to 112112 , which occurs earlier in lex order.
We can reduce the size of the decision tree by being careful; e.g., the sequence that starts 1211 … can never be lexically least because we could shift it two positions to get $11 \ldots 12$.
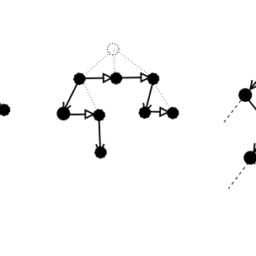
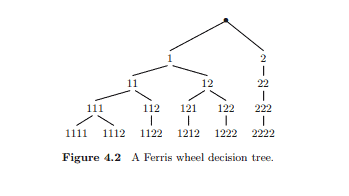
Even with these ideas, the decision tree is rather large. Hence, we’ll shorten the problem we’ve been considering to sequences of length 4 . The decision tree is shown in Figure 4.2. It is simply the tree for generating all functions from $4$ to 2 with those functions which have a (lexically) smaller circular shift removed. How did we do the removal? When we decided to begin with 2 , there was no possibility of ever choosing a 1 – a circular shift would begin with 1 and so be smaller. Also, if any 2’s are present, we can never end with a 1 because a circular shift that moved it to the front would produce a smaller sequence. This rule was applied to determine the possible decisions at 112,121 and 122. This explains everything that’s missing from the full tree for $2^4$.
This approach can get rather unwieldy when doing larger problems by hand. Try using it for the six long Ferris wheel.
Example 4.8 Another problem Four identical spheres are glued together so that three of them lie at the vertices of an equilateral triangle and the fourth lies at the center. That is, the centers of the spheres lie in a plane and three of the centers are at the corners of an equilateral triangle while the fourth is in the center. Thus, the sphere arrangement remains unchanged in appearance if it is flipped over about any of three axes or if it is rotated 120 degrees about an axis that passes through the center of the center sphere and is perpendicular to the plane of the centers. Draw yourself a picture to illustrate this – it is very useful to get into the habit of drawing pictures to help visualize problems like this.
We have four tiny identical red balls and four tiny identical green balls. The balls are to be placed in the spheres so that each sphere contains exactly two balls. How many arrangements are possible?
The calculation can be done with the help of a decision tree. The first decision could be the number of red balls to be placed in the center sphere. If no red balls are placed in the center sphere, then two green balls must be placed there and two in the outer spheres. Those two in the outer spheres can either be placed in the same sphere or in different spheres. Proceeding in this sort of way, we can construct a decision tree. You should do this, verifying that exactly six distinct arrangements are possible.
数学代写|组合学代写Combinatorics代考|Counting Structures with Symmetries
We’ve been using “equivalent” rather loosely without saying what it means. Since ambiguous terms provide an easy way to make errors, we should define it.
Definition 4.2 Equivalence An equivalence relation on a set $S$ is a partition of $S$. We say that $s, t \in S$ are equivalent if and only if they belong to the same block of the partition. If the symbol $\sim$ denotes the equivalence relation, then we write $s \sim t$ to indicate that $s$ and $t$ are equivalent. An equivalence class is a subset of $S$ consisting of all objects in the set that are equivalent to some object; i.e., an equivalence class is a block of the partition.
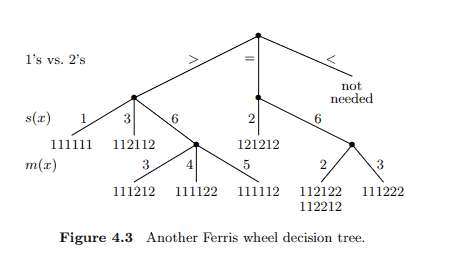
Returning to our circular sequence problem, what do the equivalence classes look like? First, 111111 is in a class by itself because all rotations give us the same sequence again. Likewise, 222222 is in a class by itself. The sequences ${121212,212121}$ is a third equivalence class. The sequences 112112 and 122122 are in different equivalence classes, each of which contains 3 sequences. So far, we have 5 equivalence classes containing a total of 10 sequences. What about the remaining $2^6-10=54$ sequences? It turns out that they fall into 9 equivalence classes of 6 sequences each. Thus there are $5+9=14$ equivalence classes; that is, the answer to our circular sequence problem is 14 .
This method is awkward for larger problems. You might try to do 12 long circular sequences of ones, twos and threes, where the answer is 44,368 . Burnside’s Lemma allows us to do such problems more easily. In order to state and prove it we need some observations about the symmetries.
In our problem the symmetries are rotations of the Ferris wheel through $0^{\circ}, 60^{\circ}, 120^{\circ}, 180^{\circ}$, $240^{\circ}$ and $300^{\circ}$. These correspond to reading a sequence circularly starting with the first, second, $\ldots$ and sixth positions, respectively. Let $S$ be the set of all six long sequences of zeroes and ones. The six symmetries correspond to six permutations of $S$ by means of the circular reading. For example, if $g_i$ is the permutation that starts reading in position $i+1$, then $g_1(111122)=111221$ and $g_3(111122)=122111$. Note that $g_0(x)=x$ for all $x$. Alternatively, we can think of $g_i$ as shifting the sequence “circularly” to the left by $i$ positions. The set $G=\left{g_0, \ldots, g_5\right}$ has some important properties, namely
(G-1) There is an $e \in G$ such that $e(x)=x$ for all $x \in S$.
(G-2) If $f \in G$, then the inverse of $f$ exists and $f^{-1} \in G$.
(G-3) If $f, g \in G$, then the composition $f g$ is in $G$.
组合学代写
数学代写|组合学代写Combinatorics代考|Listing Structures with Symmetries
通过使用第三章中介绍的决策树,我们可以生成我们的规范代表列表$C$。有很多方法可以做到这一点。我们将通过一些例子来说明这一点。许多例子都是基于例1.12中的摩天轮问题:有多少个不同的6个由1和2组成的长圆形序列?解决费里斯轮问题的一种方法是简单地生成所有序列,并拒绝那些在lex顺序中与先前序列等效的序列。例如,我们将拒绝121121和211211,因为它们等价于以lex顺序出现在前面的112112。
我们可以通过谨慎地减小决策树的大小;例如,从1211…开始的序列在词法上永远不可能是最小的,因为我们可以把它移动两个位置得到$11 \ldots 12$ .
即使有这些想法,决策树还是相当大的。因此,我们将一直考虑的问题缩短为长度为4的序列。决策树如图4.2所示。它只是用于生成从$4$到2的所有函数的树,其中删除了(词法上)较小的循环移位的函数。我们是怎么去除的?当我们决定从2开始时,就不可能选择1了——圆移从1开始,所以更小。此外,如果存在任何2,我们永远不能以1结束,因为将它移动到前面的循环移位会产生更小的序列。该规则适用于确定第112,121和122号可能的决定。这解释了$2^4$完整树中所缺少的一切。
在手工处理较大的问题时,这种方法可能会变得相当笨拙。试着用它来坐六英尺长的摩天轮。另一个问题将四个相同的球体粘在一起,使其中三个位于等边三角形的顶点,第四个位于中心。也就是说,球体的中心位于一个平面上,其中三个中心位于等边三角形的四个角上,而第四个中心位于正中央。因此,如果将球体围绕三个轴中的任何一个翻转,或者围绕穿过中心球体中心并垂直于中心平面的轴旋转120度,球体排列在外观上保持不变。给自己画一幅图来说明这一点——养成画画的习惯来帮助想象这样的问题是非常有用的。我们有四个相同的小红球和四个相同的小绿球。球被放置在球中,使每个球恰好包含两个球。有多少种可能的安排?
计算可以借助决策树来完成。第一个决定可以是放置在中心球中的红球的数量。如果中间的球没有红球,那么必须在中间的球和外围的球上各放两个绿球。这两个在外围的球体可以被放置在同一个球体中,也可以被放在不同的球体中。按照这种方式进行,我们可以构造一个决策树。您应该这样做,并验证有六种不同的排列是可能的。
数学代写|组合学代写Combinatorics代考|Counting Structures with Symmetries
我们一直在松散地使用“等效”,而没有说明它的含义。由于模棱两可的术语容易导致错误,我们应该定义它。
集合$S$上的等价关系是$S$的一个分区。我们说$s, t \in S$是等价的当且仅当它们属于分区的同一块。如果符号$\sim$表示等价关系,那么我们写$s \sim t$表示$s$和$t$是等价的。等价类是$S$的一个子集,由集合中与某个对象等价的所有对象组成;也就是说,等价类是分区的一个块。
回到我们的循环序列问题,等价类是什么样的?首先,111111本身属于一个类,因为所有的旋转都会给我们相同的序列。同样,222222本身也在一个类中。序列${121212,212121}$是第三个等价类。序列112112和122122属于不同的等价类,每个等价类包含3个序列。到目前为止,我们有5个等价类,总共包含10个序列。剩下的$2^6-10=54$序列呢?结果表明,它们可分为9个等价类,每类6个序列。因此有$5+9=14$等价类;也就是说,这个循环数列问题的答案是14。
这种方法不适用于较大的问题。你可以试着做12个由12 3组成的长圆形序列,结果是44,368。伯恩赛德引理让我们更容易解决这类问题。为了说明和证明它,我们需要一些关于对称性的观察。
在我们的问题中,对称性是摩天轮通过$0^{\circ}, 60^{\circ}, 120^{\circ}, 180^{\circ}$$240^{\circ}$和$300^{\circ}$的旋转。这对应于分别从第一个、第二个、$\ldots$和第六个位置开始循环读取一个序列。设$S$为所有六个长0和1序列的集合。这六种对称对应着$S$的六种排列方式。例如,如果$g_i$是从$i+1$位置开始读取的排列,那么是$g_1(111122)=111221$和$g_3(111122)=122111$。注意,对于所有$x$,都使用$g_0(x)=x$。或者,我们可以认为$g_i$是将序列向左“循环”移动$i$个位置。集合$G=\left{g_0, \ldots, g_5\right}$有一些重要的性质,即
(G-1)有一个$e \in G$使得$e(x)=x$适用于所有$x \in S$。
(G-2)如果$f \in G$,则$f$的逆存在且$f^{-1} \in G$。
(G-3)如果$f, g \in G$,则组成$f g$在$G$中。

微观经济学代写
微观经济学是主流经济学的一个分支,研究个人和企业在做出有关稀缺资源分配的决策时的行为以及这些个人和企业之间的相互作用。my-assignmentexpert™ 为您的留学生涯保驾护航 在数学Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在图论代写Graph Theory代写方面经验极为丰富,各种图论代写Graph Theory相关的作业也就用不着 说。
线性代数代写
线性代数是数学的一个分支,涉及线性方程,如:线性图,如:以及它们在向量空间和通过矩阵的表示。线性代数是几乎所有数学领域的核心。
博弈论代写
现代博弈论始于约翰-冯-诺伊曼(John von Neumann)提出的两人零和博弈中的混合策略均衡的观点及其证明。冯-诺依曼的原始证明使用了关于连续映射到紧凑凸集的布劳威尔定点定理,这成为博弈论和数学经济学的标准方法。在他的论文之后,1944年,他与奥斯卡-莫根斯特恩(Oskar Morgenstern)共同撰写了《游戏和经济行为理论》一书,该书考虑了几个参与者的合作游戏。这本书的第二版提供了预期效用的公理理论,使数理统计学家和经济学家能够处理不确定性下的决策。
微积分代写
微积分,最初被称为无穷小微积分或 “无穷小的微积分”,是对连续变化的数学研究,就像几何学是对形状的研究,而代数是对算术运算的概括研究一样。
它有两个主要分支,微分和积分;微分涉及瞬时变化率和曲线的斜率,而积分涉及数量的累积,以及曲线下或曲线之间的面积。这两个分支通过微积分的基本定理相互联系,它们利用了无限序列和无限级数收敛到一个明确定义的极限的基本概念 。
计量经济学代写
什么是计量经济学?
计量经济学是统计学和数学模型的定量应用,使用数据来发展理论或测试经济学中的现有假设,并根据历史数据预测未来趋势。它对现实世界的数据进行统计试验,然后将结果与被测试的理论进行比较和对比。
根据你是对测试现有理论感兴趣,还是对利用现有数据在这些观察的基础上提出新的假设感兴趣,计量经济学可以细分为两大类:理论和应用。那些经常从事这种实践的人通常被称为计量经济学家。
Matlab代写
MATLAB 是一种用于技术计算的高性能语言。它将计算、可视化和编程集成在一个易于使用的环境中,其中问题和解决方案以熟悉的数学符号表示。典型用途包括:数学和计算算法开发建模、仿真和原型制作数据分析、探索和可视化科学和工程图形应用程序开发,包括图形用户界面构建MATLAB 是一个交互式系统,其基本数据元素是一个不需要维度的数组。这使您可以解决许多技术计算问题,尤其是那些具有矩阵和向量公式的问题,而只需用 C 或 Fortran 等标量非交互式语言编写程序所需的时间的一小部分。MATLAB 名称代表矩阵实验室。MATLAB 最初的编写目的是提供对由 LINPACK 和 EISPACK 项目开发的矩阵软件的轻松访问,这两个项目共同代表了矩阵计算软件的最新技术。MATLAB 经过多年的发展,得到了许多用户的投入。在大学环境中,它是数学、工程和科学入门和高级课程的标准教学工具。在工业领域,MATLAB 是高效研究、开发和分析的首选工具。MATLAB 具有一系列称为工具箱的特定于应用程序的解决方案。对于大多数 MATLAB 用户来说非常重要,工具箱允许您学习和应用专业技术。工具箱是 MATLAB 函数(M 文件)的综合集合,可扩展 MATLAB 环境以解决特定类别的问题。可用工具箱的领域包括信号处理、控制系统、神经网络、模糊逻辑、小波、仿真等。