MY-ASSIGNMENTEXPERT™可以为您提供sydney MATH3063 Ordinary Differential Equations常微分方程的代写代考和辅导服务!
这是悉尼大学 常微分方程课程的代写成功案例。

MATH3063课程简介
This unit of study is an introduction to the theory of systems of ordinary differential equations. Such systems model many types of phenomena in engineering, biology and the physical sciences. The emphasis will not be on finding explicit solutions, but instead on the qualitative features of these systems, such as stability, instability and oscillatory behaviour. The aim is to develop a good geometrical intuition into the behaviour of solutions to such systems. Some background in linear algebra, and familiarity with concepts such as limits and continuity, will be assumed. The applications in this unit are from biology, physics, chemistry, and engineering, including population dynamics, epidemics, chemical reactions, and simple mechanical systems.
Prerequisites
At the completion of this unit, you should be able to:
- LO1. explain the principle of linear approximations to nonlinear systems and use this to analyse system behaviour close to steady states
- LO2. synthesise graphical information from nullclines and flow to construct qualitative phase plane solutions to problems in nonlinear systems
- LO3. demonstrate knowledge of the theory of existence and uniqueness, and the determination of stability of solutions of ordinary differential equations, including special cases such as Hamiltonian and gradient systems.
- LO4. interpret model results and evaluate and explain the limitations of models in representing real systems
- LO5. demonstrate a broad understanding of the role of basic bifurcations in nonlinear systems and evaluate the effect of parameter variation on observed model behaviour
- LO6. apply mathematical theory in novel and diverse applications.
MATH3063 Ordinary Differential Equations HELP(EXAM HELP, ONLINE TUTOR)
Use Newton’s laws to derive the equations of motion for a frictionless pendulum. Suppose that the pendulum moves close to the surface of the Earth, so that the acceleration of the gradational field can be assumed constant.
Rewrite the equation as a system of first order differential equation, i.e. as a vector field. Sketch the vector field, first by hand, and then using a computer program. What kinds of behavior do you expect from the pendulum? Compare this to what you see in the vector filed drawings.
Repeat the problem assuming that the pendulum is subject to the force of friction.
Suppose that the initial displacement of the pendulum is “small” compared to the length of the pendulum. Simplify the equations of motion accounting this the “smallness” assumption. Now justify Galileo’s observation that the period of a pendulum depends only on the length of the arm (and for example not the angle of maximum displacement).
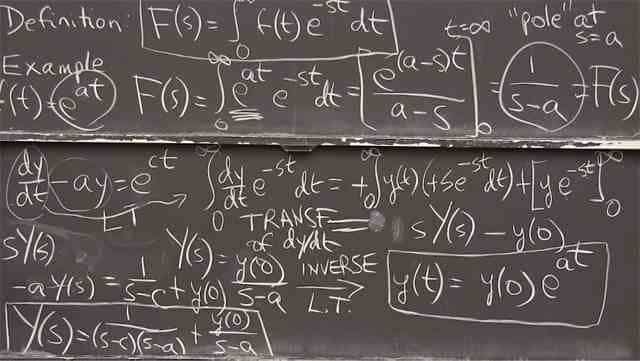
(Meet the van der Pol equation) The Van der Pol equation is given by the second-order differential equation
$$
\frac{d^2}{d t^2} x(t)-\mu\left(1-x(t)^2\right) \frac{d}{d t} x(t)+x(t)=0,
$$
and describes the voltage versus time behavior in a certain electrical circuit consisting of resistors, inductors, capacitors, a vacuum tube (triode), and a constant external voltage source.
Re-write the system as a vector field.
Take $\mu=1$ and $\left(x_0, y_0\right)=(-1,1)$. Use Euler’s method, a hand held calculator, and pen and paper to computer 10 steps on numerical integration with $h=0.1$,
Write a computer program which can numerically solve the equation (you can use or modify one of mine). Take $\mu=0.1$. Pick 25 initial conditions in the plane and integrate them “for a long time”. Describe what happens.
Same problem as above but with $\mu=0.25$.
Now repeat with $\mu=0.5, \ldots, \mu=10$, taking at least 5 different values of $\mu$. Describe what happens.
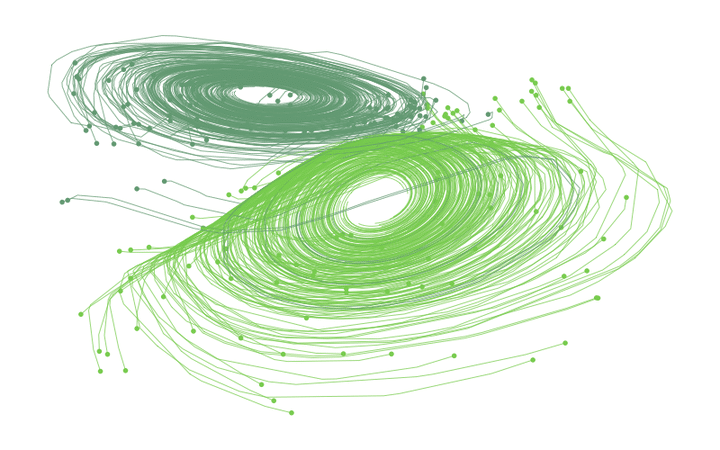
(Meet the Lorenz equations) Consider the system of three nonlinear coupled first order ordinary differential equations
$$
\begin{aligned}
& \frac{d}{d t} x=\sigma(y-x) \
& \frac{d}{d t} y=x(\rho-z)-y \
& \frac{d}{d t} z=x y-\beta z
\end{aligned}
$$
These are called the Lorenz equations, and they were derived by the Meteorologist Edward Lorenz in the early 1960’s as a simple model of weather (more precisely as a model of a a pair of coupled convection cells in the atmosphere). In the model $\sigma, \beta, \rho>0$ are positive real parameters.
- Take $\sigma=10, \beta=8 / 3$ and $\rho=0.75$, and $\left(x_0, y_0, z_0\right)=(0,0,1)$. Use Euler’s method, a hand held calculator, and pen and paper to computer 10 steps on numerical integration with $h=0.1$,
- Write a program (or use one of mine) which can simulate solutions for the Lorenz equations. Take $\sigma=10, \beta=8 / 3$ and $\rho=0.75$. Choose 25 different initial conditions and integrate them for a “long time”. Describe what happens.
- Take $\sigma=10, \beta=8 / 3$ and $\rho=2$. Choose 25 different initial conditions and integrate them for a long time. Describe what happens. Repeat this experiment with $\rho=$ $5,8,10,12$.
- Repeat with $\rho=14,18,20,22,24$.
- Repeat with $\rho=28$. This is the so called “classic parameter” for the Lorenz system.
- Explore what happens for ten different values of $\rho$ between $\rho=145.96$ and $\rho=166.07$.
(Strange attractor scavenger hunt) Write computer programs (by modifying the Lorenz code I gave you) which reproduce all 7 of the strange attractors found on the posters in the second floor hall way, outside the main math office in the FAU Science Building.

MY-ASSIGNMENTEXPERT™可以为您提供SYDNEY MATH3063 ORDINARY DIFFERENTIAL EQUATIONS常微分方程的代写代考和辅导服务!