如果你也在 怎样代写组合学Combinatorics 这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。组合学Combinatorics是数学的一个领域,主要涉及计数(作为获得结果的手段和目的)以及有限结构的某些属性。主要涉及计数,作为获得结果的手段和目的,以及有限结构的某些属性。它与数学的许多其他领域密切相关,有许多应用,从逻辑学到统计物理学,从进化生物学到计算机科学。
组合学Combinatorics因其解决的问题的广泛性而闻名。组合问题出现在纯数学的许多领域,特别是在代数、概率论、拓扑学和几何学中,以及在其许多应用领域。许多组合问题在历史上被孤立地考虑,对某个数学背景下出现的问题给出一个临时性的解决方案。然而,在二十世纪后期,强大而普遍的理论方法被开发出来,使组合学本身成为一个独立的数学分支。组合学最古老和最容易理解的部分之一是图论,它本身与其他领域有许多自然联系。在计算机科学中,组合学经常被用来获得算法分析中的公式和估计。
组合学Combinatorics代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。 最高质量的组合学Combinatorics作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此组合学Combinatorics作业代写的价格不固定。通常在专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
同学们在留学期间,都对各式各样的作业考试很是头疼,如果你无从下手,不如考虑my-assignmentexpert™!
my-assignmentexpert™提供最专业的一站式服务:Essay代写,Dissertation代写,Assignment代写,Paper代写,Proposal代写,Proposal代写,Literature Review代写,Online Course,Exam代考等等。my-assignmentexpert™专注为留学生提供Essay代写服务,拥有各个专业的博硕教师团队帮您代写,免费修改及辅导,保证成果完成的效率和质量。同时有多家检测平台帐号,包括Turnitin高级账户,检测论文不会留痕,写好后检测修改,放心可靠,经得起任何考验!
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
我们在数学Mathematics代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在组合学Combinatorics代写方面经验极为丰富,各种组合学Combinatorics相关的作业也就用不着 说。

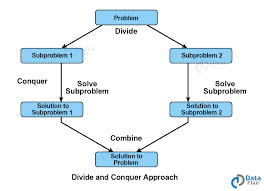
数学代写|组合学代写Combinatorics代考|Divide and Conquer
In its narrowest sense, divide and conquer refers to the division of a problem into a few smaller problems that are of the same kind as the original problem and so can be handled by a recursive method. We’ve seen binary insertion, Quicksort and merge sorting as examples of this. In a broader sense, divide and conquer refers to any method that divides a problem into a few simpler problems.
Heapsort illustrates this broader definition.
The broad divide and conquer technique is important for dealing with most complex situations. Delegation of responsibility is an application in everyday life. Scientific investigation often employs divide and conquer. In computer science it appears in both the design and implementation of algorithms, where it is referred to by such terms as “top-down programming,” “structured programming,” “object oriented programming” and “modularity.” Properly used, these are techniques for efficiently creating and implementing understandable, correct and flexible programs.
What tools are available for applying divide and conquer to smaller problems? For example, how might one discover the algorithms we’ve discussed in this text? An algorithm that has a nonrecursive
nature doesn’t seem to fit any general rules; for example, all we can recommend for discovering something like Heapsort is inspiration. You can cultivate inspiration by being familiar with a variety of ideas and by trying to look at problems in novel ways. We can say a bit more about trying to discover recursive algorithms; that is, algorithms that we use divide and conquer in its narrowest sense. Suppose the data is such that it is possible to split it into a few large blocks. You can ask yourself if anything is accomplished by solving the original problem on the separate blocks and then exploiting it. We’ll see how this works by first reviewing earlier material and then move on to some new problems.
数学代写|组合学代写Combinatorics代考|Limits on Speed
Suppose someone comes to you with two sorting algorithms and asks you which is faster. How do you answer him? Unless he has actual code for a particular machine, you couldn’t obtain actual times. However, since comparisons are the heart of sorting, we could ask: “How many comparisons does this algorithm make in the process of sorting?” We could then suggest that the algorithm that required less comparisons was the faster of the two. There are some factors that make this only a rough estimate:
• We did not include relocation overhead—somehow items must be repositioned to obtain the sorted list.
• We did not include miscellaneous overhead such as initialization and subroutine calls. We will ignore such problems and just look at the number of comparisons. Even so, there are problems:
• Using the parallel processing capabilities of supercomputers or special purpose devices will throw time estimates off because more than one comparison can be done at a time. The amount of parallelism that is possible can vary from algorithm to algorithm.
• The number of comparisons needed may vary greatly, depending on the order of the items in the unsorted list. We’ll ignore these factors in the discussion, except for parallelism in sorting networks, where it is of major importance. Besides all these problems with estimating running time, there is another problem: Running time is not the only standard that can be used to decide how good an algorithm is. Other important questions include
• How long will it take to get an error free program running?
• How much storage space will the algorithm require?
We’ll ignore these issues and focus on running time.
Let C(n) be the number of comparisons that an algorithm requires to sort n items. “Foul!” cries a careful reader. “You pointed out that the time a sort takes depends on the original order of the list and now you’re talking about this number C(n) as if that weren’t the case.” True. We should specify C(n) more carefully. It’s reasonable to consider two measures of speed:
• Worst case: C(n) = WC(n), the greatest number of comparisons the algorithm requires to sort n items. This is important where the results of a sort are needed quickly.
• Average: C(n) = AC(n), the average number of comparisons the algorithm requires to sort n items. This is important when we are doing many sorts and want to minimize overall computer usage.
组合学代写
数学代写|组合学代写Combinatorics代考|Divide and Conquer
从最狭义上讲,分治法是指将一个问题分成几个较小的问题,这些问题与原始问题具有相同的类型,因此可以用递归方法处理。我们已经见过二进制插入,快速排序和归并排序的例子。从更广泛的意义上讲,分治法指的是将一个问题分成几个更简单的问题的任何方法。
堆排序说明了这个更广泛的定义。
广泛的分治法对于处理大多数复杂的情况很重要。委派责任是日常生活中的一种应用。科学调查经常采用分而治之的方法。在计算机科学中,它出现在算法的设计和实现中,被称为“自顶向下编程”、“结构化编程编程”、“面向对象编程”和“模块化”。如果使用得当,这些技术可以有效地创建和实现可理解的、正确的和灵活的程序。
有哪些工具可以应用分治法解决较小的问题?例如,如何发现我们在本文中讨论的算法?具有非递归
性质的算法似乎不符合任何一般规则;例如,对于发现像堆排序这样的东西,我们所能推荐的只是灵感。你可以通过熟悉各种各样的想法和尝试用新颖的方式看待问题来培养灵感。关于发现递归算法,我们可以多说一点;也就是我们用分治法的最狭义的算法。假设数据是这样的,可以将其分成几个大块。您可以问自己,通过在单独的块上解决原始问题,然后利用它,是否完成了任何事情。我们将通过先复习以前的材料来了解它是如何工作的,然后再讨论一些新的问题。
数学代写|组合学代写Combinatorics代考|Limits on Speed
假设有人带着两种排序算法来问你哪一种更快。你怎么回答他?除非他有特定机器的实际代码,否则您无法获得实际时间。然而,由于比较是排序的核心,我们可以问:“这个算法在排序过程中进行了多少次比较?”然后我们可以认为,需要较少比较的算法比这两种算法更快。有一些因素使得这只是一个粗略的估计:
•我们没有包括重定位开销——为了获得排序列表,某些项必须重新定位。
•我们没有包括诸如初始化和子程序调用之类的杂项开销。我们将忽略这些问题,只看比较的次数。即便如此,也存在一些问题:使用超级计算机或特殊用途设备的并行处理能力会使时间估计偏离,因为一次可以进行多个比较。可能的并行量因算法而异。
•所需的比较次数可能变化很大,这取决于未排序列表中元素的顺序。在讨论中,我们将忽略这些因素,除了排序网络中的并行性,这是非常重要的。除了所有这些估计运行时间的问题之外,还有另一个问题:运行时间并不是用来决定算法好坏的唯一标准。其他重要的问题包括
•运行一个没有错误的程序需要多长时间?
•算法需要多少存储空间?
我们将忽略这些问题,专注于运行时间。
设C(n)为算法对n项排序所需的比较次数。“犯规!一位细心的读者喊道。“你指出排序所花费的时间取决于列表的原始顺序,而现在你谈论这个数字C(n),好像事实并非如此。”真实的。我们应该更仔细地指定C(n)考虑两种速度度量是合理的:
•最坏情况:C(n) = WC(n),算法排序n项所需的最大比较次数。在需要快速排序结果的情况下,这一点很重要。
•Average: C(n) = AC(n),算法对n个项目排序所需的平均比较次数。当我们要进行多种排序并希望最大限度地减少总体计算机使用量时,这一点很重要。

微观经济学代写
微观经济学是主流经济学的一个分支,研究个人和企业在做出有关稀缺资源分配的决策时的行为以及这些个人和企业之间的相互作用。my-assignmentexpert™ 为您的留学生涯保驾护航 在数学Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在图论代写Graph Theory代写方面经验极为丰富,各种图论代写Graph Theory相关的作业也就用不着 说。
线性代数代写
线性代数是数学的一个分支,涉及线性方程,如:线性图,如:以及它们在向量空间和通过矩阵的表示。线性代数是几乎所有数学领域的核心。
博弈论代写
现代博弈论始于约翰-冯-诺伊曼(John von Neumann)提出的两人零和博弈中的混合策略均衡的观点及其证明。冯-诺依曼的原始证明使用了关于连续映射到紧凑凸集的布劳威尔定点定理,这成为博弈论和数学经济学的标准方法。在他的论文之后,1944年,他与奥斯卡-莫根斯特恩(Oskar Morgenstern)共同撰写了《游戏和经济行为理论》一书,该书考虑了几个参与者的合作游戏。这本书的第二版提供了预期效用的公理理论,使数理统计学家和经济学家能够处理不确定性下的决策。
微积分代写
微积分,最初被称为无穷小微积分或 “无穷小的微积分”,是对连续变化的数学研究,就像几何学是对形状的研究,而代数是对算术运算的概括研究一样。
它有两个主要分支,微分和积分;微分涉及瞬时变化率和曲线的斜率,而积分涉及数量的累积,以及曲线下或曲线之间的面积。这两个分支通过微积分的基本定理相互联系,它们利用了无限序列和无限级数收敛到一个明确定义的极限的基本概念 。
计量经济学代写
什么是计量经济学?
计量经济学是统计学和数学模型的定量应用,使用数据来发展理论或测试经济学中的现有假设,并根据历史数据预测未来趋势。它对现实世界的数据进行统计试验,然后将结果与被测试的理论进行比较和对比。
根据你是对测试现有理论感兴趣,还是对利用现有数据在这些观察的基础上提出新的假设感兴趣,计量经济学可以细分为两大类:理论和应用。那些经常从事这种实践的人通常被称为计量经济学家。
Matlab代写
MATLAB 是一种用于技术计算的高性能语言。它将计算、可视化和编程集成在一个易于使用的环境中,其中问题和解决方案以熟悉的数学符号表示。典型用途包括:数学和计算算法开发建模、仿真和原型制作数据分析、探索和可视化科学和工程图形应用程序开发,包括图形用户界面构建MATLAB 是一个交互式系统,其基本数据元素是一个不需要维度的数组。这使您可以解决许多技术计算问题,尤其是那些具有矩阵和向量公式的问题,而只需用 C 或 Fortran 等标量非交互式语言编写程序所需的时间的一小部分。MATLAB 名称代表矩阵实验室。MATLAB 最初的编写目的是提供对由 LINPACK 和 EISPACK 项目开发的矩阵软件的轻松访问,这两个项目共同代表了矩阵计算软件的最新技术。MATLAB 经过多年的发展,得到了许多用户的投入。在大学环境中,它是数学、工程和科学入门和高级课程的标准教学工具。在工业领域,MATLAB 是高效研究、开发和分析的首选工具。MATLAB 具有一系列称为工具箱的特定于应用程序的解决方案。对于大多数 MATLAB 用户来说非常重要,工具箱允许您学习和应用专业技术。工具箱是 MATLAB 函数(M 文件)的综合集合,可扩展 MATLAB 环境以解决特定类别的问题。可用工具箱的领域包括信号处理、控制系统、神经网络、模糊逻辑、小波、仿真等。