数学代写|MATH2922 Modern Algebra

MY-ASSIGNMENTEXPERT™可以为您提供sydney MATH2922 Modern Algebra现代代数课程的代写代考和辅导服务！

这是悉尼大学现代代数课程的代写成功案例。

MATH2922课程简介

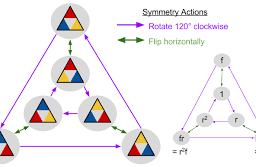
Linear and abstract algebra is one of the cornerstones of mathematics and it is at the heart of many applications of mathematics and statistics in the sciences and engineering. This unit is an advanced version of MATH2022, with more emphasis on the underlying concepts and on mathematical rigour. This unit investigates and explores properties of vector spaces, matrices and linear transformations, developing general principles relating to the solution sets of homogeneous and inhomogeneous linear equations, including differential equations. Linear independence is introduced as a way of understanding and solving linear systems of arbitrary dimension. Linear operators on real spaces are investigated, paying particular attention to the geometrical significance of eigenvalues and eigenvectors, extending ideas from first year linear algebra. To better understand symmetry, matrix and permutation groups are introduced and used to motivate the study of abstract groups theory. The unit culminates in studying inner spaces, quadratic forms and normal forms of matrices together with their applications to problems both in mathematics and in the sciences and engineering.

Prerequisites 

At the completion of this unit, you should be able to:

• LO1. appreciate the basic concepts and problems of linear algebra and be able to apply linear algebra to solve problems in mathematics, science and engineering
• LO2. understand the definitions of fields and vector spaces and be able to perform calculations in real and complex vector spaces, both algebraically and geometrically
• LO3. determine if a system of equations is consistent and find its general solution
• LO4. compute the rank of a matrix and understand how the rank of a matrix relates to the solution set of a corresponding system of linear equations
• LO5. compute the eigenvalues, eigenvectors, minimal polynomials and normal forms for linear transformations
• LO6. use the definition and properties of linear transformations and matrices of linear transformations and change of basis, including kernel, range and isomorphism
• LO7. compute inner products and determine orthogonality on vector spaces, including Gram-Schmidt orthogonalisation
• LO8. identify self-adjoint transformations and apply the spectral theorem and orthogonal decomposition of inner product spaces, and the Jordan canonical form, to solving systems of ordinary differential equations
• LO9. calculate the exponential of a matrix and use it to solve a linear system of ordinary differential equations with constant coefficients
• LO10. identify special properties of a matrix, such as symmetric of Hermitian, positive definite, etc., and use this information to facilitate the calculation of matrix characteristics
• LO11. demonstrate accurate and efficient use of advanced algebraic techniques and the capacity for mathematical reasoning through analysing, proving and explaining concepts from advanced algebra
• LO12. apply problem-solving using advanced algebraic techniques applied to diverse situations in physics, engineering and other mathematical contexts

MATH2922 Modern Algebra HELP（EXAM HELP， ONLINE TUTOR）

Since $a_1, a_2$ are units, there are nonzero $a_1^{\prime}, a_2^{\prime} \in R$ with $a_1 a_1^{\prime}=1=a_2 a_2^{\prime}$. Now we have $\left(a_1 a_2\right)\left(a_1^{\prime} a_2^{\prime}\right)=\left(a_1 a_1^{\prime}\right)\left(a_2 a_2^{\prime}\right)=1$, so $a_1 a_2$ is a unit. Suppose now that $a_1 b_1$ were a unit. Then there would be some nonzero $c \in R$ with $a_1 b_1 c=1$. But now $b_1\left(a_1 c\right)=1$, so $b_1$ is a unit, which contradicts hypothesis. Hence $a_1 b_1$ is a nonunit. The proof for $b_1 b_2$ is similar; if it were a unit then for some $c \in R$ we would have $b_1 b_2 c=1$, so $b_1\left(b_2 c\right)=1$, so $b_1$ would be a unit. Since it’s not, $b_1 b_2$ is a nonunit.

Since $a_1$ is a zero divisor, there is some $a_1^{\prime}$ with $a_1 a_1^{\prime}=0$. Hence $\left(a_1 b_1\right) a_1^{\prime}=\left(a_1 a_1^{\prime}\right) b_1=$ $0 b_1=0$, and also $a_1 b_1 \neq 0$ (else $b_1$ would be a zero divisor), so $a_1 b_1$ is a zero divisor. Note that $a_1 a_2$ might NOT be a zero divisor, because $a_1 a_2$ might be zero, which is not a zero divisor. Lastly, suppose that $b_1 b_2$ were a zero divisor. Then there would be some nonzero $c$ with $\left(b_1 b_2\right) c=0$. But then $b_1\left(b_2 c\right)=0$. Since $b_2$ is not a zero divisor, then $b_2 c$ is not zero, so that makes $b_1$ a zero divisor. This is a contradiction, so $b_1 b_2$ is not a zero divisor.

We have $0_R \in S$, because that’s part of the definition of subring. Also, for each $s \in S$, $0_R+s=s$, because $0_R$ is neutral in $R$. Hence $0_R$ is additively neutral in $S$; since this element is unique, in fact $0_R=0_S$. Suppose now that $a, b \in S$ are neither $0_S$, and also $a b=0_S$. Well, since $0_R=0_S$, we have $a, b \in R$; also neither is $0_R$, and $a b=0_R$. So if $a$ is a zero divisor in $S$, then it is a zero divisor in $R$.

Suppose that $a$ is a unit in $S$; then neither is $0_S$ and there is some $b \in S$ with $a b=1_S$. But also $a, b \in R$, neither is $0_S=0_R$ (as proved in the previous problem), and $a b=1_S=1_R$. Hence $a$ is also a unit in $R$.

We’ve already seen such an example, namely $R=\mathbb{Z}_3 \times \mathbb{Z}_3$. This has $1_R=([1],[1])$. We now take the subring $S={([0],[0]),([0],[1]),([0],[2])}$. This has $1_S=([0],[1])$, which is actually a zero divisor in $R$. Now we can take $a=([0],[2]) \in S$, which has $a \odot a=1_S$, so $a$ is a unit in $S$. However $a$ is a zero divisor in $R\left(\right.$ e.g. $\left.a \odot([1],[0])=0_R\right)$ so is certainly not a unit there.

MY-ASSIGNMENTEXPERT™可以为您提供SYDNEY MATH2922 MODERN ALGEBRA现代代数课程的代写代考和辅导服务！