如果你也在 怎样代写金融衍生品Financial Derivatives 这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。金融衍生品Financial Derivatives对于广大公众来说,长期以来一直是所有金融工具中最神秘、最不为人所知的。虽然一些金融衍生品相当简单,但不可否认的是,其他衍生品相当复杂,需要大量的数学和统计知识才能完全理解。
金融衍生品Financial Derivatives通常被视为过于复杂而难以理解的金融工具,个人投资者往往会回避。与此同时,市场专业人士指出,金融衍生品交易目前约占整个另类资产市场的40%。
金融衍生品Financial Derivatives代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。最高质量的金融衍生品Financial Derivatives作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此金融衍生品Financial Derivatives作业代写的价格不固定。通常在专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
同学们在留学期间,都对各式各样的作业考试很是头疼,如果你无从下手,不如考虑my-assignmentexpert™!
my-assignmentexpert™提供最专业的一站式服务:Essay代写,Dissertation代写,Assignment代写,Paper代写,Proposal代写,Proposal代写,Literature Review代写,Online Course,Exam代考等等。my-assignmentexpert™专注为留学生提供Essay代写服务,拥有各个专业的博硕教师团队帮您代写,免费修改及辅导,保证成果完成的效率和质量。同时有多家检测平台帐号,包括Turnitin高级账户,检测论文不会留痕,写好
金融代写|金融衍生品代写Financial Derivatives代考|SEASONALITY IN SPOT AND FUTURES PRICES
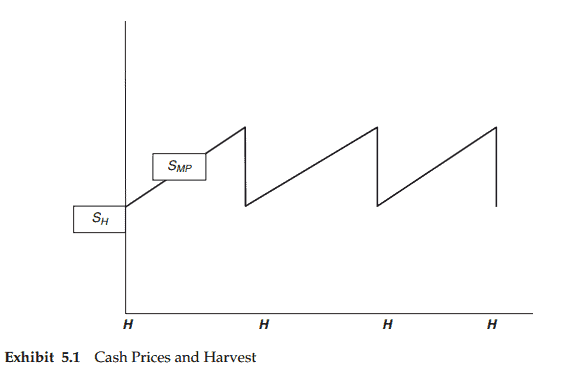
Commodities prices often exhibit patterns related to the seasonality of production or consumption. Many agricultural commodities are harvested once a year, so supply is fixed for that particular crop year while consumption occurs continuously. A typical seasonal price pattern is depicted in Exhibit 5.1. Prices serve as signals to the market as to how to allocate this crop inventory efficiently over the crop year. At harvest $(H)$, the spot price $\left(S_H\right)$ is at its lowest as the supply of the commodity peaks. After harvest, spot prices during the rest of the year are expected to be higher, reflecting the current spot price plus the cost of storage to that point in time. At the midpoint of the year, the spot is expected to be $S_{M P}$, which is also equal to $S_H$ plus the cost of storage from harvest up to the year’s midpoint. At the next harvest, new supply becomes available, and prices drop to reflect the increased inventory. Thus, the underlying seasonal price pattern is roughly sawtoothed, with a trough at harvest when supply is highest and ending in a peak at the end of the crop year when supply is at its lowest just before the next harvest.
The seasonal pattern, though predictable, is not necessarily evidence of market inefficiency. Seasonal price patterns are not as sharply defined as the discussion implies because crops vary in how successfully they can be stored. More important, seasonality will be obscured by factors that affect the spot price (and future expected spot prices) as the crop year unfolds. New information will shift the expected cash price pattern in Exhibit 5.1 up or down, and such changes in prices can overwhelm the seasonal pattern as the crop year develops. Inventory may also be carried from previous crop years, and this larger supply lowers expected spot prices and dampens the seasonal. Similarly, if more supply can be brought into the market during the crop year (from, e.g., a different climate zone), this will also disrupt the seasonal pattern.
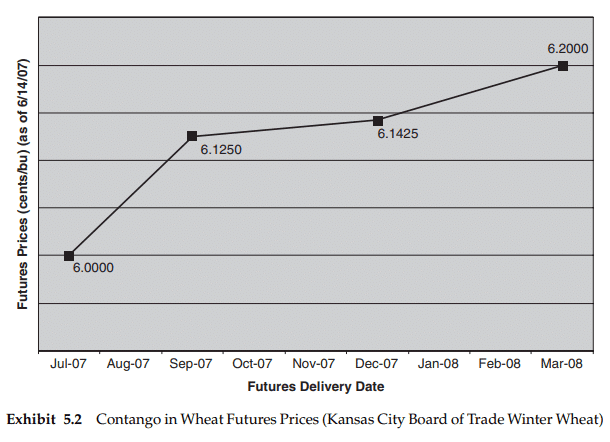
The futures price for delivery at time $t$ in the crop year will be equal to the expected spot price, which in turn is equal to the current spot price plus expected storage costs to period $t$. The futures curve, or futures strip, is the set of futures prices for different maturities, and it will be upward sloping (called contango) in this case. Exhibit 5.2 shows a strip of wheat futures in contango. The futures price for deferred delivery will be greater than the futures price for nearby delivery, reflecting the market’s estimate of the expected change in cash prices over that time. The difference between futures prices also reflects the return to storage; that is, what the market is willing to pay the holder of inventory to store the asset over the crop year. In this way, futures prices (expected cash prices) will allocate the crop inventory over the crop year. As long as the futures price/expected cash price covers the current cash price plus storage costs, the crop will be stored.
The basis of a futures contract is defined as the current spot price minus the futures price $(S P-F P)$. The basis reflects expected storage costs between today and the futures’ maturity. As the futures contract approaches maturity, storage costs approach zero, the futures price will converge to the cash price, and the basis will approach zero as well. The basis will thus also have a distinct seasonal component, and many producers use basis seasonality to make hedging decisions. However, because new information will alter the expected future cash price, the basis also fluctuates in response to information. Over time, changes in the basis will reflect both an expected change (storage costs), and an unexpected change (new information).
金融代写|金融衍生品代写Financial Derivatives代考|FUTURES PRICING
Two models have been proposed to explain the pricing of commodity futures. The theory of storage, discussed first, places major emphasis on the link between cash prices and forward prices, with forward prices reflecting physical storage costs and an imputed convenience yield arising from a mismatch between anticipated supply and consumption demand. The alternative theory of forward pricing, normal backwardation, places greater emphasis on risk management and the reward for risk.
Theory of Storage
The key differences between pricing futures on financial assets and commodities are the cost associated with carrying a commodity through time, and the cost of borrowing a physical asset in an arbitrage. For a commodity that is easily stored, such as gold, and with plentiful supply relative to demand, the futures contract will be priced at full carry over the spot price, and the differences between spot and futures price, or the futures prices for different maturities, reflect financing, physical storage, and insurance costs for that time period. For a full-carry commodity, the basic no-arbitrage framework is the same used to price forwards on financial assets. If the forward price $(F P)$ is greater than the spot price and carrying costs $[S P+c S P]$, then arbitrageurs can enter into a cash-and-carry arbitrage. They can obtain an arbitrage profit by selling the overpriced forward contract at $F P$, buying the spot commodity, financing the position, and paying the storage costs. At maturity, the arbitrage profit would be $F P-[S P+c S P]$. Thus, $[S P+c S P]$ is the upper bound for the forward price. The difference between forward prices for different maturity contracts can be expressed as a percentage $\left[\left(F P_2-F P_1\right) / F P_1\right]$, and this implied repo rate will be equal to the implied forward financing rate for that time period plus physical storage costs.
In the reverse cash and carry, if the $F P$ is less than $[S P+c S P]$, then arbitrageurs should buy the forward, sell short the gold, and invest the spot price cash flow at the financing rate until the forward contract matures. With a commodity, however, there is a charge to the arbitrageurs to borrow the physical asset, called the lease rate. The commodity owner demands a lease rate because a commodity does not necessarily increase in value as a financial asset is expected to do, and the commodity owner will want compensation for lending a real asset. While an investor in a financial asset expects to receive a positive, risk-adjusted rate of return, whether through dividends or capital gains, even if the asset is borrowed to enable a short sale, a commodity owner does not. Thus, the lower bound for a commodity futures price incorporates a lease rate for lending the physical asset.
In a full-carry commodity such as gold, the futures price will center around the equilibrium forward price in a band that reflects transaction costs, the ease of selling the commodity short (reflected in the lease rate), and other imperfections in the arbitrage transactions.
金融衍生品代写
金融代写|金融衍生品代写Financial Derivatives代考|SEASONALITY IN SPOT AND FUTURES PRICES
商品价格经常表现出与生产或消费的季节性有关的模式。许多农产品一年收获一次,因此在特定的作物年供应是固定的,而消费是持续的。典型的季节性价格模式如图5.1所示。价格向市场发出信号,说明如何在整个作物年有效地分配这些作物库存。在收获期$(H)$时,现货价格$\左(S_H\右)$处于最低水平,因为商品供应达到峰值。收获后,今年剩余时间的现货价格预计会更高,这反映了当前的现货价格加上当时的储存成本。在年中,现货价格预计为$S_{M P}$,这也等于$S_H$加上从收获到年中点的储存成本。在下一个收获季节,有了新的供应,价格就会下降,以反映库存的增加。因此,潜在的季节性价格模式大致呈锯齿状,在收获季节供应最高时出现低谷,在作物年度结束时达到峰值,此时供应在下一次收获前处于最低水平。
季节性模式虽然可以预测,但并不一定是市场效率低下的证据。季节性价格模式并不像讨论所暗示的那样泾渭分明,因为农作物的储存成功程度各不相同。更重要的是,随着作物年的展开,季节性将被影响现货价格(以及未来预期现货价格)的因素所掩盖。新的信息将使表5.1中的预期现金价格模式向上或向下变化,并且随着作物年的发展,这种价格变化可以压倒季节性模式。库存也可能来自以前的收成年份,这种较大的供应降低了预期的现货价格,抑制了季节性需求。同样,如果更多的供应可以在作物年进入市场(例如,从不同的气候带),这也将破坏季节性模式。
在作物年度$t$时刻交割的期货价格将等于预期现货价格,而现货价格又等于当前现货价格加上$t$期间的预期储存成本。期货曲线,或期货条,是不同期限的期货价格的集合,在这种情况下,它将向上倾斜(称为期货溢价)。图5.2显示小麦期货的期货溢价。延期交割的期货价格将高于近期交割的期货价格,这反映了市场对这段时间内现金价格预期变化的估计。期货价格的差异也反映了库存的回归;也就是说,市场愿意支付给库存持有者在整个作物年度储存资产的价格。通过这种方式,期货价格(预期现金价格)将在作物年度分配作物库存。只要期货价格/预期现金价格涵盖当前现金价格加上储存成本,作物就会被储存。
期货合约的基础定义为当前现货价格减去期货价格$(S P- f)$。这一基础反映了从现在到期货到期日之间的预期储存成本。随着期货合约临近到期,仓储成本趋近于零,期货价格向现金价格趋近,基差也趋近于零。因此,基差也将具有明显的季节性成分,许多生产商利用基差的季节性来做出对冲决策。然而,由于新的信息会改变预期的未来现金价格,基差也会随着信息的变化而波动。随着时间的推移,基础的变化将反映预期的变化(存储成本)和意外的变化(新信息)。
金融代写|金融衍生品代写Financial Derivatives代考|FUTURES PRICING
人们提出了两个模型来解释商品期货的定价。首先讨论的存储理论主要强调现金价格和远期价格之间的联系,远期价格反映了物理存储成本和预期供应与消费需求之间不匹配产生的估算便利收益。另一种远期定价理论,即正常的现货溢价,更强调风险管理和风险回报。
储存理论
金融资产期货和大宗商品期货定价的关键区别在于长期持有大宗商品的成本,以及在套利中借入实物资产的成本。对于易于储存的商品,如黄金,相对于需求而言供应充足,期货合约将以现货价格的全额结转定价,而现货和期货价格之间的差异,或不同期限的期货价格,反映了该时期的融资、实物储存和保险成本。对于全套利商品,基本的无套利框架与金融资产远期定价相同。如果远期价格大于现货价格,持有成本为[S P+c S P],那么套利者可以进行现金套利。他们可以通过以$ P$的价格卖出定价过高的远期合约,购买现货商品,为头寸融资,并支付存储成本来获得套利利润。到期时,套利利润为$F P-[S P+c S P]$。因此,$[S P+c S P]$是远期价格的上限。不同期限合约的远期价格之差可以用百分比$\left[\left(F P_2-F P_1\right) / F P_1\right]$来表示,这个隐含回购利率等于该时期的隐含远期融资利率加上物理存储成本。
在反向现金套利交易中,如果$F P$低于$[S P+c S P]$,那么套利者应该买入远期合约,卖空黄金,并以融资利率投资现货价格现金流,直到远期合约到期。然而,对于大宗商品,套利者在借入实物资产时要收取一笔费用,这被称为租赁利率。商品所有者要求租赁利率,因为商品并不一定会像金融资产那样增值,而商品所有者会因为借出实物资产而要求补偿。尽管金融资产的投资者期望获得正的、经风险调整后的回报率,无论是通过股息还是资本收益,即使资产是为了卖空而借入的,但大宗商品所有者却不会。因此,商品期货价格的下限包含了借出实物资产的租赁率。
在像黄金这样的全套利商品中,期货价格将以均衡远期价格为中心,该价格区间反映了交易成本、卖空商品的便利性(反映在租赁利率上)以及套利交易中的其他不完善之处。

金融代写|金融衍生品代写Financial Derivatives代考 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。
微观经济学代写
微观经济学是主流经济学的一个分支,研究个人和企业在做出有关稀缺资源分配的决策时的行为以及这些个人和企业之间的相互作用。my-assignmentexpert™ 为您的留学生涯保驾护航 在数学Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在图论代写Graph Theory代写方面经验极为丰富,各种图论代写Graph Theory相关的作业也就用不着 说。
线性代数代写
线性代数是数学的一个分支,涉及线性方程,如:线性图,如:以及它们在向量空间和通过矩阵的表示。线性代数是几乎所有数学领域的核心。
博弈论代写
现代博弈论始于约翰-冯-诺伊曼(John von Neumann)提出的两人零和博弈中的混合策略均衡的观点及其证明。冯-诺依曼的原始证明使用了关于连续映射到紧凑凸集的布劳威尔定点定理,这成为博弈论和数学经济学的标准方法。在他的论文之后,1944年,他与奥斯卡-莫根斯特恩(Oskar Morgenstern)共同撰写了《游戏和经济行为理论》一书,该书考虑了几个参与者的合作游戏。这本书的第二版提供了预期效用的公理理论,使数理统计学家和经济学家能够处理不确定性下的决策。
微积分代写
微积分,最初被称为无穷小微积分或 “无穷小的微积分”,是对连续变化的数学研究,就像几何学是对形状的研究,而代数是对算术运算的概括研究一样。
它有两个主要分支,微分和积分;微分涉及瞬时变化率和曲线的斜率,而积分涉及数量的累积,以及曲线下或曲线之间的面积。这两个分支通过微积分的基本定理相互联系,它们利用了无限序列和无限级数收敛到一个明确定义的极限的基本概念 。
计量经济学代写
什么是计量经济学?
计量经济学是统计学和数学模型的定量应用,使用数据来发展理论或测试经济学中的现有假设,并根据历史数据预测未来趋势。它对现实世界的数据进行统计试验,然后将结果与被测试的理论进行比较和对比。
根据你是对测试现有理论感兴趣,还是对利用现有数据在这些观察的基础上提出新的假设感兴趣,计量经济学可以细分为两大类:理论和应用。那些经常从事这种实践的人通常被称为计量经济学家。
Matlab代写
MATLAB 是一种用于技术计算的高性能语言。它将计算、可视化和编程集成在一个易于使用的环境中,其中问题和解决方案以熟悉的数学符号表示。典型用途包括:数学和计算算法开发建模、仿真和原型制作数据分析、探索和可视化科学和工程图形应用程序开发,包括图形用户界面构建MATLAB 是一个交互式系统,其基本数据元素是一个不需要维度的数组。这使您可以解决许多技术计算问题,尤其是那些具有矩阵和向量公式的问题,而只需用 C 或 Fortran 等标量非交互式语言编写程序所需的时间的一小部分。MATLAB 名称代表矩阵实验室。MATLAB 最初的编写目的是提供对由 LINPACK 和 EISPACK 项目开发的矩阵软件的轻松访问,这两个项目共同代表了矩阵计算软件的最新技术。MATLAB 经过多年的发展,得到了许多用户的投入。在大学环境中,它是数学、工程和科学入门和高级课程的标准教学工具。在工业领域,MATLAB 是高效研究、开发和分析的首选工具。MATLAB 具有一系列称为工具箱的特定于应用程序的解决方案。对于大多数 MATLAB 用户来说非常重要,工具箱允许您学习和应用专业技术。工具箱是 MATLAB 函数(M 文件)的综合集合,可扩展 MATLAB 环境以解决特定类别的问题。可用工具箱的领域包括信号处理、控制系统、神经网络、模糊逻辑、小波、仿真等。