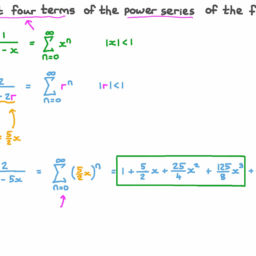
The following is a very important example known as the binomial series. It was discovered by Newton.
Example 8.6.1 Find a Taylor series for the function $(1+x)^{\alpha}$ centered at 0 valid for $|x|<1$.
Use Theorem 8.2.1 to do this. First note that if $y(x) \equiv(1+x)^{\alpha}$, then $y$ is a solution of the following initial value problem.
$$
y^{\prime}-\frac{\alpha}{(1+x)} y=0, y(0)=1 \text {. }
$$
Next it is necessary to observe there is only one solution to this initial value problem. To see this, multiply both sides of the differential equation in $8.16$ by $(1+x)^{-\alpha}$. When this is done, one obtains
$$
\frac{d}{d x}\left((1+x)^{-\alpha} y\right)=(1+x)^{-\alpha}\left(y^{\prime}-\frac{\alpha}{(1+x)} y\right)=0
$$
Therefore, from $8.17$, there must exist a constant, $C$, such that $(1+x)^{-\alpha} y=C .$ However, $y(0)=1$ and so it must be that $C=1$. Therefore, there is exactly one solution to the initial value problem in $8.16$ and it is $y(x)=(1+x)^{\alpha}$.
The strategy for finding the Taylor series of this function consists of finding a series which solves the initial value problem above. Let $y(x) \equiv \sum_{n=0}^{\infty} a_{n} x^{n}$ be a solution to 8.16. Of course it is not known at this time whether such a series exists. However, the process of finding it will demonstrate its existence. From Theorem $8.2 .1$ and the initial value problem, $(1+x) \sum_{n=0}^{\infty} a_{n} n x^{n-1}-\sum_{n=0}^{\infty} \alpha a_{n} x^{n}=0$ and so
$$
\sum_{n=1}^{\infty} a_{n} n x^{n-1}+\sum_{n=0}^{\infty} a_{n}(n-\alpha) x^{n}=0
$$
Changing the variable of summation in the first sum,
$$
\sum_{n=0}^{\infty} a_{n+1}(n+1) x^{n}+\sum_{n=0}^{\infty} a_{n}(n-\alpha) x^{n}=0
$$
下面是一个非常重要的例子,称为二项式级数。是牛顿发现的。
例 8.6.1 为函数 $(1+x)^{\alpha}$ 找到一个以 0 为中心的泰勒级数,对于 $|x|<1$ 有效。
使用定理 8.2.1 来做到这一点。首先注意如果$y(x)\equiv(1+x)^{\alpha}$,那么$y$是下面初值问题的一个解。
$$
y^{\prime}-\frac{\alpha}{(1+x)} y=0, y(0)=1 \text {. }
$$
接下来有必要观察这个初始值问题只有一个解决方案。要看到这一点,请将 $8.16$ 中微分方程的两边乘以 $(1+x)^{-\alpha}$。完成此操作后,您将获得
$$
\frac{d}{dx}\left((1+x)^{-\alpha} y\right)=(1+x)^{-\alpha}\left(y^{\prime}-\frac {\alpha}{(1+x)} y\right)=0
$$
因此,从 $8.17$ 开始,必定存在一个常数 $C$,使得 $(1+x)^{-\alpha} y=C .$ 但是,$y(0)=1$ 所以它必须是$C=1$。因此,$8.16$ 中的初值问题只有一个解,它是 $y(x)=(1+x)^{\alpha}$。
找到这个函数的泰勒级数的策略包括找到一个能解决上述初始值问题的级数。设 $y(x) \equiv \sum_{n=0}^{\infty} a_{n} x^{n}$ 是 8.16 的解。当然,目前还不知道这样的系列是否存在。但是,找到它的过程将证明它的存在。从定理 $8.2 .1$ 和初值问题,$(1+x) \sum_{n=0}^{\infty} a_{n} nx^{n-1}-\sum_{n=0}^ {\infty} \alpha a_{n} x^{n}=0$ 等等
$$
\sum_{n=1}^{\infty} a_{n} nx^{n-1}+\sum_{n=0}^{\infty} a_{n}(n-\alpha) x^{n }=0
$$
改变第一次求和中的求和变量,
$$
\sum_{n=0}^{\infty} a_{n+1}(n+1) x^{n}+\sum_{n=0}^{\infty} a_{n}(n-\alpha ) x^{n}=0
$$

微积分note Integer Multiples of Irrational Numbers 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。