如果你也在 怎样代写计量经济学Econometrics是将统计方法应用于经济数据,以赋予经济关系以经验内容。更确切地说,它是 “基于理论和观察的同步发展,通过适当的推理方法对实际经济现象进行定量分析”。 一本经济学入门教科书将计量经济学描述为允许经济学家 “从堆积如山的数据中筛选出简单的关系”。
计量经济学Econometrics的一个基本工具是多元线性回归模型。计量经济学理论使用统计理论和数理统计来评估和发展计量经济学方法。计量经济学家试图找到具有理想统计特性的估计器,包括无偏性、效率和一致性。应用计量经济学使用理论计量经济学和现实世界的数据来评估经济理论,开发计量经济学模型,分析经济历史和预测。
my-assignmentexpert™ 计量经济学Econometrics作业代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。my-assignmentexpert™, 最高质量的计量经济学Econometrics作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于统计Statistics作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此计量经济学Econometrics作业代写的价格不固定。通常在经济学专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
my-assignmentexpert™ 为您的留学生涯保驾护航 在经济Economy作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的计量经济学Econometrics代写服务。我们的专家在经济Economy代写方面经验极为丰富,各种计量经济学Econometrics相关的作业也就用不着 说。
我们提供的计量经济学Econometrics及其相关学科的代写,服务范围广, 其中包括但不限于:
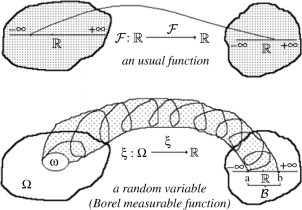
经济代写|计量经济学代写Econometrics代考|General Properties
In this section I will review the most important results regarding algebras, $\sigma-$ algebras, and probability measures.
Our first result is trivial:
Theorem 1.1: If an algebra contains only a finite number of sets, then it is a $\sigma$-algebra. Consequently, an algebra of subsets of a finite set $\Omega$ is a $\sigma$-algebra.
However, an algebra of subsets of an infinite set $\Omega$ is not necessarily a $\sigma$ algebra. A counterexample is the collection $\mathscr{F}{}$ of all subsets of $\Omega=(0,1]$ of the type $(a, b]$, where $a{}$ is an algebra. Next, let $p_{n}=\left[10^{n} \pi\right] / 10^{n}$ and $a_{n}=1 / p_{n}$, where $[x]$ means truncation to the nearest integer $\leq x$. Note that $p_{n} \uparrow \pi$; hence, $a_{n} \downarrow \pi^{-1}$ as $n \rightarrow \infty$. Then, for $n=1,2,3, \ldots,\left(a_{n}, 1\right] \in \mathscr{F}{}$, but $\cup{n=1}^{\infty}\left(a_{n}, 1\right]=\left(\pi^{-1}, 1\right] \notin \mathscr{F}{}$ because $\pi^{-1}$ is irrational. Thus, $\mathscr{F}{*}$ is not a $\sigma$-algebra.
Theorem 1.2: If $\mathscr{F}$ is an algebra, then $A, B \in \mathscr{F}$ implies $A \cap B \in \mathscr{F}$; hence, by induction, $A_{j} \in \mathscr{F}$ for $j=1, \ldots, n<\infty$ implies $\cap_{j=1}^{n} A_{j} \in \mathscr{F}$. A collection $\mathscr{F}$ of subsets of a nonempty set $\Omega$ is an algebra if it satisfies condition (1.5) and the condition that, for any pair $A, B \in \mathscr{F}, A \cap B \in \mathscr{F}$.
经济代写|计量经济学代写Econometrics代考|Borel Sets
An important special case of Definition $1.4$ is where $\Omega=\mathbb{R}$ and $\boldsymbol{c}$ is the collection of all open intervals:
$$
\boldsymbol{c}={(a, b): \forall a<b, a, b \in \mathbb{R}}
$$
Definition 1.5: The $\sigma$-algebra generated by the collection (1.18) of all open intervals in $\mathbb{R}$ is called the Euclidean Borel field, denoted by $\boldsymbol{B}$, and its members are called the Borel sets.
Note, however, that $\mathcal{B}$ can be defined in different ways because the $\sigma$-algebras generated by the collections of open intervals, closed intervals ${[a, b]: \forall a \leq$ $b, a, b \in \mathbb{R}}$ and half-open intervals ${(-\infty, a]: \forall a \in \mathbb{R}}$, respectively, are all the same! We show this for one case only:
Theorem 1.6: $\mathcal{B}=\sigma({(-\infty, a]: \forall a \in \mathbb{R}})$
Proof: Let
$$
\boldsymbol{\sigma}{}={(-\infty, a]: \forall a \in \mathbb{R}} . $$ is a $\sigma$-algebra containing $\boldsymbol{\sigma}$. But $\boldsymbol{B}=\sigma(\boldsymbol{\sigma})$ is the smallest $\sigma$-algebra containing $\boldsymbol{\varsigma}$; hence, $\boldsymbol{B}=\sigma(\boldsymbol{\sigma}) \subset \sigma\left(\boldsymbol{\sigma}{}\right)$.
To prove this, construct an arbitrary set $(a, b)$ in $(6$ out of countable unions or complements of sets in $\boldsymbol{c}{}$, or both, as follows: Let $A=$ $(-\infty, a]$ and $B=(-\infty, b]$, where $a{}$; hence, $A, \tilde{B} \in \sigma\left(\boldsymbol{\varsigma}{}\right)$, and thus $$ \sim(a, b]=(-\infty, a] \cup(b, \infty)=A \cup \tilde{B} \in \sigma\left(\boldsymbol{c}{}\right)
$$
This implies that $\sigma\left(\boldsymbol{c}{}\right)$ contains all sets of the type $(a, b]$; hence, $(a, b)=$ $\cup{n=1}^{\infty}(a, b-(b-a) / n] \in \sigma\left(\boldsymbol{\varsigma}{}\right)$. Thus, $\boldsymbol{c} \subset \sigma\left(\boldsymbol{\varsigma}{}\right)$. (b) If the collection $\boldsymbol{\sigma}{}$ defined by (1.19) is contained in $\boldsymbol{B}=\sigma(\boldsymbol{\sigma})$, then $\sigma\left(\boldsymbol{\sigma}^{}\right)$ is a $\sigma$-algebra containing $\boldsymbol{\sigma}{}$. But $\sigma\left(\boldsymbol{\sigma}{}\right)$ is the smallest $\sigma$-algebra containing $\boldsymbol{\varsigma}{}$; hence, $\sigma\left(\boldsymbol{\varsigma}_{*}\right) \subset \sigma(\boldsymbol{\varsigma})=\boldsymbol{\mathcal { B }}$.
To prove the latter, observe that, for $m=1,2, \ldots, A_{m}=\cup_{n=1}^{\infty}(a-$ $\left.n, a+m^{-1}\right)$ is a countable union of sets in (๘; hence, $\tilde{A}{m} \in \sigma(\boldsymbol{6})$, and consequently $(-\infty, a]=\cap{m=1}^{\infty} A_{m}=\sim\left(\cup_{m=1}^{\infty} \tilde{A}{m}\right) \in \sigma(\boldsymbol{\varsigma})$. Thus, $\boldsymbol{c}{*} \subset \sigma(\boldsymbol{c})=\mathbf{J}$.
We have shown now that $\boldsymbol{B}=\sigma(\boldsymbol{6}) \subset \sigma\left(\boldsymbol{\varsigma}{}\right)$ and $\sigma\left(\boldsymbol{\varsigma}{}\right) \subset \sigma(\boldsymbol{6})=\boldsymbol{B}$. Thus, $\boldsymbol{J}$ and $\sigma\left(\boldsymbol{\sigma}_{*}\right)$ are the same. Q.E.D. ${ }^{8}$
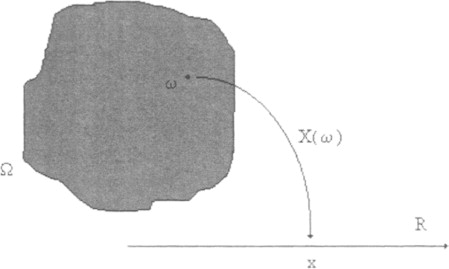
计量经济学代写
经济代写|计量经济学代写ECONOMETRICS代考|GENERAL PROPERTIES
在本节中,我将回顾有关代数的最重要的结果,σ−代数和概率测度。
我们的第一个结果是微不足道的:
定理 1.1:如果一个代数只包含有限数量的集合,那么它是σ-代数。因此,有限集子集的代数Ω是一个σ-代数。
然而,一个无限集的子集的代数Ω不一定是σ代数。一个反例是集合 $\mathscr{F}{}$ of all subsets of $\Omega=(0,1]$ of the type $(a, b]$, where $a{}$ is an algebra. Next, let $p_{n}=\left[10^{n} \pi\right] / 10^{n}$ and $a_{n}=1 / p_{n}$, where $[x]$ means truncation to the nearest integer $\leq x$. Note that $p_{n} \uparrow \pi$; hence, $a_{n} \downarrow \pi^{-1}$ as $n \rightarrow \infty$. Then, for $n=1,2,3, \ldots,\left(a_{n}, 1\right] \in \mathscr{F}{}$, but $\cup{n=1}^{\infty}\left(a_{n}, 1\right]=\left(\pi^{-1}, 1\right] \notin \mathscr{F}{}$ because $\pi^{-1}$ is irrational. Thus, $\mathscr{F}{*}$ is not a $\sigma$-algebra.
定理 1.2:如果F是代数,那么一种,乙∈F暗示一种∩乙∈F; 因此,通过归纳,一种j∈F为了j=1,…,n<∞暗示∩j=1n一种j∈F. 一个集合F非空集的子集Ω是一个代数,如果它满足条件1.5并且条件是,对于任何一对一种,乙∈F,一种∩乙∈F.
经济代写|计量经济学代写ECONOMETRICS代考|BOREL SETS
定义的一个重要特例1.4在哪Ω=R和C是所有开区间的集合:
C=(一种,b):∀一种<b,一种,b∈R
定义 1.5:σ- 集合生成的代数1.18在所有开区间中R称为欧几里得波雷尔场,记为乙,其成员称为 Borel 集。
但是请注意,乙可以用不同的方式定义,因为σ- 由开区间、闭区间的集合生成的代数[一种,b]:∀一种≤$$b,一种,b∈R和半开区间(−∞,一种]:∀一种∈R,分别都是一样的!我们仅针对一种情况展示这一点:
定理 1.6:乙=σ((−∞,一种]:∀一种∈R)
证明:设
$$
\boldsymbol{\sigma} {}={-\infty, a]: \forall a \in \mathbb{R}} 。$$ 是一个包含 $\boldsymbol{\sigma}$ 的 $\sigma$-代数。但是 $\boldsymbol{B}=\sigma(\boldsymbol{\sigma}-\infty, a]: \forall a \in \mathbb{R}} 。$$ 是一个包含 $\boldsymbol{\sigma}$ 的 $\sigma$-代数。但是 $\boldsymbol{B}=\sigma(\boldsymbol{\sigma}一世s吨H和s米一种ll和s吨\西格玛−一种lG和br一种C这n吨一种一世n一世nG\boldsymbol{\varsigma};H和nC和,\boldsymbol{B}=\sigmaσ\subset \sigma\left(\boldsymbol{\sigma}{ }\right)$。
为了证明这一点,构造一个任意集合(一种,b)(6$ out of countable unions or complements of sets in $\boldsymbol{c}{}$, or both, as follows: Let $A=$ $(-\infty, a]$ and $B=(-\infty, b]$, where $a{}$; hence, $A, \tilde{B} \in \sigma\left(\boldsymbol{\varsigma}{}\right)$, and thus $$ \sim(a, b]=(-\infty, a] \cup(b, \infty)=A \cup \tilde{B} \in \sigma\left(\boldsymbol{c}{}\right)
$$
This implies that $\sigma\left(\boldsymbol{c}{}\right)$ contains all sets of the type $(a, b]$; hence, $(a, b)=$ $\cup{n=1}^{\infty}(a, b-(b-a) / n] \in \sigma\left(\boldsymbol{\varsigma}{}\right)$. Thus, $\boldsymbol{c} \subset \sigma\left(\boldsymbol{\varsigma}{}\right)$. (b) If the collection $\boldsymbol{\sigma}{}$ defined by (1.19) is contained in $\boldsymbol{B}=\sigma(\boldsymbol{\sigma})$, then $\sigma\left(\boldsymbol{\sigma}^{}\right)$ is a $\sigma$-algebra containing $\boldsymbol{\sigma}{}$. But $\sigma\left(\boldsymbol{\sigma}{}\right)$ is the smallest $\sigma$-algebra containing $\boldsymbol{\varsigma}{}$; hence, $\sigma\left(\boldsymbol{\varsigma}_{*}\right) \subset \sigma(\boldsymbol{\varsigma})=\boldsymbol{\mathcal { B }}$.
为了证明后者,观察到,对于米=1,2,…,一种米=∪n=1∞(一种− n,一种+米−1)是 (๘) 中集合的可数并集;因此,$\tilde{A} {m} \in \sigma6,一种ndC这ns和q在和n吨l是(-\infty, a]=\cap {m=1}^{\infty} A_{m}=\sim\left(\cup_{m=1}^{\infty} \tilde{A} {m} \right) \in \sigmaε.吨H在s,\boldsymbol{c} {*} \subset \sigmaC= \ mathbf {J} $。
我们现在已经证明l{6}) \subset \sigma\left(\boldsymbol{\varsigma}{}\right)$ and $\sigma\left(\boldsymbol{\varsigma}{}\right) \subset \sigma(\boldsymbol{6})=\boldsymbol{B}$. Thus, $\boldsymbol{J}$ and $\sigma\left(\boldsymbol{\sigma}_{*}\right)$ are the same. Q.E.D. ${ }^{8}$

经济代写|计量经济学代写Econometrics代考 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。