如果你也在 怎样代写组合数学Combinatorial Mathematics这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。组合数学Combinatorial Mathematics是数学的一个领域,主要涉及计数,作为获得结果的手段和目的,以及有限结构的某些属性。它与数学的许多其他领域密切相关,有许多应用,从逻辑学到统计物理学,从进化生物学到计算机科学。
组合数学Combinatorial Mathematics因其解决的问题的广泛性而闻名。组合问题出现在纯数学的许多领域,特别是在代数、概率论、拓扑学和几何学中,]以及在其许多应用领域。许多组合问题在历史上都是孤立考虑的,对某个数学背景下出现的问题给出一个临时的解决方案。然而,在二十世纪后期,强大而普遍的理论方法被开发出来,使组合学本身成为一个独立的数学分支。组合学最古老和最容易理解的部分之一是图论,它本身与其他领域有许多自然联系。在计算机科学中,组合学经常被用来获得算法分析中的公式和估计。
my-assignmentexpert™ 组合数学Combinatorial Mathematics作业代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。my-assignmentexpert™, 最高质量的组合数学Combinatorial Mathematics作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于统计Statistics作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此组合数学Combinatorial Mathematics作业代写的价格不固定。通常在经济学专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
my-assignmentexpert™ 为您的留学生涯保驾护航 在数学Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的组合数学Combinatorial Mathematics代写服务。我们的专家在数学Mathematics代写方面经验极为丰富,各种组合数学Combinatorial Mathematics相关的作业也就用不着 说。
我们提供的组合数学Combinatorial Mathematics及其相关学科的代写,服务范围广, 其中包括但不限于:

数学代写|组合数学作业代写Combinatorial Mathematics代考|Connectivity Parameters
How difficult is it to disconnect a graph? We consider first deletion of vertices and then deletion of edges.
SEPARATING SETS
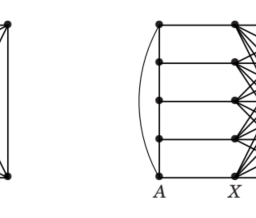
7.1.1. Definition. A separating set or vertex cut of a graph $G$ is a set $S \subseteq V(G)$ such that $G-S$ has more than one component. A graph $G$ is $k$-connected if it has more than $k$ vertices and every vertex cut has size at least $k$. The connectivity of $G$, written $\kappa(G)$, is the maximum $k$ such that $G$ is $k$-connected.
A non-complete graph $G$ has a separating set, and $\kappa(G)$ is the minimum size of such a set. In this case, the neighborhood of a vertex of minimum degree is a separating set, and hence $\kappa(G) \leq \delta(G)$. Also, if a graph $G$ is $k$-connected, and $k>0$, then $G$ is also $(k-1)$-connected, by the definition.
7.1.2. Example. Connectivity of $K_{n}$ and $K_{r, s}$. A complete graph has no separating set. By requiring $k$-connected graphs to have more than $k$ vertices, we obtain $\kappa\left(K_{n}\right)=n-1$. This allows general connectivity results (such as $\left.\kappa(G) \leq \delta(G)\right)$ to hold also for $K_{n}$. A graph with more than two vertices has connectivity 1 if it is connected and has a cut-vertex. A graph with more than one vertex has connectivity 0 if and only if it is disconnected. Unfortunately, $K_{1}$ is an anomaly; it is connected but has connectivity 0 .
Every induced subgraph of $K_{r, s}$ having at least one vertex from each part is connected. Hence every vertex cut contains a full part, and $\kappa\left(K_{r, s}\right)=\min {r, s}$ (the convention for $K_{2}$ is consistent with this).
数学代写|组合数学作业代写Combinatorial Mathematics代考|Properties of k-Connected Graphs
Being $k$-connected is more restrictive than being connected. When $G$ is connected and $x, y \in V(G)$, there is an $x, y$-path. When $G$ has $k$ such paths sharing no internal vertices, separating $y$ from $x$ requires deleting at least $k$ vertices. We will show that this obviously sufficient condition for being $k$-connected is also necessary. When phrased appropriately, these concepts apply also to digraphs.
7.2.1. Definition. A digraph $D$ is strongly connected (or strong) if it contains a path from each vertex to every other. It is $k$-connected if it has more than $k$ vertices and $D-S$ is strong for any set $S \subseteq V(D)$ with $|S|<k$. The connectivity $\kappa(D)$ is the maximum $k$ such that $D$ is $k$-connected. For $\oslash \neq S \subset V(D)$, the edge cut $[S, \bar{S}]$ is the set of edges from $S$ to $\bar{S}$. A digraph $D$ is $k$-edgeconnected if every edge cut has size at least $k$. The edge-connectivity $\kappa^{\prime}(D)$ is the minimum size of an edge cut.
Note that a graph or digraph $G$ is $k$-edge-connected if and only if for every nonempty proper vertex subset $S$, at least $k$ edges leave $S$.
数学代写|组合数学作业代写COMBINATORIAL MATHEMATICS代考|Spanning Cycles
A $k$-connected graph has a cycle through any $k$ vertices (Theorem 7.2.13), but cycles through larger sets are not guaranteed (Exercise 7.2.11). However, when the minimum degree is large enough in terms of the number of vertices, there is a cycle through all the vertices. We first consider conditions for spanning cycles, and later we discuss “long cycle” versions of these results.
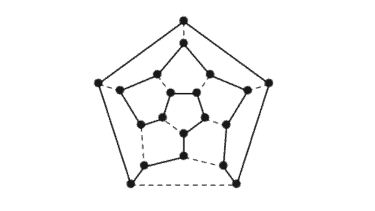
Spanning cycles were introduced by Kirkman in 1855 in a paper on polyhedral graphs. Nevertheless, they are called Hamiltonian cycles to honor Sir William Rowan Hamilton (1805-1865). In studying non-commutative algebra, he devised a game on the dodecahedron graph where one player would specify a 5-vertex path and the other would extend it to a spanning cycle. Hamilton sold “The Icosian Game” to a dealer in puzzles, for $£ 25$. In another version, “The Traveller’s Dodecahedron”, the vertices were named for 20 important cities. Neither was commercially successful, but Hamilton’s name was associated with the concept.
组合数学代写
数学代写|组合数学作业代写COMBINATORIAL MATHEMATICS代考|CONNECTIVITY PARAMETERS
断开图表有多难?我们考虑首先删除顶点,然后删除边。
分离集合
7.1.1。定义。图的分离集或顶点割G是一个集合小号⊆在(G)这样G−小号有不止一种成分。图表G是ķ- 如果它有超过ķ顶点和每个顶点切割至少有大小ķ. 的连通性G, 写ķ(G), 是最大值ķ这样G是ķ-连接的。
不完全图G有一个分离集,并且ķ(G)是这样一个集合的最小尺寸。在这种情况下,最小度数的顶点的邻域是一个分离集,因此ķ(G)≤d(G). 此外,如果一个图表G是ķ-连接,并且ķ>0, 然后G也是(ķ−1)-连接,根据定义。
7.1.2. 例子。连接性ķn和ķr,s. 完全图没有分离集。通过要求ķ-连通图有超过ķ顶点,我们得到 $K_{n}$ and $K_{r, s}$. A complete graph has no separating set. By requiring $k$-connected graphs to have more than $k$ vertices, we obtain $\kappa\left(K_{n}\right)=n-1$. This allows general connectivity results (such as $\left.\kappa(G) \leq \delta(G)\right)$ to hold also for $K_{n}$. A graph with more than two vertices has connectivity 1 if it is connected and has a cut-vertex. A graph with more than one vertex has connectivity 0 if and only if it is disconnected. Unfortunately, $K_{1}$是一个异常;它已连接但具有连接性 0 。
的每个诱导子图ķr,s每个部分至少有一个顶点是相连的。因此每个顶点切割都包含一个完整的部分,并且ķ(ķr,s)=分钟r,s 吨H和C这n在和n吨一世这nF这r$ķ2$一世sC这ns一世s吨和n吨在一世吨H吨H一世s.
数学代写|组合数学作业代写COMBINATORIAL MATHEMATICS代考|PROPERTIES OF K-CONNECTED GRAPHS
存在ķ-connected 比被连接更严格。什么时候G已连接并且X,是∈在(G), 有一个X,是-小路。什么时候G拥有ķ这样的路径不共享内部顶点,分离是从X至少需要删除ķ顶点。我们将证明这个明显的充分条件ķ-connected 也是必要的。如果措辞恰当,这些概念也适用于有向图。
7.2.1. 定义。有向图D是强连接的这rs吨r这nG如果它包含从每个顶点到其他顶点的路径。这是ķ- 如果它有超过ķ顶点和D−小号对任何集合都很强大小号⊆在(D)和|小号|<ķ. 连通性ķ(D)是最大值ķ这样D是ķ-连接的。为了⊘≠小号⊂在(D), 边缘切割[小号,小号¯]是来自的边集小号到小号¯. 有向图D是ķ-edgeconnected 如果每个边缘切割至少有大小ķ. 边缘连接ķ′(D)是边缘切割的最小尺寸。
请注意,图或有向图G是ķ-edge-connected 当且仅当对于每个非空的适当顶点子集小号, 至少ķ边缘离开小号.
数学代写|组合数学作业代写COMBINATORIAL MATHEMATICS代考|SPANNING CYCLES
一种ķ-连通图有一个循环通过任何ķ顶点吨H和这r和米7.2.13,但不能保证循环通过更大的集合和X和rC一世s和7.2.11. 但是,当最小度数就顶点数而言足够大时,所有顶点都有一个循环。我们首先考虑跨越周期的条件,然后我们讨论这些结果的“长周期”版本。
1855 年,柯克曼在一篇关于多面体图的论文中引入了跨越循环。尽管如此,它们被称为哈密顿循环以纪念威廉·罗文·汉密尔顿爵士1805−1865. 在研究非交换代数时,他在十二面体图上设计了一个游戏,其中一个玩家将指定一个 5 顶点路径,另一个将其扩展到一个跨越循环。汉密尔顿将“伊科西亚游戏”卖给了一位拼图经销商,££25. 在另一个版本“旅行者的十二面体”中,顶点以 20 个重要城市命名。两者都没有在商业上取得成功,但汉密尔顿的名字与这个概念有关。

数学代写|组合数学作业代写Combinatorial Mathematics代考 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。