如果你也在 怎样代写multi-level modeling:nested这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。multi-level modeling:nested多层次模型(也称为分层线性模型、线性混合效应模型、混合模型、嵌套数据模型、随机系数、随机效应模型、随机参数模型或分割图设计)是在一个以上层次上变化的参数的统计模型。这些模型可以被看作是线性模型(尤其是线性回归)的概括,尽管它们也可以扩展到非线性模型。在有了足够的计算能力和软件之后,这些模型变得更加流行了。
multi-level modeling:nested多层次模型特别适合于研究设计,即参与者的数据被组织在一个以上的层次(即嵌套数据)。分析单位通常是个人(较低层次),他们被嵌套在背景/总体单位(较高层次)中。虽然多层次模型中最低层次的数据通常是个人,但也可以研究个人的重复测量。因此,多层次模型为重复测量的单变量或多变量分析提供一种替代的分析类型。此外,多水平模型还可以用来替代方差分析,在方差分析中,因变量的分数在测试处理差异之前会根据协变量(如个体差异)进行调整。多水平模型能够分析这些实验,而不需要方差分析所要求的回归斜率的同质性假设。
my-assignmentexpert™multi-level modeling:nested作业代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。my-assignmentexpert™, 最高质量的multi-level modeling:nested作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于统计Statistics作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此multi-level modeling:nested作业代写的价格不固定。通常在经济学专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
my-assignmentexpert™ 为您的留学生涯保驾护航 在数学Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在multi-level modeling:nested代写方面经验极为丰富,各种multi-level modeling:nested相关的作业也就用不着 说。
我们提供的multi-level modeling:nested及其相关学科的代写,服务范围广, 其中包括但不限于:
数学代写|multi-level modeling:nested代考|Residence Time Distribution, RTD
The problem of mixing in chemically reactive flows is of technological importance in chemical reactors, combustion and propulsion systems, environmental studies, pharmacology, biophysics and so on. The aim of the research in the domain of mixing is to obtain an understanding of the physics of mixing, of the effect of these motions on the transport properties, of the effect of chemical kinetics and to combine all these aspects in the form of a model of the process. Physical effects in chemical reactors are difficult to separate from the chemical rate processes. In trying to do so one usually distinguishes between chemical kinetics and fluid dynamics putting down the performance equation of a chemical reactor as follows:
Output $=\mathrm{f}$ (input, kinetics, flow pattern)
While turbulent mixing continues to be largely a very difficult subject, there exists a considerable need for the development of methods for the calculation of mean flow properties. A number of predictive methods have therefore been developed based largely on heuristic reasoning. Improvements in such methods have arisen principally through the use of computational facilities. At present the mathematical description of the intricate process in a chemical device is usually worked out with the aid of approximate pictures of the internal structure of the flows (Fogler 2006).
Two types of ideal flow are commonly used as limits of flow patterns in process vessels; these are the “plug flow” and the “perfectly mixed” flow. The conditions for the physical realization of the plug flow are fulfilled in a pistontype flow, when it is assumed that no mixing takes place in the direction of flow. The model is employed to describe tubular apparatus with a large length-todiameter ratio. At the other extreme, perfect mixing assumes that the vessel contents are completely homogeneous and the outlet-stream properties are identical to the vessel-fluid properties. In chemical engineering the usual tendency is to come closer to conditions of perfect mixing by fitting apparatus with special mixers, baffle plates etc. Non-idealities of flow in industrial apparatus can be traced to the following most important reasons: presence of dead spaces, channeling or by passing, recycling or cross-flow streams, developed turbulence etc. Stagnant fluid or dead spaces represent regions with extremely poor contacting. A dead space will contain fluid elements for interval of time with an order of magnitude over the mean residence time. In bypassing or channeling some of the fluids slip or pass through the vessel considerably faster than others do. Bypassing may be found in flow through poorly packed vessels, through heat exchanger in two-phase operations etc. In recycling a certain amount of fluid is recirculated or returned to the vessel inlet. This type of flow may be desirable for example in auto-thermal reactions. When constructing a flow model for a given chemical reactor one starts by knowledge of the pattern of fluid passage through the reactor. This flow behavior could be determined by finding the complete history of each fluid element. It was pointed out that, instead of this complexity of the flow pattern, it is enough to know how long the fluid elements stay in the reactor, in other words, to determine the RTD of the fluid particles in the exit stream.
数学代写|multi-level modeling:nested代考|Discrete Model for Residence Time Distributions
The RTD for discrete-time measurements in flow systems exhibiting many scales of time particularly for systems presenting a number of parallel pathways with widely different residence time is described here. Denote by $x(n)$ the input at the moment $n$, by $y$ (n) the output at the same moment and by $h$ the RTD function, then:
$$
\mathrm{y}(\mathrm{n})=\sum_{\infty}^{-\infty} h(\mathrm{n}-\mathrm{k}) \mathrm{x}(\mathrm{k})=\mathrm{h}(\mathrm{n}) * \mathrm{x}(\mathrm{n})
$$
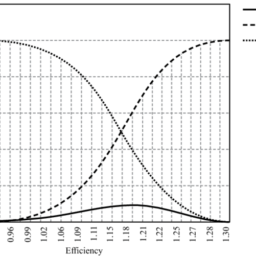
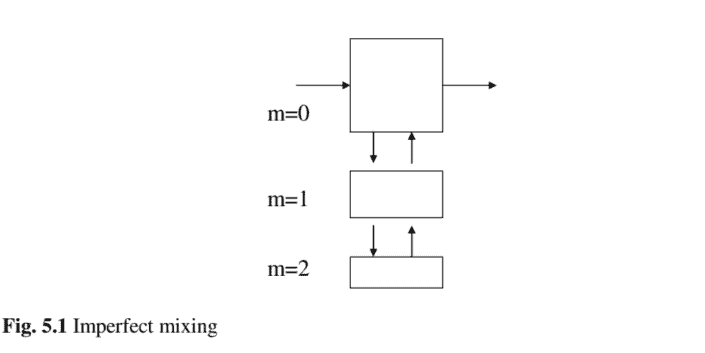
Here ” $*$ ” is the convolution mark and $\mathrm{h}(\mathrm{n}-\mathrm{k})$ stands for the output at the moment $\mathrm{n}$ due to an input which is equal to unit at the moment $\mathrm{k}$ and is null for all the others. Several discrete-time studies have attempted to develop methods for interpreting inlet-outlet tracer tests in vessels and mixers with flow heterogeneities. The model developed in the following is based on the observation that perturbations of an RTD take place at different scales of time. Possible behavior of mixing systems could be classified using the number $m$ of conditioning levels. Such levels correspond to a hierarchy of dead spaces in the sense that the space related to the level $\mathrm{m}$ appears as a dead space with respect to the space corresponding to the level m-1 but as short-circuit with respect to the space related to the level $\mathrm{m}+1$. Intuitively a complex system presenting $\mathrm{m}$ parallel pathways with strongly different residence times should give rise to a hierarchy of $\mathrm{m}$ conditioning levels corresponding to $\mathrm{m}$ more slower motions. Obviously in this picture a scale of time is associated to each conditioning level.
Fig. $5.1$ shows an imperfect mixing system. In the physical picture shown in Fig. $5.1$ there exist three scales of time. The space corresponding to $\mathrm{m}=0$ is active while those corresponding to $m=1$ and $m=2$ are more and more slow.
A classical quantitative approach to the non-ideality of mixing is due Cholette and Cloutier (1959) who proposed several models for a real stirred tank consisting of a “perfectly mixed” region a “dead” or “stagnant” region and a certain fraction of the feed by passing both regions. In this model three scales of time have been considered.
数学代写|MULTI-LEVEL MODELING:NESTED代考|Local Anesthetic Effects
The primary effect of chemical inactivation of the membrane functions by drugs or other chemicals consists of modifying the conformations of the active membranes components.
Anesthetics are supposed to act on the excitable membrane in a charged form inside the nerve axon (Strichartz and Ritchie 1987).
Some correlations of the exponential type have been proposed for the relaxation of the amplitude of compound action potential. An application of multi-scale models concerns some experiments describing the decrease of the action potential by procaine (Iordache and Frangopol 1988a, Iordache et al. 1988a).
Table $5.1$ shows experimental data, the action potential relative amplitude of procaine $10 \mathrm{mM}$ as a function of time step $\mathrm{n}$.
The value of $\alpha$ in eq. (5.23) may be correlated to the mean residence time, $\overline{\mathrm{n}}=1 /(1-\alpha)$. Taking $\overline{\mathrm{n}}=6.25$ it results $\alpha=0.84$ (the time step size is $5 \mathrm{~min}$ ).
我们得出结论,$M=1$ 提供了所研究现象的令人满意的画面。
对于 $\mathrm{M}=1$,系数为 $\mathrm{q} {0}=0.878$ 和 $\mathrm{q} {1}=0.081$。
表 $5.1$ 还比较了扰动模型 $(M=1)$ 和理想模型 $(M=0)$。$M=2$ 显示不相关的结果。
如果流量和动力学的统计数据以及系数 $\mathrm{q}_{\mathrm{m}}$ 已知,则多尺度建模方法可以代表麻醉治疗先验设计的进展。更多尺度的过程的存在表明给定尺度的激发或抑制可能是药物动力学控制的有效手段。
多层线性模型代写
数学代写|MULTI-LEVEL MODELING:NESTED代考|RESIDENCE TIME DISTRIBUTION, RTD
化学反应流中的混合问题在化学反应器、燃烧和推进系统、环境研究、药理学、生物物理学等领域具有重要的技术意义。混合领域研究的目的是了解混合的物理特性、这些运动对传输特性的影响、化学动力学的影响,并以模型的形式将所有这些方面结合起来的过程。化学反应器中的物理效应很难与化学速率过程分开。在尝试这样做时,通常会区分化学动力学和流体动力学,将化学反应器的性能方程如下:
输出=F 一世np在吨,ķ一世n和吨一世Cs,Fl这在p一种吨吨和rn
虽然湍流混合在很大程度上仍然是一个非常困难的主题,但仍然非常需要开发用于计算平均流动特性的方法。因此,已经开发了许多主要基于启发式推理的预测方法。此类方法的改进主要是通过使用计算设施来实现的。目前,化学装置中复杂过程的数学描述通常是借助流动内部结构的近似图片来完成的。F这Gl和r2006.
两种类型的理想流量通常用作工艺容器中流动模式的限制;这些是“活塞流”和“完美混合”流。当假定在流动方向上不发生混合时,活塞式流动满足了活塞流的物理实现条件。该模型用于描述具有大长径比的管状设备。在另一个极端,完美混合假设容器内容物完全均匀,并且出口流特性与容器流体特性相同。在化学工程中,通常的趋势是通过为设备配备特殊的混合器、挡板等来接近完美混合的条件。工业设备中流动的不理想可以追溯到以下最重要的原因:死区的存在、通道或通过、再循环或错流流、发展的湍流等。停滞流体或死区代表接触极差的区域。死区将包含时间间隔内的流体元素,其平均停留时间的数量级。在绕过或引导一些流体时,一些流体比其他流体滑出或通过容器的速度要快得多。在流过填充不良的容器、通过两相操作中的热交换器等的流动中可能会发现旁路。在再循环中,一定量的流体被再循环或返回到容器入口。例如在自热反应中可能需要这种类型的流动。在为给定的化学反应器构建流动模型时,首先要了解流体通过反应器的模式。这种流动行为可以通过查找每个流体元素的完整历史来确定。有人指出,除了这种复杂的流动模式,知道流体元素在反应器中停留的时间就足够了,换句话说,可以确定出口流中流体颗粒的 RTD。
数学代写|MULTI-LEVEL MODELING:NESTED代考|DISCRETE MODEL FOR RESIDENCE TIME DISTRIBUTIONS
此处描述了用于在具有许多时间尺度的流动系统中进行离散时间测量的 RTD,特别是对于呈现许多具有不同停留时间的并行路径的系统。表示为X(n)此刻的输入n, 经过是 n同时输出HRTD 函数,然后:
是(n)=∑∞−∞H(n−ķ)X(ķ)=H(n)∗X(n)
这里 ”∗” 是卷积标记并且H(n−ķ)代表此刻的输出n由于此时输入等于单位ķ并且对于所有其他人都是空的。一些离散时间研究试图开发方法来解释具有流动异质性的容器和混合器中的入口-出口示踪剂测试。下面开发的模型基于对 RTD 扰动发生在不同时间尺度上的观察。混合系统的可能行为可以使用数字进行分类米的调理水平。这些级别对应于死空间的层次结构,因为空间与级别相关米相对于级别 m-1 的空间显示为死区,但相对于级别相关的空间显示为短路米+1. 直观地呈现一个复杂的系统米具有强烈不同停留时间的平行路径应该产生一个层次结构米对应的调节水平米更慢的动作。显然,在这张图片中,时间尺度与每个调节级别相关联。
如图。5.1显示了一个不完美的混合系统。如图所示的实物图。5.1存在三个时间尺度。对应的空间米=0是活跃的,而那些对应于米=1和米=2越来越慢。
混合非理想性的经典定量方法归功于 Cholette 和 Cloutier1959他为真正的搅拌罐提出了几种模型,包括“完全混合”区域、“死”或“停滞”区域以及通过这两个区域的一定比例的进料。在这个模型中,考虑了三个时间尺度。
数学代写|MULTI-LEVEL MODELING:NESTED代考|LOCAL ANESTHETIC EFFECTS
药物或其他化学物质对膜功能的化学灭活的主要作用包括改变活性膜成分的构象。
麻醉剂应该以带电形式作用于神经轴突内的可兴奋膜小号吨r一世CH一种r吨和一种ndR一世吨CH一世和1987.
已经提出了一些指数类型的相关性来放松复合动作电位的幅度。多尺度模型的应用涉及一些描述普鲁卡因降低动作电位的实验一世这rd一种CH和一种ndFr一种nG这p这l1988一种,一世这rd一种CH和和吨一种l.1988一种.
桌子5.1显示实验数据,普鲁卡因的动作电位相对幅度10米米作为时间步长的函数n.
的价值一种在等式。5.23可能与平均停留时间相关,n¯=1/(1−一种). 服用n¯=6.25结果一种=0.84 吨H和吨一世米和s吨和ps一世和和一世s$5 米一世n$.
我们得出结论,米=1 提供了所研究现象的令人满意的画面。
对于 米=1,系数为 $\mathrm{q} {0}=0.878和和\mathrm{q} {1}=0.081$。
表 5.1 还比较了扰动模型 (米=1) 和理想模型 (米=0)。米=2 显示不相关的结果。
如果流量和动力学的统计数据以及系数 q米 已知,则多尺度建模方法可以代表麻醉治疗先验设计的进展。更多尺度的过程的存在表明给定尺度的激发或抑制可能是药物动力学控制的有效手段。

数学代写|multi-level modeling:nested代考 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。
微观经济学代写
微观经济学是主流经济学的一个分支,研究个人和企业在做出有关稀缺资源分配的决策时的行为以及这些个人和企业之间的相互作用。my-assignmentexpert™ 为您的留学生涯保驾护航 在数学Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在图论代写Graph Theory代写方面经验极为丰富,各种图论代写Graph Theory相关的作业也就用不着 说。
线性代数代写
线性代数是数学的一个分支,涉及线性方程,如:线性图,如:以及它们在向量空间和通过矩阵的表示。线性代数是几乎所有数学领域的核心。
博弈论代写
现代博弈论始于约翰-冯-诺伊曼(John von Neumann)提出的两人零和博弈中的混合策略均衡的观点及其证明。冯-诺依曼的原始证明使用了关于连续映射到紧凑凸集的布劳威尔定点定理,这成为博弈论和数学经济学的标准方法。在他的论文之后,1944年,他与奥斯卡-莫根斯特恩(Oskar Morgenstern)共同撰写了《游戏和经济行为理论》一书,该书考虑了几个参与者的合作游戏。这本书的第二版提供了预期效用的公理理论,使数理统计学家和经济学家能够处理不确定性下的决策。
微积分代写
微积分,最初被称为无穷小微积分或 “无穷小的微积分”,是对连续变化的数学研究,就像几何学是对形状的研究,而代数是对算术运算的概括研究一样。
它有两个主要分支,微分和积分;微分涉及瞬时变化率和曲线的斜率,而积分涉及数量的累积,以及曲线下或曲线之间的面积。这两个分支通过微积分的基本定理相互联系,它们利用了无限序列和无限级数收敛到一个明确定义的极限的基本概念 。
计量经济学代写
什么是计量经济学?
计量经济学是统计学和数学模型的定量应用,使用数据来发展理论或测试经济学中的现有假设,并根据历史数据预测未来趋势。它对现实世界的数据进行统计试验,然后将结果与被测试的理论进行比较和对比。
根据你是对测试现有理论感兴趣,还是对利用现有数据在这些观察的基础上提出新的假设感兴趣,计量经济学可以细分为两大类:理论和应用。那些经常从事这种实践的人通常被称为计量经济学家。
Matlab代写
MATLAB 是一种用于技术计算的高性能语言。它将计算、可视化和编程集成在一个易于使用的环境中,其中问题和解决方案以熟悉的数学符号表示。典型用途包括:数学和计算算法开发建模、仿真和原型制作数据分析、探索和可视化科学和工程图形应用程序开发,包括图形用户界面构建MATLAB 是一个交互式系统,其基本数据元素是一个不需要维度的数组。这使您可以解决许多技术计算问题,尤其是那些具有矩阵和向量公式的问题,而只需用 C 或 Fortran 等标量非交互式语言编写程序所需的时间的一小部分。MATLAB 名称代表矩阵实验室。MATLAB 最初的编写目的是提供对由 LINPACK 和 EISPACK 项目开发的矩阵软件的轻松访问,这两个项目共同代表了矩阵计算软件的最新技术。MATLAB 经过多年的发展,得到了许多用户的投入。在大学环境中,它是数学、工程和科学入门和高级课程的标准教学工具。在工业领域,MATLAB 是高效研究、开发和分析的首选工具。MATLAB 具有一系列称为工具箱的特定于应用程序的解决方案。对于大多数 MATLAB 用户来说非常重要,工具箱允许您学习和应用专业技术。工具箱是 MATLAB 函数(M 文件)的综合集合,可扩展 MATLAB 环境以解决特定类别的问题。可用工具箱的领域包括信号处理、控制系统、神经网络、模糊逻辑、小波、仿真等。