如果你也在 怎样代写统计物理Statistical Physics of Matter PHYS730这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。统计物理Statistical Physics of Matter是在统计力学的基础上发展起来的一个物理学分支,它在解决物理问题时使用概率论和统计学的方法,特别是处理大群体和近似的数学工具。它可以描述各种具有内在随机性的领域。其应用包括物理学、生物学、化学、神经科学等领域的许多问题。它的主要目的是以支配原子运动的物理定律来阐明物质的总体属性。
统计物理Statistical mechanics解释并定量描述了超导性、超流性、湍流、固体和等离子体的集体现象以及液体的结构特征。它是现代天体物理学的基础。在固态物理学中,统计物理学有助于液晶、相变和临界现象的研究。许多物质的实验研究完全基于系统的统计描述。其中包括冷中子、X射线、可见光等的散射。统计物理学在材料科学、核物理学、天体物理学、化学、生物学和医学(如研究传染病的传播)中也发挥了作用。
my-assignmentexpert™ 统计物理Statistical Physics of Matter作业代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。my-assignmentexpert™, 最高质量的统计物理Statistical Physics of Matter作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于统计Statistics作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此统计物理Statistical Physics of Matter作业代写的价格不固定。通常在经济学专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
my-assignmentexpert™ 为您的留学生涯保驾护航 在物理physics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的物理physics代写服务。我们的专家在统计物理Statistical Physics of Matter代写方面经验极为丰富,各种统计物理Statistical Physics of Matter相关的作业也就用不着 说。
我们提供的统计物理Statistical Physics of Matter PHYS730及其相关学科的代写,服务范围广, 其中包括但不限于:
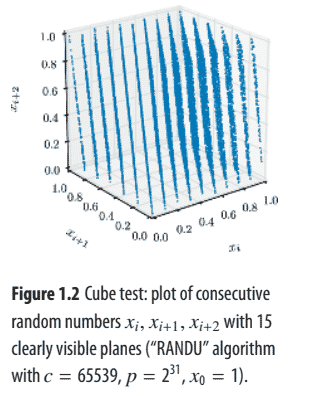
物理代写|统计物理代写Statistical Physics of Matter代考|Definition of Random Numbers
Random numbers are a sequence of numbers in random order. The probability that a given number occurs next in such a sequence is always the same. Physical systems can produce random events, for example, in electronic circuits (electronic flicker noise) and in systems where quantum effects play an important role (e.g., radioactive decay or photon emissions in semiconductors). However, physical random numbers are usually bad in the sense that they are often correlated and not reproducible.
Generating random numbers with algorithms is also a bit problematic because computers are completely deterministic, while randomness should be nondeterministic. We therefore must content ourselves with generating so-called pseudo-random numbers, which are computed with deterministic algorithms based on strongly nonlinear functions. These numbers should follow a well-defined distribution and should have long periods. Furthermore, we should be able to compute them quickly and in a reproducible way.
A very important function for generating pseudo-random numbers is the modulo operator $\bmod (\%$ in $\mathrm{C}++$. It determines the remainder of a division of one integer number by another one.
Given two numbers $a$ (dividend) and $n$ (divisor), we write $a$ modulo $n$ or $a \bmod n$, which represents the remainder of dividing $a$ by $n$. For the mathematical definition of the modulo operator, we consider the integers $a, q$, and $r$. We then express $a$ as
$$
a=n q+r,
$$
where $r(0 \leq r<|n|)$ is the remainder (i.e., the result of $a \bmod n)$. One useful property of the mod operator for generating RNs is that it is a strongly nonlinear function.
We distinguish between two classes of pseudo-random number generators (RNGs): multiplicative and additive RNGs.
- Multiplicative RNGs are simpler and faster to program and are based on integers.
- Additive RNGs are more difficult to implement and are based on binary variables.
In the following sections, we describe these RNGs in detail and outline different methods that allow us to examine the quality of random sequences.
物理代写|统计物理代写Statistical Physics of Matter代考|Congruential RNG (Multiplicative)
This iteration generates random numbers in the interval $[0, p-1]^{1}$. To transform a random number $x_{i} \in[0, p-1]$ into a normalized random number $z_{i} \in[0,1)$, we simply divide $x_{i}$ by $p$ and obtain
$$
0 \leq z_{i}=\frac{x_{i}}{p}<1,
$$
where $z_{i}$ is a rational number (i.e., $z_{i} \in \mathbb{Q}$ ). The random numbers $z_{i}$ are homogeneously distributed, which means that every number between 0 and 1 is equally probable.
Since all integers are smaller than $p$, the sequence must repeat after maximally $(p-1)$ iterations. Thus, the maximal period of the RNG defined by eq. (1.2) is $(p-1)$. If we pick the seed value $x_{0}=0$, the sequence remains at this value. Therefore, $x_{0}=0$ cannot be used as a seed of the described congruential RNG.
In 1910, the American mathematician Robert D. Carmichael [4] proved that the maximal period of a congruential RNG can be obtained if $p$ is a Mersenne prime number and if it is the smallest integer number that satisfies
$$
c^{p-1} \bmod p=1 .
$$

统计物理代写
物理代写|统计物理代写STATISTICAL PHYSICS OF MATTER代 考|DEFINITION OF RANDOM NUMBERS
随机数是随机顺序的数字序列。在这样的序列中,给定数字下一个出现的概率总是相同的。物理系统可以产生随机事件,例如在电子电路中
electronicflickernoise以及在量子效应发挥重要作用的系统中e.g., radioactivedecayorphotonemissionsinsemiconductors. 然而,物理随机数通常是不好
的,因为它们通常是相关的并且不可重现。
用算法生成随机数也有点问题,因为计算机是完全确定的,而随机性应该是不确定的。因此,我们必须满足于生成所谓的伪随机数,这些伪随机数是使用基于强非
线性函数的确定性算法计算的。这些数字应该道循明确的分布,并且应该有很长的周期。此外,我们应该能够以可重夏的方式快速计算它们。
生成伪随机数的一个非常重要的函数是模运算符 $\bmod (\%$ 在 $\mathrm{C}++$. 它确定一个整数除以另一个整数的余数。
给定两个数字 $a$ dividend和 $n$ divisor,我们写 $a$ 模块 $n$ 或者 $a \bmod n$ ,表示除法的余数 $a$ 经过 $n$. 对于模运算符的数学定义,我们考虑整数 $a, q$ ,和 $r$. 然后我们表达 $a$
作为
在哪里 $r(0 \leq r<|n|)$ 是余数i.e., theresultof $\$ a \bmod n$ 美元。用于生成 RN 的 mod 算子的一个有用特性是它是一个强非线性函数。
我们区分两类伪随机数生成器 $R N G s$ : 乘法和加法 RNG。
乘法 RNG 编程更简单、更快,并且基于整数。
加法 RNG 更难实现,并且基于二进制变量。
在以下部分中,我们将详细描述这些 RNG,并概述允许我们检亘随机序列质量的不同方法。
物理代写|统计物理代写STATISTICAL PHYSICS OF MATTER代 考|CONGRUENTIAL RNG Multiplicative
此迭代在区间内生成随机数 $[0, p-1]^{1}$. 转换随机数 $x_{i} \in[0, p-1]$ 成一个归一化的随机数 $z_{i} \in[0,1)$ ,我们简单地划分 $x_{i}$ 经过 $p$ 并获得
$$
0 \leq z_{i}=\frac{x_{i}}{p}<1,
$$
在哪里 $z_{i}$ 是有理数 $i . e ., \$ z_{i} \in \mathbb{Q} \$$. 随机数 $z_{i}$ 是均匀分布的,这意味着 0 和 1 之间的每个数字都是等概率的。
因为所有整数都小于 $p$ ,该序列必须在最大重复之后 $(p-1)$ 迭代。因此,由等式定义的 RNG 的最大周期。 $1.2$ 是 $(p-1)$. 如果我们选择种子值 $x_{0}=0$ ,序列保持在
这个值。所以, $x_{0}=0$ 不能用作所描述的同余 RNG 的种子。
1910 年,美国数学家罗伯特·D·卡迈克尔
证明了可以得到同余 RNG 的最大周期,如果 $p$ 是梅森靑数,如果它是满足的最小整数
$c^{p-1} \bmod p=1$

物理代写|统计物理代写STATISTICAL PHYSICS OF MATTER代考 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。
电磁学代考
物理代考服务:
物理Physics考试代考、留学生物理online exam代考、电磁学代考、热力学代考、相对论代考、电动力学代考、电磁学代考、分析力学代考、澳洲物理代考、北美物理考试代考、美国留学生物理final exam代考、加拿大物理midterm代考、澳洲物理online exam代考、英国物理online quiz代考等。
光学代考
光学(Optics),是物理学的分支,主要是研究光的现象、性质与应用,包括光与物质之间的相互作用、光学仪器的制作。光学通常研究红外线、紫外线及可见光的物理行为。因为光是电磁波,其它形式的电磁辐射,例如X射线、微波、电磁辐射及无线电波等等也具有类似光的特性。
大多数常见的光学现象都可以用经典电动力学理论来说明。但是,通常这全套理论很难实际应用,必需先假定简单模型。几何光学的模型最为容易使用。
相对论代考
上至高压线,下至发电机,只要用到电的地方就有相对论效应存在!相对论是关于时空和引力的理论,主要由爱因斯坦创立,相对论的提出给物理学带来了革命性的变化,被誉为现代物理性最伟大的基础理论。
流体力学代考
流体力学是力学的一个分支。 主要研究在各种力的作用下流体本身的状态,以及流体和固体壁面、流体和流体之间、流体与其他运动形态之间的相互作用的力学分支。
随机过程代写
随机过程,是依赖于参数的一组随机变量的全体,参数通常是时间。 随机变量是随机现象的数量表现,其取值随着偶然因素的影响而改变。 例如,某商店在从时间t0到时间tK这段时间内接待顾客的人数,就是依赖于时间t的一组随机变量,即随机过程
Matlab代写
MATLAB 是一种用于技术计算的高性能语言。它将计算、可视化和编程集成在一个易于使用的环境中,其中问题和解决方案以熟悉的数学符号表示。典型用途包括:数学和计算算法开发建模、仿真和原型制作数据分析、探索和可视化科学和工程图形应用程序开发,包括图形用户界面构建MATLAB 是一个交互式系统,其基本数据元素是一个不需要维度的数组。这使您可以解决许多技术计算问题,尤其是那些具有矩阵和向量公式的问题,而只需用 C 或 Fortran 等标量非交互式语言编写程序所需的时间的一小部分。MATLAB 名称代表矩阵实验室。MATLAB 最初的编写目的是提供对由 LINPACK 和 EISPACK 项目开发的矩阵软件的轻松访问,这两个项目共同代表了矩阵计算软件的最新技术。MATLAB 经过多年的发展,得到了许多用户的投入。在大学环境中,它是数学、工程和科学入门和高级课程的标准教学工具。在工业领域,MATLAB 是高效研究、开发和分析的首选工具。MATLAB 具有一系列称为工具箱的特定于应用程序的解决方案。对于大多数 MATLAB 用户来说非常重要,工具箱允许您学习和应用专业技术。工具箱是 MATLAB 函数(M 文件)的综合集合,可扩展 MATLAB 环境以解决特定类别的问题。可用工具箱的领域包括信号处理、控制系统、神经网络、模糊逻辑、小波、仿真等。

