MY-ASSIGNMENTEXPERT™可以为您提供lse.ac.uk ST310 Machine Learning机器学习课程的代写代考和辅导服务!
这是伦敦政经学校机器学习课程的代写成功案例。
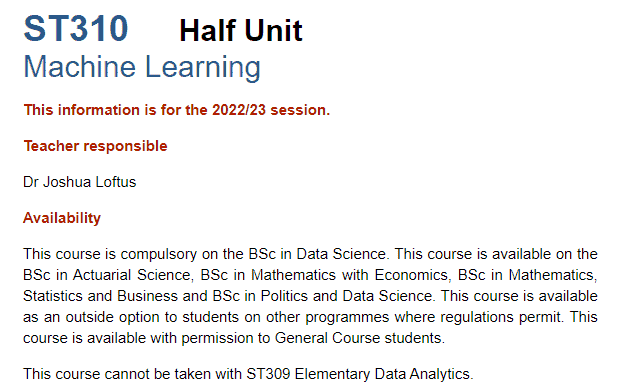
ST310课程简介
Teacher responsible
Dr Joshua Loftus
Availability
This course is compulsory on the BSc in Data Science. This course is available on the BSc in Actuarial Science, BSc in Mathematics with Economics, BSc in Mathematics, Statistics and Business and BSc in Politics and Data Science. This course is available as an outside option to students on other programmes where regulations permit. This course is available with permission to General Course students.
This course cannot be taken with ST309 Elementary Data Analytics.
Prerequisites
The primary focus of this course is on the core machine learning techniques in the context of high-dimensional or large datasets (i.e. big data). The first part of the course covers elementary and important statistical methods including nearest neighbours, linear regression, logistic regression, regularisation, cross-validation, and variable selection. The second part of the course deals with more advanced machine learning methods including regression and classification trees, random forests, bagging, boosting, deep neural networks, k-means clustering and hierarchical clustering. The course will also introduce causal inference motivated by analogy between double machine learning and two-stage least squares. All the topics will be delivered using illustrative real data examples. Students will also gain hands-on experience using R or Python programming languages and software environments for data analysis, computing and visualisation.
ST310 Machine Learning HELP(EXAM HELP, ONLINE TUTOR)
(Rank) Let $A=\left[\begin{array}{ccc}1 & 2 & 1 \ 1 & 0 & 3 \ 1 & 1 & 2\end{array}\right]$ and $B=\left[\begin{array}{ccc}1 & 2 & 3 \ 1 & 0 & 1 \ 1 & 1 & 2\end{array}\right]$. For each matrix $A$ and $B$,
a. [2 points] what is its rank?
b. [2 points] what is a (minimal size) basis for its column span?
a. To find the rank of a matrix, we can row reduce it to its echelon form and count the number of nonzero rows. Using row reduction, we obtain:
$$
\left[\begin{array}{lll}
1 & 2 & 1 \
1 & 0 & 3 \
1 & 1 & 2
\end{array}\right] \rightarrow\left[\begin{array}{ccc}
1 & 2 & 1 \
0 & -2 & 2 \
0 & -1 & 1
\end{array}\right] \rightarrow\left[\begin{array}{ccc}
1 & 2 & 1 \
0 & 1 & -1 \
0 & 0 & 0
\end{array}\right]
$$
Therefore, the echelon form of $\$ A \$$ has 2 nonzero rows, which means that the rank of $\$ A \$$ is $\$ \backslash$ boxed ${2} \$$.
Similarly, we can row reduce matrix $\$ B \$$ to its echelon form:
$$
\left[\begin{array}{lll}
1 & 2 & 3 \
1 & 0 & 1 \
1 & 1 & 2
\end{array}\right] \rightarrow\left[\begin{array}{ccc}
1 & 2 & 3 \
0 & -2 & -2 \
0 & -1 & -1
\end{array}\right] \rightarrow\left[\begin{array}{ccc}
1 & 2 & 3 \
0 & 1 & 1 \
0 & 0 & 0
\end{array}\right]
$$
Therefore, the echelon form of $\$ B \$$ has 2 nonzero rows, which means that the rank of $\$ B \$$ is also $\$ \backslash$ boxed ${2} \$$.
b. To find a basis for the column span of a matrix, we can extract the pivot columns from its echelon form. The pivot columns are the columns that contain the pivots (i.e., the leading nonzero entries) of the echelon form. These columns form a basis for the column span of the original matrix.
From the echelon form of $\$ A \$$, we see that the pivot columns are the first and second columns. Therefore, a basis for the column span of $\$ A \$$ is $\$ \backslash$ boxed ${$ left{ $\backslash$ begin ${$ bmatrix $} 1 \backslash 1 \backslash 1 \backslash$ end ${$ bmatrix $}$, begin ${$ bmatrix $} 2 \backslash 0 \backslash$ $1 \backslash$ end ${$ bmatrix $} \backslash$ right $}} \$$.
From the echelon form of $\$ B \$$, we see that the pivot columns are the first and second columns. Therefore, a basis for the column span of $\$ B \$$ is $\$ \backslash$ boxed{left{\begin } { \text { bmatrix } } 1 \backslash 1 \backslash 1 \backslash \text { end } { \text { bmatrix } } \text { , } \backslash \text { begin } { \text { bmatrix } } 2 \backslash 0 \backslash $1 \backslash$ end{bmatrix $} \backslash$ right $}} \$$. Notice that the same basis works for both $\$ A \$$ and $\$ B \$$ because they have the same echelon form up to row permutations.
(Linear equations) Let $A=\left[\begin{array}{lll}0 & 2 & 4 \\ 2 & 4 & 2 \\ 3 & 3 & 1\end{array}\right], b=\left[\begin{array}{lll}-2 & -2 & -4\end{array}\right]^T$, and $c=\left[\begin{array}{lll}1 & 1 & 1\end{array}\right]^T$. a. $[1$ points] What is $A c ?$ b. [2 points] What is the solution to the linear system $A x=b$ ? Show your work.
a. To compute $\$ A c \$$, we multiply the matrix $\$ A \$$ by the column vector $\$ c \$$ :
$$
A c=\left[\begin{array}{lll}
0 & 2 & 4 \
2 & 4 & 2 \
3 & 3 & 1
\end{array}\right]\left[\begin{array}{l}
1 \
1 \
1
\end{array}\right]=\left[\begin{array}{l}
0 \cdot 1+2 \cdot 1+4 \cdot 1 \
2 \cdot 1+4 \cdot 1+2 \cdot 1 \
3 \cdot 1+3 \cdot 1+1 \cdot 1
\end{array}\right]=\left[\begin{array}{l}
6 \
8 \
7
\end{array}\right]
$$
b. To solve the linear system $\$ A x=b \$$, we can first find the augmented matrix $\$[A \mid b] \$$ and then perform row reduction:
$$
\begin{aligned}
& {\left[\begin{array}{lll|l}
0 & 2 & 4 & -2 \
2 & 4 & 2 & -2 \
3 & 3 & 1 & -4
\end{array}\right] \rightarrow\left[\begin{array}{lll|l}
1 & 1 & \frac{1}{3} & -\frac{4}{3} \
0 & 1 & \frac{4}{3} & -\frac{2}{3} \
0 & 0 & -\frac{10}{3} & \frac{10}{3}
\end{array}\right] \rightarrow} \
& {\left[\begin{array}{lll|l}
1 & 0 & -\frac{1}{3} & -\frac{2}{3} \
0 & 1 & \frac{4}{3} & -\frac{2}{3} \
0 & 0 & 1 & -1
\end{array}\right] \rightarrow\left[\begin{array}{lll|l}
1 & 0 & 0 & 0 \
0 & 1 & 0 & 2 \
0 & 0 & 1 & -1
\end{array}\right] .}
\end{aligned}
$$
Therefore, the solution to the linear system is $\$ x=\backslash$ boxed $\backslash$ begin ${$ bmatrix $} 0$ $\backslash 2 \backslash-1 \backslash$ end ${$ bmatrix $}}$.

MY-ASSIGNMENTEXPERT™可以为您提供LSE.AC.UK ST310 MACHINE LEARNING机器学习课程的代写代考和辅导服务!