如果你也在 怎样代写图论Graph Theory 这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。图论Graph Theory在数学和计算机科学领域,图论是对图的研究,涉及边和顶点之间的关系。它是一门热门学科,在计算机科学、信息技术、生物科学、数学和语言学中都有应用。
图论Graph Theory在数学和计算机科学领域,图论是对图的研究,涉及边和顶点之间的关系。它是一门热门学科,在计算机科学、信息技术、生物科学、数学和语言学中都有应用。近年来,图论已经成为各种学科的重要数学工具,从运筹学和化学到遗传学和语言学,从电气工程和地理到社会学和建筑。同时,它本身也作为一门有价值的数学学科出现。
my-assignmentexpert™图论Graph Theory代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。my-assignmentexpert™, 最高质量的图论Graph Theory作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于统计Statistics作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此图论Graph Theory作业代写的价格不固定。通常在经济学专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
my-assignmentexpert™ 为您的留学生涯保驾护航 在网课代修方面已经树立了自己的口碑, 保证靠谱, 高质且原创的网课代写服务。我们的专家在图论Graph Theory代写方面经验极为丰富,各种图论Graph Theory相关的作业也就用不着 说。
我们提供的图论Graph Theory MATH334及其相关学科的代写,服务范围广, 其中包括但不限于:

数学代写|图论代写Graph Theory代写|Strong Digraphs
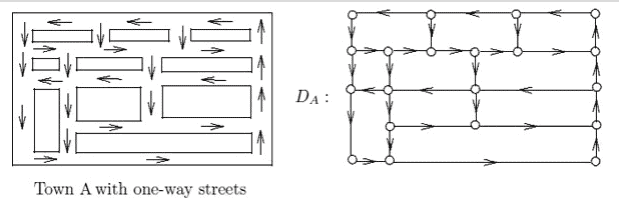
We saw in Section 6.1 that the street system of a town can be naturally represented by a graph. In this case, the vertices of the graph are the street intersections in the town, while the edges of the graph are the street segments between intersections. The street systems of two towns A and B are shown in Figure 7.1 together with the graphs $G_{\mathrm{A}}$ and $G_{\mathrm{D}}$ that model them.
Both graphs $G_A$ and $G_B$ of Figure 7.1 have the important property that they are connected, meaning that it is possible to travel between any two locations in both Town A and Town B. (Of course, this is a characteristic one would expect of any town.) The graph $G_B$ has a bridge, however, while $G_A$ does not. In fact, it may be the case that the street segment in Town B that gives rise to the bridge in $G_B$ is a road that goes over a bridge in the town. Of course, a major disadvantage of having such a street in Town B is that if it should ever become necessary to close that street, then traveling between some pairs of locations in Town B is impossible. We have no such difficulties in Town A, however. The fact that we can travel between any two street intersections in Town A even after one of its streets may be closed allows us to do something else in Town A, as we are about to discover. Before
continuing with this discussion, however, it is convenient to revisit the concept of a digraph (directed graph).
Recall that a digraph $D$ consists of a finite nonempty set $V$ of objects called vertices and a set $E$ of ordered pairs of distinct vertices. Each element of $E$ is an arc or a directed edge. If a digraph $D$ has the property that for each pair $u, v$ of distinct vertices of $D$, at most one of $(u, v)$ and $(v, u)$ is an $\operatorname{arc}$ of $D$, then $D$ is an oriented graph. An oriented graph can also be obtained by assigning a direction to (that is, orienting) each edge of a graph $G$. The digraph $D$ is then referred to as an orientation of $G$. A digraph $H$ is called a subdigraph of a digraph $D$ if $V(H) \subseteq V(D)$ and $E(H) \subseteq$ $E(D)$.
数学代写|图论代写Graph Theory代写|Tournaments
It is difficult to know just how far back competitions go. There have been competitions between two individuals (tennis, chess, bridge, jousting) and competitions between two teams (soccer, basketball, baseball). There have even been competitions between frogs, as Mark Twain wrote of Dan’l Webster in The Celebrated Jumping Frog of Calaveras County. In some competitions, there is a single match between two individuals or two teams and the victor in the match decides the outcome of the competition. In other competitions, often called tournaments, several individuals (or teams) are involved and there is a formula to decide who plays whom. Losing a match causes that individual or team to be eliminated and the tournament continues with those individuals who have won the earlier matches. Other tournaments are “double elimination,” where a player or team is allowed to lose one match but is eliminated when a second loss occurs.
Other tournaments are “round robin tournaments,” where each team plays every other team exactly once in the competition and the outcome of the tournament is decided only after all these matches have been played. For example, suppose that a round robin tournament involves eight teams (denoted by 1 , $2, \ldots, 8)$. Then every team must play each of the other seven teams once. In the first “round,” there are then four matches, each involving a pair of teams. There are seven rounds in this round robin tournament. Figure 7.8 shows how such a schedule might look. If only seven teams were involved, then in any round robin tournament only three matches can take place in a round with one team not playing (this team receives a “bye”). In this case, we can replace each occurrence of 8 in Figure 7.8 with “bye.” We will see in Section 8.2 how such schedules can be constructed.
Round robin tournaments give rise quite naturally to a class of digraphs, not so coincidentally called tournaments. A tournament is an orientation of a complete graph. Therefore, a tournament can be defined as a digraph such that for every pair $u, v$ of distinct vertices, exactly one of $(u, v)$ and $(v, u)$ is an arc. A tournament $T$ then models a round robin tournament. The vertices of $T$ are the teams in the round robin tournament and $(u, v)$ is an $\operatorname{arc}$ in $T$ if team $u$ defeats team $v$. (Ties are not permitted.)
图论代写
数学代写|图论代写Graph Theory代写|Strong Digraphs
我们在6.1节中看到,城镇的街道系统可以用图自然地表示。在这种情况下,图的顶点是城镇的街道交叉口,图的边是交叉口之间的街道段。A和B两个镇的街道系统如图7.1所示,以及对其建模的图形$G_{\ mathm {A}}$和$G_{\ mathm {D}}$。
图7.1中的两个图$G_A$和$G_B$都有一个重要的属性,即它们是相连的,这意味着可以在镇A和镇b的任意两个位置之间旅行(当然,这是任何城镇的一个特征)。然而,图$G_B$有桥,而$G_A$没有桥。事实上,可能是这样的情况:B镇的街道段产生了$G_B$中的桥梁,这是一条经过该镇桥梁的道路。当然,在B镇拥有这样一条街道的一个主要缺点是,如果有必要关闭这条街道,那么在B镇的一些地点之间旅行是不可能的。然而,我们在A镇没有这样的困难。我们可以在A镇的任意两个十字路口之间穿梭,即使其中一条街道可能已经关闭,这一事实允许我们在A镇做其他事情,正如我们即将发现的。然而,在
继续讨论之前,有向图(有向图)的概念是很方便的。
回想一下,有向图$D$由一个有限的非空集合$V$和一个由不同顶点的有序对$E$组成。$E$的每个元素是一条弧或一条有向边。如果有向图$D$具有这样的性质:对于$D$的不同顶点$u, v$, $(u, v)$和$(v, u)$中最多有一个是$D$的$\算子名{arc}$,则$D$是一个有向图。有向图也可以通过为图$G$的每条边指定方向(即定向)来获得。有向图$D$被称为$G$的方向。如果$V(H) \subseteq$ V(D)$和$E(H) \subseteq$ $E(D)$,则有向图$H$称为有向图$D$的子向图$H$。
数学代写|图论代写Graph Theory代写|Tournaments
很难知道竞争的历史有多久远。有两个人之间的比赛(网球、国际象棋、桥牌、比武)和两支球队之间的比赛(足球、篮球、棒球)。甚至还有青蛙之间的比赛,正如马克·吐温在《卡拉维拉斯县著名的跳蛙》中对丹·韦伯斯特的描写。在一些比赛中,两个人或两支队伍进行一场比赛,比赛的胜利者决定比赛的结果。在其他比赛中,通常被称为锦标赛,几个人(或团队)参与其中,并有一个公式来决定谁与谁比赛。输掉一场比赛将导致该个人或团队被淘汰,比赛继续进行,由之前赢得比赛的个人继续进行。其他比赛还有“双淘汰赛”,即允许一名球员或球队输掉一场比赛,但在第二场比赛中被淘汰。
其他比赛是“循环赛”,每支球队在比赛中只与其他球队进行一次比赛,比赛的结果只有在所有这些比赛结束后才能决定。例如,假设一个循环赛有八支球队参加(用1,$2,\ldots, 8表示)$。然后每队必须和其他七队各打一次。在第一轮比赛中,有四场比赛,每一场有一对球队参加。这次循环赛有七轮。图7.8显示了这样一个时间表的样子。如果只有七支球队参赛,那么在任何循环赛中,一轮只能进行三场比赛,其中一支球队不参加比赛(这支球队会收到“bye”)。在这种情况下,我们可以用“bye”替换图7.8中出现的每个8。我们将在8.2节中看到如何构造这样的时间表。
循环赛很自然地产生了一类有向图,并不是很巧合地被称为锦标赛。比武是完全图的一个方向。因此,锦标赛可以定义为一个有向图,使得对于每个不同顶点的$u, v$, $(u, v)$和$(v, u)$中恰好有一个是弧。一个锦标赛$T$然后模拟一个循环赛。$T$的顶点是循循赛中的球队,如果$u$击败$v$, $(u, v)$是$T$中的$\operatorname{arc}$。(不允许打领带)

数学代写|图论代写Graph Theory代写 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。
微观经济学代写
微观经济学是主流经济学的一个分支,研究个人和企业在做出有关稀缺资源分配的决策时的行为以及这些个人和企业之间的相互作用。my-assignmentexpert™ 为您的留学生涯保驾护航 在数学Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在图论代写Graph Theory代写方面经验极为丰富,各种图论代写Graph Theory相关的作业也就用不着 说。
线性代数代写
线性代数是数学的一个分支,涉及线性方程,如:线性图,如:以及它们在向量空间和通过矩阵的表示。线性代数是几乎所有数学领域的核心。
博弈论代写
现代博弈论始于约翰-冯-诺伊曼(John von Neumann)提出的两人零和博弈中的混合策略均衡的观点及其证明。冯-诺依曼的原始证明使用了关于连续映射到紧凑凸集的布劳威尔定点定理,这成为博弈论和数学经济学的标准方法。在他的论文之后,1944年,他与奥斯卡-莫根斯特恩(Oskar Morgenstern)共同撰写了《游戏和经济行为理论》一书,该书考虑了几个参与者的合作游戏。这本书的第二版提供了预期效用的公理理论,使数理统计学家和经济学家能够处理不确定性下的决策。
微积分代写
微积分,最初被称为无穷小微积分或 “无穷小的微积分”,是对连续变化的数学研究,就像几何学是对形状的研究,而代数是对算术运算的概括研究一样。
它有两个主要分支,微分和积分;微分涉及瞬时变化率和曲线的斜率,而积分涉及数量的累积,以及曲线下或曲线之间的面积。这两个分支通过微积分的基本定理相互联系,它们利用了无限序列和无限级数收敛到一个明确定义的极限的基本概念 。
计量经济学代写
什么是计量经济学?
计量经济学是统计学和数学模型的定量应用,使用数据来发展理论或测试经济学中的现有假设,并根据历史数据预测未来趋势。它对现实世界的数据进行统计试验,然后将结果与被测试的理论进行比较和对比。
根据你是对测试现有理论感兴趣,还是对利用现有数据在这些观察的基础上提出新的假设感兴趣,计量经济学可以细分为两大类:理论和应用。那些经常从事这种实践的人通常被称为计量经济学家。
Matlab代写
MATLAB 是一种用于技术计算的高性能语言。它将计算、可视化和编程集成在一个易于使用的环境中,其中问题和解决方案以熟悉的数学符号表示。典型用途包括:数学和计算算法开发建模、仿真和原型制作数据分析、探索和可视化科学和工程图形应用程序开发,包括图形用户界面构建MATLAB 是一个交互式系统,其基本数据元素是一个不需要维度的数组。这使您可以解决许多技术计算问题,尤其是那些具有矩阵和向量公式的问题,而只需用 C 或 Fortran 等标量非交互式语言编写程序所需的时间的一小部分。MATLAB 名称代表矩阵实验室。MATLAB 最初的编写目的是提供对由 LINPACK 和 EISPACK 项目开发的矩阵软件的轻松访问,这两个项目共同代表了矩阵计算软件的最新技术。MATLAB 经过多年的发展,得到了许多用户的投入。在大学环境中,它是数学、工程和科学入门和高级课程的标准教学工具。在工业领域,MATLAB 是高效研究、开发和分析的首选工具。MATLAB 具有一系列称为工具箱的特定于应用程序的解决方案。对于大多数 MATLAB 用户来说非常重要,工具箱允许您学习和应用专业技术。工具箱是 MATLAB 函数(M 文件)的综合集合,可扩展 MATLAB 环境以解决特定类别的问题。可用工具箱的领域包括信号处理、控制系统、神经网络、模糊逻辑、小波、仿真等。