物理代写|PHYS3034 Quantum mechanics

MY-ASSIGNMENTEXPERT™可以为您提供sydney PHYS3034 Quantum mechanics量子力学课程的代写代考和辅导服务！

这是悉尼大学量子力学课程的代写成功案例。

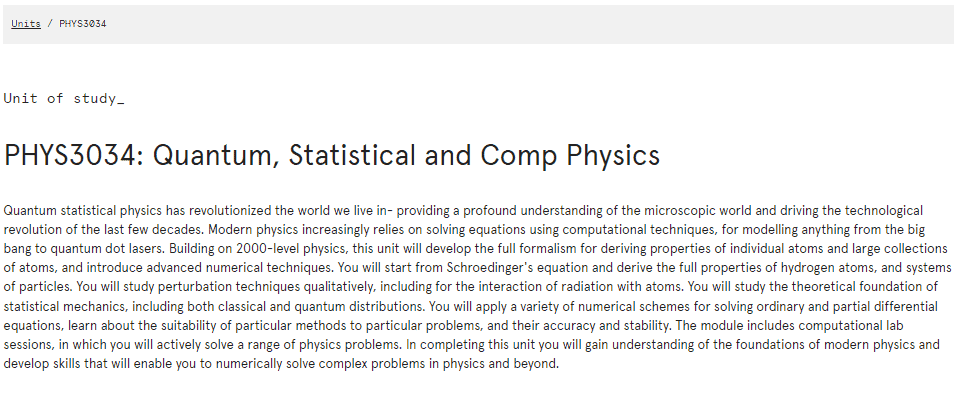
PHYS3034课程简介

Quantum statistical physics has revolutionized the world we live in- providing a profound understanding of the microscopic world and driving the technological revolution of the last few decades. Modern physics increasingly relies on solving equations using computational techniques, for modelling anything from the big bang to quantum dot lasers. Building on 2000-level physics, this unit will develop the full formalism for deriving properties of individual atoms and large collections of atoms, and introduce advanced numerical techniques. You will start from Schroedinger’s equation and derive the full properties of hydrogen atoms, and systems of particles. You will study perturbation techniques qualitatively, including for the interaction of radiation with atoms. You will study the theoretical foundation of statistical mechanics, including both classical and quantum distributions. You will apply a variety of numerical schemes for solving ordinary and partial differential equations, learn about the suitability of particular methods to particular problems, and their accuracy and stability. The module includes computational lab sessions, in which you will actively solve a range of physics problems. In completing this unit you will gain understanding of the foundations of modern physics and develop skills that will enable you to numerically solve complex problems in physics and beyond.

Prerequisites 

At the completion of this unit, you should be able to:

• LO1. demonstrate an understanding of key concepts in two foundation areas of physics – quantum mechanics of atoms and statistical physics
• LO2. apply these concepts to develop models, and to solve qualitative and quantitative problems in scientific contexts, using appropriate mathematical and computing techniques as necessary – Compare and critique different models in quantum and statistical physics
• LO3. design computer programs to solve physical problems
• LO4. compare and critique different approaches to numerically solving physical problems
• LO5. communicate scientific information appropriately, through written work
• LO6. analyse a physical problem in quantum physics and statistical physics and develop a formalism appropriate for solving it
• LO7. demonstrate a sense of responsibility, ethical behaviour, and independence as a learner and as a scientist.

PHYS3034 Quantum mechanics HELP（EXAM HELP， ONLINE TUTOR）

Solution: The rock starts out at rest, and picks up speed as it falls; it spends more time near the top, so the average distance will surely be less than $h / 2$. Ignoring air resistance, the distance $x$ at time $t$ is
$$
x(t)=\frac{1}{2} g t^2
$$

The velocity is $d x / d t=g t$, and the total flight time is $T=\sqrt{2 h / g}$. The probability that a particular photograph was taken between $t$ and $t+d t$ is $d t / T$, so the probability that it shows a distance in the corresponding range $x$ to $x+d x$ is
$$
\frac{d t}{T}=\frac{d x}{g t} \sqrt{\frac{g}{2 h}}=\frac{1}{2 \sqrt{h x}} d x \text {. }
$$
Thus the probability density (Equation 1.14) is
$$
\rho(x)=\frac{1}{2 \sqrt{h x}}, \quad(0 \leq x \leq h)
$$
(outside this range, of course, the probability density is zero).
We can check this result, using Equation 1.16:
$$
\int_0^h \frac{1}{2 \sqrt{h x}} d x=\left.\frac{1}{2 \sqrt{h}}\left(2 x^{1 / 2}\right)\right|_0 ^h=1
$$
The average distance (Equation 1.17) is
$$
\langle x\rangle=\int_0^h x \frac{1}{2 \sqrt{h x}} d x=\left.\frac{1}{2 \sqrt{h}}\left(\frac{2}{3} x^{3 / 2}\right)\right|_0 ^h=\frac{h}{3},
$$
which is somewhat less than $h / 2$, as anticipated.

Solution: The first part is easy:
$$
\Psi(x, t)=c_1 \psi_1(x) e^{-i E_1 t / \hbar}+c_2 \psi_2(x) e^{-i E_2 t / \hbar}
$$
where $E_1$ and $E_2$ are the energies associated with $\psi_1$ and $\psi_2$. It follows that
$$
\begin{aligned}
|\Psi(x, t)|^2 & =\left(c_1 \psi_1 e^{i E_1 t / \hbar}+c_2 \psi_2 e^{i E_2 t / \hbar}\right)\left(c_1 \psi_1 e^{-i E_1 t / \hbar}+c_2 \psi_2 e^{-i E_2 t / \hbar}\right) \
& =c_1^2 \psi_1^2+c_2^2 \psi_2^2+2 c_1 c_2 \psi_1 \psi_2 \cos \left[\left(E_2-E_1\right) t / \hbar\right] .
\end{aligned}
$$
The probability density oscillates sinusoidally, at an angular frequency $\omega=\left(E_2-E_1\right) / \hbar$; this is certainly not a stationary state. But notice that it took a linear combination of stationary states (with different energies) to produce motion

Solution: Using Equation 2.62,
$$
\begin{aligned}
\psi_1(x) & =A_1 \hat{a}{+} \psi_0=\frac{A_1}{\sqrt{2 \hbar m \omega}}\left(-\hbar \frac{d}{d x}+m \omega x\right)\left(\frac{m \omega}{\pi \hbar}\right)^{1 / 4} e^{-\frac{m \omega}{2 \hbar} x^2} \ & =A_1\left(\frac{m \omega}{\pi \hbar}\right)^{1 / 4} \sqrt{\frac{2 m \omega}{\hbar}} x e^{-\frac{m \omega}{2 \hbar} x^2} \end{aligned} $$ We can normalize it “by hand”: $$ \begin{aligned} & \int\left|\psi_1\right|^2 d x=\left|A_1\right|^2 \sqrt{\frac{m \omega}{\pi \hbar}}\left(\frac{2 m \omega}{\hbar}\right) \int{-\infty}^{\infty} x^2 e^{-\frac{m \omega}{\hbar} x^2} d x=\left|A_1\right|^2, \
& \text { so, as it happens, } A_1=1 .
\end{aligned}
$$
I wouldn’t want to calculate $\psi_{50}$ this way (applying the raising operator fifty times!), but never mind: In principle Equation 2.62 does the job-except for the normalization.

Solution:
$$
\langle V\rangle=\left\langle\frac{1}{2} m \omega^2 x^2\right\rangle=\frac{1}{2} m \omega^2 \int_{-\infty}^{\infty} \psi_n^* x^2 \psi_n d x
$$
There’s a beautiful device for evaluating integrals of this kind (involving powers of $x$ or $\hat{p}$ ): Use the definition (Equation 2.48) to express $x$ and $\hat{p}$ in terms of the raising and lowering operators:
$$
x=\sqrt{\frac{\hbar}{2 m \omega}}\left(\hat{a}{+}+\hat{a}{-}\right) ; \quad \hat{p}=i \sqrt{\frac{\hbar m \omega}{2}}\left(\hat{a}{+}-\hat{a}{-}\right) .
$$
In this example we are interested in $x^2$
$$
x^2=\frac{\hbar}{2 m \omega}\left[\left(\hat{a}{+}\right)^2+\left(\hat{a}{+} \hat{a}{-}\right)+\left(\hat{a}{-} \hat{a}{+}\right)+\left(\hat{a}{-}\right)^2\right] \text {. }
$$
So
$$
\langle V\rangle=\frac{\hbar \omega}{4} \int_{-\infty}^{\infty} \psi_n^*\left[\left(\hat{a}{+}\right)^2+\left(\hat{a}{+} \hat{a}{-}\right)+\left(\hat{a}{-} \hat{a}{+}\right)+\left(\hat{a}{-}\right)^2\right] \psi_n d x
$$

$\operatorname{But}\left(\hat{a}{+}\right)^2 \psi_n$ is (apart from normalization) $\psi{n+2}$, which is orthogonal to $\psi_n$, and the same goes for $\left(\hat{a}{-}\right)^2 \psi_n$, which is proportional to $\psi{n-2}$. So those terms drop out, and we can use Equation 2.66 to evaluate the remaining two:
$$
\langle V\rangle=\frac{\hbar \omega}{4}(n+n+1)=\frac{1}{2} \hbar \omega\left(n+\frac{1}{2}\right)
$$
As it happens, the expectation value of the potential energy is exactly half the total (the other half, of course, is kinetic). This is a peculiarity of the harmonic oscillator, as we’ll see later on (Problem $3.37$ ).

MY-ASSIGNMENTEXPERT™可以为您提供SYDNEY PHYS3034 QUANTUM MECHANICS量子力学课程的代写代考和辅导服务！