数学代写|MS-C1342 Linear Algebra

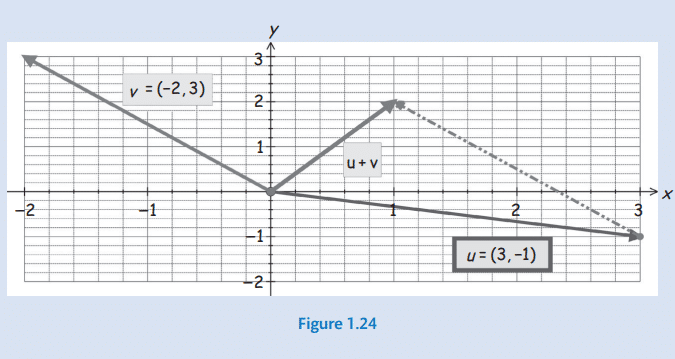
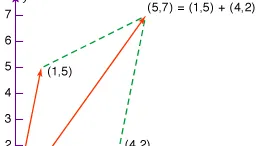
MY-ASSIGNMENTEXPERT™可以为您提供mycourses MS-C1342 Linear Algebra线性代数的代写代考和辅导服务！

这是阿尔托大学线性代数课程的代写成功案例。

MS-C1342课程简介

There are two options. A student can either return homework exercises and attend a final exam, or just attend the exam. In the first option, the homework and the exam are weighted at 40 and 60 percent respectively; in the second option, the final mark is 100 percent from the exam. As the final exam will be more difficult than the homework, the first option is highly recommended.

The first option is only valid for the course exam (first exam right after the course). In later examinations, only the 100 percent exam option is available.

Exceptions to this rule can be granted only if a student was forced to miss the course exam for impediments clearly beyond the student’s control. A summer job is not considered an impediment beyond the student’s control: generally, it is the student’s responsibility to only start a summer job after all courses and exams are over, or alternatively to negotiate time off with the employer.

Prerequisites 

• Period V: 24 April – 9 June 2023
• Lecturer: Vanni Noferini, vanni dot noferini at aalto dot fi
• Head assistant: Juha-Pekka Puska, juha hyphen pekka dot puska at aalto dot fi
• Registration in Sisu
• In 2023 lectures and exercise sessions will be held in person at the Aalto campus.
• Instructions for the exercises are in the Assignments section.

MS-C1342 Linear algebra HELP（EXAM HELP， ONLINE TUTOR）

Solution
Since $\mathbf{u}=\mathbf{v}$ we have
$$
\begin{aligned}
& x-3=1 \text { gives } x=4 \
& y+1=2 \text { gives } y=1 \
& z+x=3 \text { gives } z+4=3 \text { implies } z=-1 \
&
\end{aligned}
$$
Our solution is $x=4, y=1$ and $z=-1$.

Solution
(a) Multiplying each entry of the matrix by 10 gives
$$
10\left(\begin{array}{ll}
1 & 2 \
3 & 4
\end{array}\right)=\left(\begin{array}{ll}
1 \times 10 & 2 \times 10 \
3 \times 10 & 4 \times 10
\end{array}\right)=\left(\begin{array}{ll}
10 & 20 \
30 & 40
\end{array}\right)
$$
(b) Multiplying each element in the second matrix by $1 / 2$ (or dividing by 2 ) gives
$$
\frac{1}{2}\left(\begin{array}{ccccc}
1 & 2 & 5 & 6 & 1 \
7 & 9 & 11 & 6 & 3
\end{array}\right)=\left(\begin{array}{ccccc}
0.5 & 1 & 2.5 & 3 & 0.5 \
3.5 & 4.5 & 5.5 & 3 & 1.5
\end{array}\right)
$$

Solution
First we find $\mathbf{B}+\mathbf{C}=\left(\begin{array}{ll}1 & 2 \ 3 & 4\end{array}\right)+\left(\begin{array}{ll}5 & 6 \ 7 & 8\end{array}\right)=\left(\begin{array}{cc}6 & 8 \ 10 & 12\end{array}\right)$. Next we left multiply this by matrix $\mathbf{A}$ :
$$
\mathbf{A}(\mathbf{B}+\mathbf{C})=\left(\begin{array}{ll}
2 & 3 \
6 & 7 \
1 & 2
\end{array}\right)\left(\begin{array}{cc}
6 & 8 \
10 & 12
\end{array}\right)=\left(\begin{array}{cc}
42 & 52 \
106 & 132 \
26 & 32
\end{array}\right)
$$
Similarly, we can show $\mathbf{A B}+\mathbf{A C}$ gives the same result. In this case, we have
$$
\mathbf{A}(\mathbf{B}+\mathbf{C})=\mathbf{A B}+\mathbf{A C}
$$

Solution
(a) Multiplying the bracket term first:
$$
\begin{gathered}
(A B)=\left(\begin{array}{ll}
1 & 2 \
9 & 7
\end{array}\right)\left(\begin{array}{rrr}
2 & 3 & -1 \
5 & -7 & 6
\end{array}\right) \
=\left(\begin{array}{rrr}
12 & -11 & 11 \
53 & -22 & 33
\end{array}\right) \
\left(\text { AB) } C=\left(\begin{array}{rrr}
12 & -11 & 11 \
53 & -22 & 33
\end{array}\right)\left(\begin{array}{r}
2 \
-5 \
9
\end{array}\right)=\left(\begin{array}{l}
178 \
513
\end{array}\right)\right.
\end{gathered}
$$
(b) By Theorem (1.16) (a) we have (AB) $\mathbf{C}=\mathbf{A}$ (BC), therefore we have the answer of part (a).
(c) Matrices $\mathbf{B}$ and $\mathbf{C}$ are of different size, therefore we cannot add them. That is we cannot evaluate $\mathbf{B}+\mathbf{C}$ which means $\mathbf{A}(\mathbf{B}+\mathbf{C})$ is impossible.
(d) By Theorem (1.16) (b) we have $\mathbf{A B}+\mathbf{A C}=\mathbf{A}(\mathbf{B}+\mathbf{C})$, therefore we also cannot evaluate $\mathbf{A B}+\mathbf{A C}$ because we cannot add $\mathbf{B}$ and $\mathbf{C}$.
(e) As part (c) above, we cannot add matrices B and C. Hence we cannot evaluate (B + C) A. For the remaining parts, we use Theorem (1.16) (d) which says that
$$
\mathrm{A} \times \mathrm{O}=\mathrm{O} \times \mathbf{A}=\mathbf{O}
$$
For parts (f) and (g) we have
$$
\begin{aligned}
& \mathbf{A O}{22}=\left(\begin{array}{ll} 1 & 2 \ 9 & 7 \end{array}\right)\left(\begin{array}{ll} 0 & 0 \ 0 & 0 \end{array}\right)=\left(\begin{array}{ll} 0 & 0 \ 0 & 0 \end{array}\right), \ & \mathbf{B O}{33}=\left(\begin{array}{rrr}
2 & 3 & -1 \
5 & -7 & 6
\end{array}\right)\left(\begin{array}{lll}
0 & 0 & 0 \
0 & 0 & 0 \
0 & 0 & 0
\end{array}\right)=\left(\begin{array}{lll}
0 & 0 & 0 \
0 & 0 & 0
\end{array}\right)
\end{aligned}
$$
(h) We have $\mathbf{C O}_{13}=\left(\begin{array}{r}2 \ -5 \ 9\end{array}\right)\left(\begin{array}{lll}0 & 0 & 0\end{array}\right)=(0)=0$.

MY-ASSIGNMENTEXPERT™可以为您提供mycourses MS-C1342 Linear Algebra线性代数的代写代考和辅导服务！