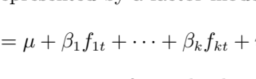物理代考| Component Form 量子力学代写
物理代写
$9.2$ Component Form
Let us start with the component form of our relations. In order to do this we need to introduce the concept of a continuous component, and we also need to make use of the Dirac delta function that was introduced when discussing transition rates. In fact, the concepts and notation for what we are doing here were originally introduced by Dirac in his fundamental work. ${ }^{1}$
We first label the components of the abstract state vectors that we are studying with a subscript $x$, and calculate the inner product of two of those vectors as $^{2}$
$$
\left\langle\psi_{m} \mid \psi_{n}\right\rangle=\sum_{x}\left(\psi_{m}\right){x}^{}\left(\psi{n}\right){x} $$ We now have to define the continuous sum, as well as the components in the $x$-direction. We do this by writing the sum as an integral and using our coordinate-space wave functions for the components $$ \left\langle\psi{m} \mid \psi_{n}\right\rangle=\sum_{x}\left(\psi_{m}\right){x}^{}\left(\psi{n}\right){x} \equiv \int d x \psi{m}^{}(x) \psi_{n}(x) $$ This defines the continuous sum. It is just the integral over the appropriate interval of two of our previous wave functions. ${ }^{1}$ See [Dirac (1930)]. antum Mechanics Formal Structure of Quantum Mechanics introduce the eigenstates of the hermitian position oper- Formal Structure of Quantum We can then rewrite the above as $$ \left\langle\psi_{m} \mid \psi_{n}\right\rangle=\sum_{x}\left(\psi_{m}\right){x}^{}\left(\psi{n}\right){x}=\sum{x}\left\langle\psi_{m} \mid x\right\rangle\left\langle x \mid \psi_{n}\right\rangle
$$
where we have identified the wave functions as
$$
\begin{aligned}
\left\langle x \mid \psi_{n}\right\rangle &=\psi_{n}(x) \
\left\langle\psi_{m} \mid x\right\rangle &=\psi_{m}^{*}(x)
\end{aligned}
$$
We now have the consistent physical interpretation that the probability for finding the particle in the interval $d x$ at the position $x$ if it is in the state $\left|\psi_{n}\right\rangle$ is the absolute square of the probability amplitude obtained from the inner product $\left\langle x \mid \psi_{n}\right\rangle$
$$ \left|\left\langle x \mid \psi_{n}\right\rangle\right|^{2} d x=\left|\psi_{n}(x)\right|^{2} d x \quad ; \text { probability } $$ Equation (9.7) allows us to identify the completeness relation for the eigenstates of position $$ \sum_{x}|x\rangle\langle x|=\hat{1} $$
What about the inner product of these states? Here we are forced to deal with the fact that the eigenvalues of position are truly continuous, and we write $^{4}$
物理代考
$9.2$ 组件表格
让我们从关系的组件形式开始。为了做到这一点,我们需要引入连续分量的概念,并且我们还需要利用在讨论转换率时引入的 Dirac delta 函数。事实上,我们在这里所做的事情的概念和符号最初是由狄拉克在他的基础著作中引入的。 ${ }^{1}$
我们首先用下标 $x$ 标记我们正在研究的抽象状态向量的分量,然后将其中两个向量的内积计算为 $^{2}$
$$
\left\langle\psi_{m} \mid \psi_{n}\right\rangle=\sum_{x}\left(\psi_{m}\right){x}^{}\left(\psi {n}\右){x} $$ 我们现在必须定义连续和,以及 $x$ 方向的分量。我们通过将总和写成积分并使用我们的坐标空间波函数来实现这一点 $$ \left\langle\psi{m} \mid \psi_{n}\right\rangle=\sum_{x}\left(\psi_{m}\right){x}^{}\left(\psi {n}\right){x} \equiv \int dx \psi{m}^{}(x) \psi_{n}(x) $$ 这定义了连续总和。它只是我们之前的两个波函数在适当区间上的积分。 ${ }^{1}$ 见 [狄拉克 (1930)]。 安腾力学 量子力学的形式结构介绍了厄米位置运算的本征态 量子的形式结构 然后我们可以将上面的内容重写为 $$ \left\langle\psi_{m} \mid \psi_{n}\right\rangle=\sum_{x}\left(\psi_{m}\right){x}^{}\left(\psi {n}\right){x}=\sum{x}\left\langle\psi_{m} \mid x\right\rangle\left\langle x \mid \psi_{n}\right\rangle
$$
我们已经将波函数确定为
$$
\开始{对齐}
\left\langle x \mid \psi_{n}\right\rangle &=\psi_{n}(x) \
\left\langle\psi_{m} \mid x\right\rangle &=\psi_{m}^{*}(x)
\end{对齐}
$$
我们现在有了一致的物理解释,如果粒子处于状态 $\left|\psi_{n}\right\rangle$,则在区间 $dx$ 中位置 $x$ 找到粒子的概率是绝对平方由内积 $\left\langle x \mid \psi_{n}\right\rangle$ 得到的概率幅值
$$ \left|\left\langle x \mid \psi_{n}\right\rangle\right|^{2} dx=\left|\psi_{n}(x)\right|^{2} dx \四边形; \text { probability } $$ 方程(9.7)允许我们识别位置 $$ \sum_{x}|x\rangle\langle x|=\hat{1} $$ 的特征态的完整性关系
这些状态的内积呢?这里我们不得不处理 position 的特征值是真正连续的,我们写成 $^{4}$

物理代考| Classical Optics量子力学代写 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。
电磁学代考
物理代考服务:
物理Physics考试代考、留学生物理online exam代考、电磁学代考、热力学代考、相对论代考、电动力学代考、电磁学代考、分析力学代考、澳洲物理代考、北美物理考试代考、美国留学生物理final exam代考、加拿大物理midterm代考、澳洲物理online exam代考、英国物理online quiz代考等。
光学代考
光学(Optics),是物理学的分支,主要是研究光的现象、性质与应用,包括光与物质之间的相互作用、光学仪器的制作。光学通常研究红外线、紫外线及可见光的物理行为。因为光是电磁波,其它形式的电磁辐射,例如X射线、微波、电磁辐射及无线电波等等也具有类似光的特性。
大多数常见的光学现象都可以用经典电动力学理论来说明。但是,通常这全套理论很难实际应用,必需先假定简单模型。几何光学的模型最为容易使用。
相对论代考
上至高压线,下至发电机,只要用到电的地方就有相对论效应存在!相对论是关于时空和引力的理论,主要由爱因斯坦创立,相对论的提出给物理学带来了革命性的变化,被誉为现代物理性最伟大的基础理论。
流体力学代考
流体力学是力学的一个分支。 主要研究在各种力的作用下流体本身的状态,以及流体和固体壁面、流体和流体之间、流体与其他运动形态之间的相互作用的力学分支。
随机过程代写
随机过程,是依赖于参数的一组随机变量的全体,参数通常是时间。 随机变量是随机现象的数量表现,其取值随着偶然因素的影响而改变。 例如,某商店在从时间t0到时间tK这段时间内接待顾客的人数,就是依赖于时间t的一组随机变量,即随机过程