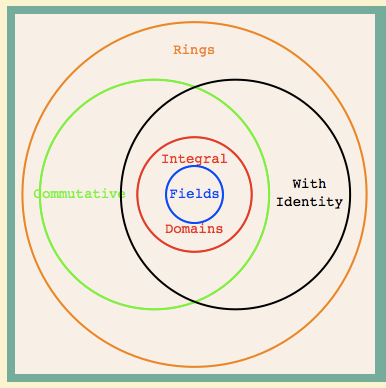
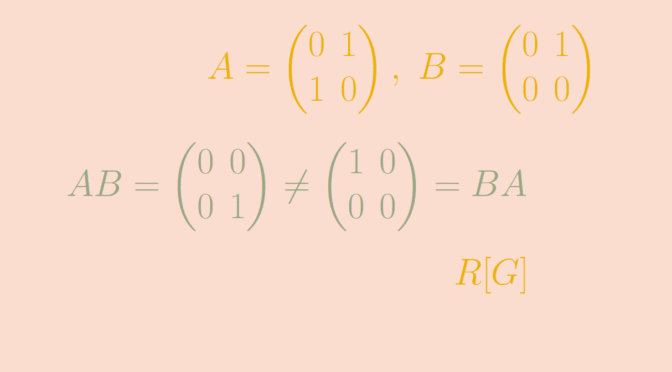如果你也在 怎样代写交换代数Commutative Algebra MATH483这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。交换代数Commutative Algebra换元代数的许多其他概念是代数几何中出现的几何概念的对应物。这就是Krull维度、主分解、正则环、Cohen-Macaulay环、Gorenstein环和许多其他概念的情况。
交换代数Commutative Algebra这门学科最初被称为理想理论,始于理查德-戴德金关于理想的工作,其本身是基于恩斯特-库默尔和利奥波德-克罗内克的早期工作。后来,大卫-希尔伯特(David Hilbert)引入了环这个术语,以概括早期的数环术语。希尔伯特引入了一种更抽象的方法,以取代基于复数分析和经典不变理论的更具体和面向计算的方法。反过来,希尔伯特也强烈地影响了埃米-诺特,他用一个升链条件(现在称为诺特条件)来重塑许多早期的结果。另一个重要的里程碑是希尔伯特的学生伊曼纽尔-拉斯克的工作,他引入了初级理想并证明了拉斯克-诺特定理的第一个版本。
交换代数Commutative Algebra代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。最高质量的交换代数Commutative Algebra作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此交换代数Commutative Algebra作业代写的价格不固定。通常在专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
同学们在留学期间,都对各式各样的作业考试很是头疼,如果你无从下手,不如考虑my-assignmentexpert™!
my-assignmentexpert™提供最专业的一站式服务:Essay代写,Dissertation代写,Assignment代写,Paper代写,Proposal代写,Proposal代写,Literature Review代写,Online Course,Exam代考等等。my-assignmentexpert™专注为留学生提供Essay代写服务,拥有各个专业的博硕教师团队帮您代写,免费修改及辅导,保证成果完成的效率和质量。同时有多家检测平台帐号,包括Turnitin高级账户,检测论文不会留痕,写好后检测修改,放心可靠,经得起任何考验!
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
我们在数学Mathematics代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在Matlab代写方面经验极为丰富,各种Matlab相关的作业也就用不着说。
数学代写|交换代数代考Commutative Algebra代写|Primary decomposition
The characterization of a primary submodule in terms of condition (iv) of Proposition 5.2.13 seems to have slipped E. Noether’s mind, who wrote in this regard: “As well the definition of primary and prime ideal cannot be transported to modules since the product of two quantities (elements) is not defined.” ([116, Section 9, p. 56]) In spite of the fact that the notion of the quotient was sufficiently known and largely used by Noether, the idea of a zero-divisor on a module does not seem to be fully undertaken at the time. In this book, as well as in most books on the subject, the argument in the proof of Theorem 5.2.17 is elegantly abstract. The original argument of Lasker and Noether, in the case of ideals, went through the consideration of an irreducible decomposition rather than a primary one. Actually, Noether dedicated quite some space for such a consideration, with many examples throughout. Perhaps the reason irreducible decomposition essentially faded out in modern exposés is the lack of uniqueness or of a stable number of components. Thus, from a decomposition into irreducible components one passes to a primary normally nonirreducible decomposition by clipping together primary submodules with same radical-the main step in reaching a reduced primary decomposition.
数学代写|交换代数代考Commutative Algebra代写|Hilbert and Artin
Theorem 2.5.4 was proved by David Hilbert in Über die Theorie der algebraischen Formen, Mathematische Annalen, 36 (1890) 473-534. The proof given here is reminiscent of the original argument given by Hilbert with the enormous time gap making Hilbert unaware of the theory of Noetherian rings (only much later fully entrenched by E. Noether). In its original form, he took $R$ to be a polynomial ring over a field in finitely many variables and proceeded by induction on the number of variables, showing that any homogeneous ideal is finitely generated. The proof given in Theorem 2.5.4 stays close to Hilbert’s own argument by choosing an infinitely countable set of elements in the given ideal. Hilbert himself was quite aware that this hypothesis was not quite in the generality he wished. In fact, he proceeded in the same paper to explain how one switches from such a countable set to the case of an arbitrary ideal (then called module in the language of Kronecker). For that, he assumed without further ado the axiom of choice as a self-granted hypothesis.
Also, Hilbert’s original theorem restricted himself to the case of homogeneous polynomials (the German Formen). Nonetheless, his argument remains essentially valid for any polynomials. The reason behind this restriction is that he was really interested in the applications to the theory of invariants, a much deeper work he took over in the sequel.
The proof of Theorem 2.5.14 came after a long period of preliminary work both in the commutative as in the noncommutative situation. The idea of the length of a module crystallized slowly from the corresponding notion for finite groups-a clear statement of the main facts was given by E. Noether in 1926 ([118, Section 10]) for the purpose of establishing a version of Theorem 2.5.13.
Now, the implication that an Artin ring is Noetherian had to somehow be firmly grounded on properties of rings alien to modules for the simple fact that there exist nonfinitely generated Artinian modules (similar definition)-in fact, the usual local cohomology modules are of this nature. Thus, if one takes for granted the theory of Jordan-Hölder warranting that any two composition series have the same length and this length is by definition the length of $R$ (more generally, this is defined for a module), an alternative argument goes as follows: let $I_{1} \subset I_{2} \subset \cdots$ be an ascending chain of ideals in $R$. Then one has a sequence of surjective ring homomorphisms $R / I_{1} \rightarrow R / I_{2} \rightarrow \cdots$. As noted, for any ideal $I \subset R, R / I$ is Artinian as well. By the additivity property (here one needs the notion of length for a module as well) the length of $R / I_{i}$ is strictly larger than the one of $R / I_{i+1}$. Since $R / I_{1}$ has finite length, then $R / I_{i}=R / I_{i+1}$ for sufficiently large $i$. This makes the original chain of ideals stationary.
交换代数代写
数学代写|交换代数代考COMMUTATIVE ALGEBRA代 写|PRIMARY DECOMPOSITION
命题5.2.13的条件(iv)对初级子模的描述似乎忘了E.Noether的想法,他在这方面写道。”同样,主理想和质理想的定义也不能转移到模块上,因为两个量(元素)的乘积没有定义。” ([116,第9节,第56页])尽管商的概念已被诺特充分了解并在很大程度上被使用,但模块上的零除数的概念在当时似乎并没有被完全采纳。在这本书中,以及在大多数关于这个问题的书中,定理5.2.17的证明中的论证是优雅抽象的。拉斯克和诺特的原始论证,在理想的情况下,通过考虑不可还原的分解而不是主要的分解。实际上,诺特用了相当多的篇幅来考虑这样的问题,通篇有许多例子。也许不可还原的分解在现代的论述中基本淡出的原因是缺乏唯一性或稳定的成分数。因此,从一个分解为不可还原的成分,我们可以通过将具有相同基数的初级子模剪在一起,转为一个通常是不可还原的初级分解–这是达到还原初级分解的主要步骤。
数学代写|交换代数代考COMMUTATIVE ALGEBRA代 写|HILBERT AND ARTIN
定理 2.5.4 由 David Hilbert 在 On the Theory of Algebraic Forms, Mathematical Annals, 36 中证明 $1890473-534$ 。这里给出的证明让人想起希尔伯特给出的原始论证,巨 大的时间间隔使希尔伯特不知道诺特环理论onlymuchlaterfullyentrenchedbyE. Noether. 他以原来的形式 $R$ 是有限多个变量的域上的多项式环,并通过对变 量数的归纳进行,表明任何齐次理想都是有限生成的。定理 $2.5 .4$ 中给出的证明通过在给定理想中选择无限可数的元表集来接近希尔伯特自己的论点。希尔伯特本 人很清楚,这个假设并不完全符合他所希望的普遍性。事实上,他在同一篇论文中继续解释了如何从这样的可数集切换到任意理想的情况 thencalledmoduleinthelanguageofKronecker. 为此,他靟不牴力地假设选择公理是一个自以为是的假设。
此外,莃尔伯特的原定理仅限于齐次多项式的情况theGermanFormen. 尽管如此,他的论点对任何多项式仍然基本有效。䢒种限制背后的原因是他对不变量理 论的应用非常感兴趣,这是他在续集中接手的更深入的工作。
定理 $2.5 .14$ 的证明是在对可交换和非对易情况的长期初步工作之后得出的。模长度的概念从相应的有限群概念中慢慢结晶出来一一E. Noether 在 1926 年对主要事实 做出了清晰的陈述 $[118$, Section 10$]$ 为了建立定理 $2.5 .13$ 的版本。
现在,Artin 环是 Noetherian 的暗示必须以某种方式牢固地基于与模块不同的环的属性,因为存在非无限生成的 Artinian 模块这一简单事实similardefinition-事 实上,通常的局部上同调模块就是这种性质。因此,如果人们认为 Jordan-Hölder 的理论保证任何两个组合序列具有相同的长度并且这个长度根据定义是 $R$. moregenerally, thisisdefinedforamodule,另一种说法如下: $I_{1} \subset I_{2} \subset \cdots$ 成为一个上升的理想链 $R$. 那么一个有一系列满射环同态 $R / I_{1} \rightarrow R / I_{2} \rightarrow \cdots$. 如前所述,对于任何理想 $I \subset R, R / I$ 也是Artinian。由可加性性质hereoneneedsthenotionoflengthforamoduleaswell的长度 $R / I_{i}$ 严格大于 $R / I_{i+1}$. 自从 $R / I_{1}$ 有有限的长度,那么 $R / I_{i}=R / I_{i+1}$ 对于足够大 $i$. 这使得最初的理想链静止。

数学代写|交换代数代考Commutative Algebra代写 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。
微观经济学代写
微观经济学是主流经济学的一个分支,研究个人和企业在做出有关稀缺资源分配的决策时的行为以及这些个人和企业之间的相互作用。my-assignmentexpert™ 为您的留学生涯保驾护航 在数学Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在图论代写Graph Theory代写方面经验极为丰富,各种图论代写Graph Theory相关的作业也就用不着 说。
线性代数代写
线性代数是数学的一个分支,涉及线性方程,如:线性图,如:以及它们在向量空间和通过矩阵的表示。线性代数是几乎所有数学领域的核心。
博弈论代写
现代博弈论始于约翰-冯-诺伊曼(John von Neumann)提出的两人零和博弈中的混合策略均衡的观点及其证明。冯-诺依曼的原始证明使用了关于连续映射到紧凑凸集的布劳威尔定点定理,这成为博弈论和数学经济学的标准方法。在他的论文之后,1944年,他与奥斯卡-莫根斯特恩(Oskar Morgenstern)共同撰写了《游戏和经济行为理论》一书,该书考虑了几个参与者的合作游戏。这本书的第二版提供了预期效用的公理理论,使数理统计学家和经济学家能够处理不确定性下的决策。
微积分代写
微积分,最初被称为无穷小微积分或 “无穷小的微积分”,是对连续变化的数学研究,就像几何学是对形状的研究,而代数是对算术运算的概括研究一样。
它有两个主要分支,微分和积分;微分涉及瞬时变化率和曲线的斜率,而积分涉及数量的累积,以及曲线下或曲线之间的面积。这两个分支通过微积分的基本定理相互联系,它们利用了无限序列和无限级数收敛到一个明确定义的极限的基本概念 。
计量经济学代写
什么是计量经济学?
计量经济学是统计学和数学模型的定量应用,使用数据来发展理论或测试经济学中的现有假设,并根据历史数据预测未来趋势。它对现实世界的数据进行统计试验,然后将结果与被测试的理论进行比较和对比。
根据你是对测试现有理论感兴趣,还是对利用现有数据在这些观察的基础上提出新的假设感兴趣,计量经济学可以细分为两大类:理论和应用。那些经常从事这种实践的人通常被称为计量经济学家。
Matlab代写
MATLAB 是一种用于技术计算的高性能语言。它将计算、可视化和编程集成在一个易于使用的环境中,其中问题和解决方案以熟悉的数学符号表示。典型用途包括:数学和计算算法开发建模、仿真和原型制作数据分析、探索和可视化科学和工程图形应用程序开发,包括图形用户界面构建MATLAB 是一个交互式系统,其基本数据元素是一个不需要维度的数组。这使您可以解决许多技术计算问题,尤其是那些具有矩阵和向量公式的问题,而只需用 C 或 Fortran 等标量非交互式语言编写程序所需的时间的一小部分。MATLAB 名称代表矩阵实验室。MATLAB 最初的编写目的是提供对由 LINPACK 和 EISPACK 项目开发的矩阵软件的轻松访问,这两个项目共同代表了矩阵计算软件的最新技术。MATLAB 经过多年的发展,得到了许多用户的投入。在大学环境中,它是数学、工程和科学入门和高级课程的标准教学工具。在工业领域,MATLAB 是高效研究、开发和分析的首选工具。MATLAB 具有一系列称为工具箱的特定于应用程序的解决方案。对于大多数 MATLAB 用户来说非常重要,工具箱允许您学习和应用专业技术。工具箱是 MATLAB 函数(M 文件)的综合集合,可扩展 MATLAB 环境以解决特定类别的问题。可用工具箱的领域包括信号处理、控制系统、神经网络、模糊逻辑、小波、仿真等。