如果你也在 怎样代写电路设计Filter Design这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。电路设计Filter Design是设计一个满足一系列要求的信号处理滤波器的过程,其中一些要求可能是相互冲突的。其目的是找到一个能充分满足每项要求的滤波器,使其发挥作用。
电路设计Filter Design过程可以被描述为一个优化问题,其中每个要求都有助于一个应该被最小化的误差函数。设计过程的某些部分可以自动化,但通常需要有经验的电气工程师来获得一个好的结果。是一个具有欺骗性的复杂课题。尽管电路设计很容易理解和计算,但其设计和实现的实际挑战是巨大的,是高级研究的主题。
my-assignmentexpert™ 电路设计Filter Design作业代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。my-assignmentexpert™, 最高质量的电路设计Filter Design作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于统计Statistics作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此电路设计Filter Design作业代写的价格不固定。通常在经济学专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
my-assignmentexpert™ 为您的留学生涯保驾护航 在EE作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的电路设计Filter Design代写服务。我们的专家在EE代写方面经验极为丰富,各种电路设计Filter Design相关的作业也就用不着 说。
我们提供的电路设计Filter Design及其相关学科的代写,服务范围广, 其中包括但不限于:
EE代写|电路设计作业代写Filter Design代考|Lowpass Circuits
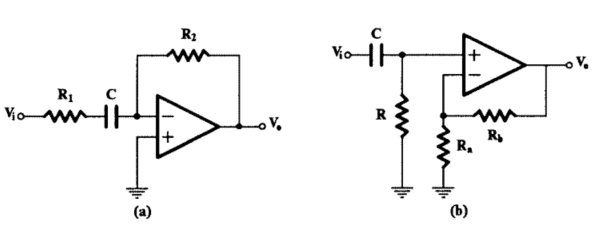
A first-order lowpass circuit is shown in Fig. 4.1(a). Its transfer voltage ratio can easily be shown to be
$$
H(s) \equiv \frac{V_{o}}{V_{i}}=-\frac{1 / C R_{1}}{s+1 / C R_{2}}
$$
Thus, the circuit can realize the transfer function
$$
F(s)=\frac{a}{s+b}
$$
with
$$
a=-\frac{1}{C R_{1}}, b=\frac{1}{C R_{2}}
$$
The de gain is
$$
\frac{a}{b}=-\frac{R_{2}}{R_{1}}
$$
and can be adjusted to any desired practical value.
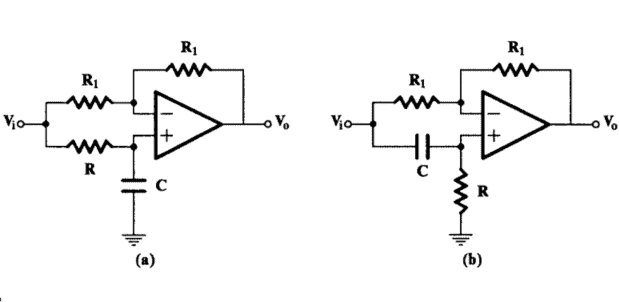
EE代写|电路设计作业代写Filter Design代考|Highpass Circuits
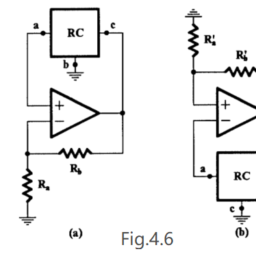
Both circuits in Fig. $4.2$ can realize the first-order highpass function
$$
F(s)=\frac{a s}{s+b}
$$
The transfer function of the circuit in Fig. 4.2(a) is
$$
H(s)=-\frac{R_{2}}{R_{1}} \frac{s}{s+1 / C R_{1}}
$$
Therefore, following coefficient matching, we get from Eqs. (4.5) and (4.6) the design equations as follows:
$$
a=-\frac{R_{2}}{R_{1}}, \quad b=\frac{1}{C R_{1}}
$$
Clearly, the value of one component will have to be selected arbitrarily.
Similarly, the transfer function of the circuit in Fig. 4.2(b) is
$$
H(s)=\frac{K s}{s+1 /(R C)}
$$
and, after coefficient matching, we obtain
$$
a=k=1+\frac{R_{b}}{R_{a}}, \quad b=\frac{1}{C R}
$$
Here, two components should have their values arbitrarily selected.
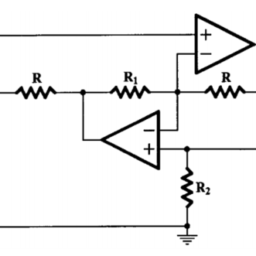
EE代写|电路设计作业代写FILTER DESIGN代考|Allpass Circuits
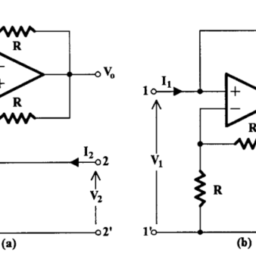
Two circuits [1] suitable for the realization of the first-order allpass function,
$$
F(s)=\frac{s-a}{s+a}
$$
are shown in Fig. 4.3.
The transfer function of the circuit in Fig. 4.3(a) is the following:
$$
H(s)=\frac{V_{o}}{V_{i}}=-\frac{s-1 / C R}{s+1 / C R}
$$
The same holds for the circuit in Fig. 4.3(b), but without the negative sign in front. For both circuits, coefficient matching gives the following design relationship:
$$
a=\frac{1}{C R}
$$
电路设计代写
EE代写|电路设计作业代写FILTER DESIGN代考|LOWPASS CIRCUITS
一阶低通电路如图 4.1 所示一种. 它的传输电压比可以很容易地表示为
$$
H(s) \equiv \frac{V_{o}}{V_{i}}=-\frac{1 / C R_{1}}{s+1 / C R_{2}}
$$
因此,电路可以实现传递函数
$$
F(s)=\frac{a}{s+b}
$$
增益为
$$
F(s)=\frac{a}{s+b}
$$
with
$$
a=-\frac{1}{C R_{1}}, b=\frac{1}{C R_{2}}
$$
The de gain is
$$
\frac{a}{b}=-\frac{R_{2}}{R_{1}}
$$
并且可以调整到任何所需的实用值。
EE代写|电路设计作业代写FILTER DESIGN代考|HIGHPASS CIRCUITS
图 2 中的两个电路4.2可以实现一阶高通函数
$$
F(s)=\frac{a s}{s+b}
$$
图 4.2 电路的传递函数一种是
$$
H(s)=-\frac{R_{2}}{R_{1}} \frac{s}{s+1 / C R_{1}}
$$
因此,在系数匹配之后,我们从方程得到。4.5和4.6设计方程如下:
$$
a=-\frac{R_{2}}{R_{1}}, \quad b=\frac{1}{C R_{1}}
$$
显然,必须任意选择一个组件的值。
类似地,图 4.2 中电路的传递函数b是
$$
H(s)=\frac{K s}{s+1 /(R C)}
$$
并且,在系数匹配之后,我们得到
$$
a=k=1+\frac{R_{b}}{R_{a}}, \quad b=\frac{1}{C R}
$$
这里,两个组件的值应该任意选择。
EE代写|电路设计作业代写FILTER DESIGN代考|ALLPASS CIRCUITS
两个电路1适合实现一阶全通功能,
$$
F(s)=\frac{s-a}{s+a}
$$
如图 4.3 所示。
图 4.3 电路的传递函数一种如下:
$$
H(s)=\frac{V_{o}}{V_{i}}=-\frac{s-1 / C R}{s+1 / C R}
$$
图 4.3 中的电路也是如此b,但前面没有负号。对于这两种电路,系数匹配给出以下设计关系:
$$
a=\frac{1}{C R}
$$

EE代写 |电路设计作业代写Filter Design代考 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。