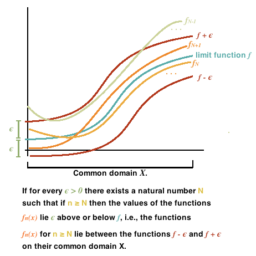
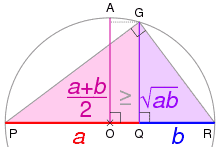
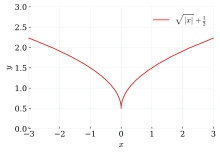There is an interesting rule which is often useful for evaluating difficult limits called L’Hôpital’s $^{\top}$ rule. The best versions of this rule are based on the Cauchy Mean value theorem, Theorem $7.8 .2$ on Page 141 .
Theorem $8.8 .1$ Let $[a, b] \subseteq[-\infty, \infty]$ and suppose $f, g$ are functions which satisfy,
$$
\begin{gathered}
\lim {x \rightarrow b-} f(x)=\lim {x \rightarrow b-} g(x)=0, \
\text { with } g^{\prime}(x) \neq 0 \text { on }(a, b) . \text { Supp } \
\lim {x \rightarrow b-} \frac{f^{\prime}(x)}{g^{\prime}(x)}=L . \end{gathered} $$ and $f^{\prime}$ and $g^{\prime}$ exist on $(a, b)$ with $g^{\prime}(x) \neq 0$ on $(a, b)$. Suppose also that Then claim the rule as his own but Bernoulli accused him of plagarism. Nevertheless, superior to what was known as L’Hôpital’s rule ever since. The version of the rule presented here is superion to what was discovered by Bernoulli and depends on the Cauchy mean value theorem which was found over 100 years after the time of L’Hôpital. By the definition of limit and $8.20 \mathrm{~ t h e r e ~ e x i s t s ~}$ $\left|\frac{f^{\prime}(t)}{g^{\prime}(t)}-L\right|<\frac{\varepsilon}{2} .$ Now pick $x, y$ such that $cc$, Now letting $y \rightarrow b-$, Since $\varepsilon>0$ is arbitrary, this shows $\left|\frac{f(x)-f(y)}{g(x)-g(y)}-L\right|<\frac{\varepsilon}{2} .$ The following corollary is proved in the same way. Corollary $8.8 .2$ Let $[a, b] \subseteq[-\infty, \infty]$ and suppose $f, g(x)$ $\lim {x \rightarrow a+} f(x)=\lim {x \rightarrow a+} g(x)=0, \frac{f(x)}{g(x)}-\varepsilon .$ and $f^{\prime}$ and $g^{\prime}$ exist on $(a, b)$ with $g^{\prime}(x) \neq 0$ on $(a, b)$. Suppose also that $$ \lim {x \rightarrow a+} \frac{f^{\prime}(x)}{g^{\prime}(x)}=L .
$$
Then
$$
\lim {x \rightarrow a+} \frac{f(x)}{g(x)}=L . $$ Here is a simple example which illustrates the use of this rule. The following corollary is proved in the same way. Corollary 8.8.2 Let $[a, b] \subseteq[-\infty, \infty]$ and suppose $f, g$ are functions which satisfy, $$ \lim {x \rightarrow a+} f(x)=\lim _{x \rightarrow a+} g(x)=0,
$$

有一个有趣的规则通常对评估困难的限制很有用,称为 L’Hôpital 的 $^{\top}$ 规则。该规则的最佳版本基于第 141 页的 Cauchy 中值定理,定理 $7.8 .2$。
定理 $8.8 .1$ 令 $[a, b] \subseteq[-\infty, \infty]$ 并假设 $f, g$ 是满足的函数,
$$
\开始{聚集}
\lim {x \rightarrow b-} f(x)=\lim {x \rightarrow b-} g(x)=0, \
\text { with } g^{\prime}(x) \neq 0 \text { on }(a, b) 。 \文本{补充} \
\lim {x \rightarrow b-} \frac{f^{\prime}(x)}{g^{\prime}(x)}=L 。 \结束{聚集} $$ 和 $f^{\prime}$ 和 $g^{\prime}$ 存在于 $(a, b)$ 上 $g^{\prime}(x) \neq 0$ 在 $(a, b)$ .还假设 然后声称该规则是他自己的,但伯努利指责他抄袭。然而,从那以后,它优于被称为 L’Hôpital 的规则。这里介绍的规则版本优于伯努利发现的规则,它依赖于在 L’Hôpital 时代之后 100 多年发现的柯西中值定理。 根据 limit 和 $8.20 的定义 \mathrm{~ t h e r e ~ e x i s t s ~}$ $\left|\frac{f^{\prime}(t)}{g^{\prime}(t)}-L\right|<\frac{\varepsilon}{2} .$ 现在选择 $x, y$ 使得 $cc$, 现在让 $y \rightarrow b-$, 由于 $\varepsilon>0$ 是任意的,这表明 $\left|\frac{f(x)-f(y)}{g(x )-g(y)}-L\right|<\frac{\varepsilon}{2} .$ 以同样的方式证明以下推论。推论 $8.8 .2$ 令 $[a, b] \subseteq[-\infty, \infty]$ 并假设 $f, g(x)$ $\lim {x \rightarrow a+} f(x)=\lim {x \rightarrow a+} g(x)=0, \frac{f(x)}{g(x)}-\varepsilon .$ 和 $f^{\prime}$ 和 $g^{\prime}$ 存在于 $(a, b)$ 上 $g^{\prime}(x) \neq 0$ 在 $(a, b)$ .还假设 $$ \lim {x \rightarrow a+} \frac{f^{\prime}(x)}{g^{\prime}(x)}=L 。
$$
然后
$$
\lim {x \rightarrow a+} \frac{f(x)}{g(x)}=L 。 $$ 这是一个简单的例子,说明了这个规则的使用。 以同样的方式证明以下推论。 推论 8.8.2 令 $[a, b] \subseteq[-\infty, \infty]$ 并假设 $f, g$ 是满足的函数, $$ \lim {x \rightarrow a+} f(x)=\lim _{x \rightarrow a+} g(x)=0,
$$

微积分note Integer Multiples of Irrational Numbers 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。