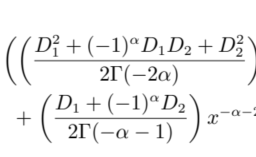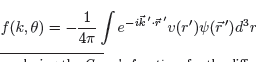数学代写| Intuitionist Logic 离散代考
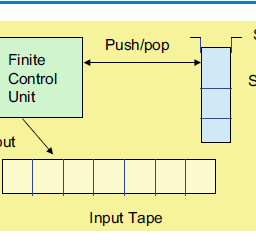
离散数学在计算领域有广泛的应用,例如密码学、编码理论、 形式方法, 语言理论, 可计算性, 人工智能, 理论 数据库和软件的可靠性。 离散数学的重点是理论和应用,而不是为了数学本身而研究数学。 一切算法的基础都是离散数学一切加密的理论基础都是离散数学
编程时候很多奇怪的小技巧(特别是所有和位计算相关的东西)核心也是离散数学
其他相关科目课程代写:组合学Combinatorics集合论Set Theory概率论Probability组合生物学Combinatorial Biology组合化学Combinatorial Chemistry组合数据分析Combinatorial Data Analysis
my-assignmentexpert愿做同学们坚强的后盾,助同学们顺利完成学业,同学们如果在学业上遇到任何问题,请联系my-assignmentexpert™,我们随时为您服务!
离散数学代写
The controversial school of intuitionist mathematics was founded by the Dutch mathematician, L. E. J. Brouwer, who was a famous topologist, and well known for his fixpoint theorem in topology. This constructive approach to mathematics proved to be highly controversial, as its acceptance as a foundation of mathematics would have led to the rejection of many accepted theorems in classical mathematics (including his own fixed point theorem).
Brouwer was deeply interested in the foundations of mathematics, and the problems arising from the paradoxes of set theory. He was determined to provide a secure foundation for mathematics, and his view was that an existence theorem in mathematics that demonstrates the proof of a mathematical object has no validity, object. He, therefore, rejected indirect proof and the law of the excluded middle $(P \vee \neg P)$ or equivalently $(\neg \neg P \rightarrow P)$, and he insisted on an explicit construction of the mathematical object.
The problem with the law of the excluded middle (LEM) arises in dealing with properties of infinite sets. For finite sets, one can decide if all elements of the set possess a certain property $P$ by testing each one. However, this procedure is no longer possible for infinite sets. We may know that a certain element of the infinite set does not possess the property, or it may be the actual method of construction of the set that allows us to prove that every element has the property. However, the application of the law of the excluded middle is invalid for infinite sets, as we a property $P$ that there exists at least one element which does not have the property $P$. In other words, the law of the excluded middle may only be applied in cases where the conclusion can be reached in a finite number of steps.
Consequently, if the Brouwer view of the world were accepted, then many of the classical theorems of mathematics (including his own well-known results in topology) could no longer be said to be true. His approach to the foundations of mathematics hardly made him popular with other mathematicians (the differences were so fundamental that it was more like a war), and intuitionism never became mainstream in mathematics. It led to deep and bitter divisions between Hilbert and Brouwer, with Hilbert accusing Brouwer (and Weyl) of trying to overthrow to science. Hilbert argued that a suitable foundation for mathematics should aim to preserve most of mathematics. Brouwer described Hilbert’s formalist program as a false theory that would produce nothing of mathematical value. For Brouwer, ‘to exist’ is synonymous with ‘constructive existence’, and constructive mathematics is
图论代考
有争议的直觉主义数学学派由著名的拓扑学家 L. E. J. Brouwer 创立,他以拓扑学中的不动点定理而闻名。这种对数学的建设性方法被证明是非常有争议的,因为它被接受为数学的基础会导致拒绝经典数学中许多公认的定理(包括他自己的不动点定理)。
Brouwer 对数学基础以及集合论悖论引发的问题深感兴趣。他决心为数学提供一个安全的基础,他的观点是,证明数学对象的证明的数学存在定理没有有效性,对象。因此,他拒绝间接证明和排中律 $(P \vee \neg P)$ 或等价的 $(\neg \neg P \rightarrow P)$,并坚持数学对象的显式构造.
排中律 (LEM) 的问题出现在处理无限集的性质时。对于有限集合,可以通过测试每个元素来确定集合中的所有元素是否都具有某个属性 $P$。但是,对于无限集,此过程不再可能。我们可能知道无限集的某个元素不具备该属性,或者它可能是集合的实际构造方法使我们能够证明每个元素都具有该属性。但是,排中律的应用对于无限集是无效的,因为我们有一个属性 $P$,它至少存在一个不具有属性 $P$ 的元素。换句话说,排中律可能只适用于可以在有限数量的步骤中得出结论的情况。
因此,如果布劳威尔的世界观被接受,那么许多经典的数学定理(包括他自己在拓扑学中著名的结果)就不能再被说成是正确的了。他对数学基础的研究几乎没有让他受到其他数学家的欢迎(差异是如此根本,以至于更像是一场战争),直觉主义从未成为数学的主流。这导致希尔伯特和布劳威尔之间产生了深刻而痛苦的分歧,希尔伯特指责布劳威尔(和外尔)试图推翻科学。希尔伯特认为,一个合适的数学基础应该旨在保护大部分数学。 Brouwer 将希尔伯特的形式主义纲领描述为一种不会产生任何数学价值的错误理论。对于 Brouwer,“存在”是“建设性存在”的同义词,而建设性数学是

数学代写| DISCRETE MATHEMATICS代考 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。
抽象代数代考
抽象代数就是一门概念繁杂的学科,我们最重要的一点我想并不是掌握多少例子。即便是数学工作者也不会刻意记住Jacobson环、正则环这类东西,重要的是你要知道这门学科的基本工具和基本手法,对概念理解了没有,而这一点不需要用例子来验证,只需要看看你的理解和后续概念是否相容即可。
矩阵论代考matrix theory
数学,矩阵理论是一门研究矩阵在数学上的应用的科目。矩阵理论本来是线性代数的一个小分支,但其后由于陆续在图论、代数、组合数学和统计上得到应用,渐渐发展成为一门独立的学科。
密码学代考
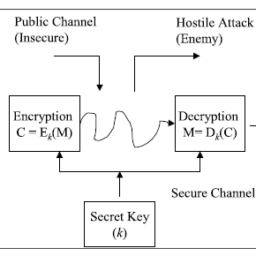
密码学是研究编制密码和破译密码的技术科学。 研究密码变化的客观规律,应用于编制密码以保守通信秘密的,称为编码学;应用于破译密码以获取通信情报的,称为破译学,总称密码学。 电报最早是由美国的摩尔斯在1844年发明的,故也被叫做摩尔斯电码。
- Cryptosystem
- A system that describes how to encrypt or decrypt messages
- Plaintext
- Message in its original form
- Ciphertext
- Message in its encrypted form
- Cryptographer
- Invents encryption algorithms
- Cryptanalyst
- Breaks encryption algorithms or implementations
编码理论代写
编码理论(英语:Coding theory)是研究编码的性质以及它们在具体应用中的性能的理论。编码用于数据压缩、加密、纠错,最近也用于网络编码中。不同学科(如信息论、电机工程学、数学、语言学以及计算机科学)都研究编码是为了设计出高效、可靠的数据传输方法。这通常需要去除冗余并校正(或检测)数据传输中的错误。
编码共分四类:[1]
数据压缩和前向错误更正可以一起考虑。