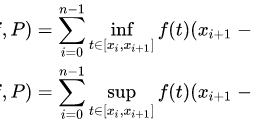0 0

By admin 数学代写 2022年3月21日

微积分（Calculus），数学概念，是高等数学中研究函数的微分(Differentiation)、积分(Integration)以及有关概念和应用的数学分支。它是数学的一个基础学科，内容主要包括极限、微分学、积分学及其应用。微分学包括求导数的运算，是一套关于变化率的理论。它使得函数、速度、加速度和曲线的斜率等均可用一套通用的符号进行讨论。积分学，包括求积分的运算，为定义和计算面积、体积等提供一套通用的方法

my-assignmentexpert™ 微积分calculus作业代写，免费提交作业要求，满意后付款，成绩80\%以下全额退款，安全省心无顾虑。专业硕博写手团队，所有订单可靠准时，保证 100% 原创。my-assignmentexpert™，最高质量的微积分calculus作业代写，服务覆盖北美、欧洲、澳洲等国家。在代写价格方面，考虑到同学们的经济条件，在保障代写质量的前提下，我们为客户提供最合理的价格。由于economics作业种类很多，同时其中的大部分作业在字数上都没有具体要求，因此微积分calculus作业代写的价格不固定。通常在经济学专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。

想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的报价比上列的价格能便宜好几倍。

my-assignmentexpert™ 为您的留学生涯保驾护航在经济学作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的微积分calculus代写服务。我们的专家在微积分calculus学代写方面经验极为丰富，各种微积分calculus相关的作业也就用不着说。

我们提供的econ代写服务范围广, 其中包括但不限于:

单变量微积分
多变量微积分
傅里叶级数
黎曼积分
ODE
微分学

Justify the following statements:
(a) If $\int_{a}^{\infty} f(x) \mathrm{d} x$ exists, then $\int_{c}^{\infty} f(x) \mathrm{d} x$ exists for any $c>a$.
(b) If $\int_{-\infty}^{b} f(x) \mathrm{d} x$ exists, then $\int_{-\infty}^{c} f(x) \mathrm{d} x$ exists for any $c<b$.
(c) If $f$ is defined on $(-\infty, \infty)$ and integrable on every closed and bounded interval, and if $\int_{-\infty}^{c} f(x) \mathrm{d} x$ and $\int_{c}^{\infty} f(x) \mathrm{d} x$ exist for some $c \in \mathbb{R}$, then $\int_{-\infty}^{d} f(x) \mathrm{d} x$ and $\int_{d}^{\infty} f(x) \mathrm{d} x$ exist for any $d \in \mathbb{R}$.
Suppose $f: \mathbb{R} \rightarrow \mathbb{R}$ is an even function, that is, $f(-x)=f(x)$ for every $x \in \mathbb{R}$. Prove that $\int_{-\infty}^{\infty} f(x) \mathrm{d} x$ exists if and only if $\int_{0}^{\infty} f(x) \mathrm{d} x$ exists, and in that case
$$
\int_{-\infty}^{\infty} f(x) \mathrm{d} x=2 \int_{0}^{\infty} f(x) \mathrm{d} x
$$
Suppose $f: \mathbb{R} \rightarrow \mathbb{R}$ is an odd function, that is, $f(-x)=-f(x)$ for every $x \in \mathbb{R}$. Prove that $\int_{-\infty}^{0} f(x) \mathrm{d} x$ exists if and only if $\int_{0}^{\infty} f(x) \mathrm{d} x$ exists, and in that case
$$
\int_{-\infty}^{\infty} f(x) \mathrm{d} x=0
$$
Justify the following statements:
(a) Suppose $f$ is defined on $[a, \infty)$ with values in $[0, \infty)$. Then $\int_{a}^{\infty} f(x) \mathrm{d} x$ converges if and only if $\lim {n \rightarrow \infty} \int{a}^{n} f(x) \mathrm{d} x$ exists.
(b) Suppose $f$ is defined on $(-\infty, b]$ with values in $[0, \infty)$. Then $\int_{-\infty}^{b} f(x) \mathrm{d} x$ converges if and only if $\lim {n \rightarrow \infty} \int{-n}^{b} f(x) \mathrm{d} x$ exists.

证明以下陈述的合理性：
(a) 如果∫一种∞F(X)dX存在，那么∫C∞F(X)dX存在于任何C>一种.
(b) 如果∫−∞bF(X)dX存在，那么∫−∞CF(X)dX存在于任何C<b.
(c) 如果F定义在(−∞,∞)并且在每个闭有界区间上可积，如果∫−∞CF(X)dX和∫C∞F(X)dX为某些人而存在C∈R，然后∫−∞dF(X)dX和∫d∞F(X)dX存在于任何d∈R.
认为F:R→R是偶函数，即F(−X)=F(X)对于每个X∈R. 证明∫−∞∞F(X)dX当且仅当存在∫0∞F(X)dX存在，并且在这种情况下
∫−∞∞F(X)dX=2∫0∞F(X)dX
认为F:R→R是一个奇函数，即F(−X)=−F(X)对于每个X∈R. 证明∫−∞0F(X)dX当且仅当存在∫0∞F(X)dX存在，并且在这种情况下
∫−∞∞F(X)dX=0
证明以下陈述的合理性：
(a) 假设F定义在[一种,∞)与值[0,∞). 然后∫一种∞F(X)dX收敛当且仅当 $\lim {n \rightarrow \infty} \int {a}^{n} f(x) \mathrm{d} x和X一世s吨s.(b)小号你pp○s和F一世sd和F一世n和d○n(-\infty, b]在一世吨Hv一种一世你和s一世n[0, \infty).吨H和n\int_{-\infty}^{b} f(x) \mathrm{d} xC○nv和rG和s一世F一种nd○n一世和一世F\lim {n \rightarrow \infty} \int {-n}^{b} f(x) \mathrm{d} x$ 存在。