如果你也在 怎样金融数学Financial Mathematics这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。金融数学Financial Mathematics是将数学方法应用于金融问题。(有时使用的同等名称是定量金融、金融工程、数学金融和计算金融)。它借鉴了概率、统计、随机过程和经济理论的工具。传统上,投资银行、商业银行、对冲基金、保险公司、公司财务部和监管机构将金融数学的方法应用于诸如衍生证券估值、投资组合结构、风险管理和情景模拟等问题。依赖商品的行业(如能源、制造业)也使用金融数学。 定量分析为金融市场和投资过程带来了效率和严谨性,在监管方面也变得越来越重要。
定量金融作为经济学的一个子领域,关注资产和金融工具的估值以及资源的配置。几个世纪的经验产生了关于经济运行方式和我们评估资产的方式的基本理论。模型描述了基本变量之间的关系,如资产价格、市场运动和利率。这些数学工具使我们能够得出原本难以发现或从直觉上无法立即看出的结论。模型应用的一个例子是银行的压力测试。 特别是在现代计算技术的帮助下,我们可以存储大量的数据并同时对许多变量进行建模,从而有能力对相当大和复杂的系统进行建模。因此,科学计算的技术,如数值分析、蒙特卡洛模拟和优化是金融数学的重要组成部分。
任何科学的很大一部分都是在对研究对象的基本了解的基础上建立可检验的假设,并通过可重复的研究来证明或反驳这些假设的能力。从这个角度来看,数学是代表理论的语言,并提供测试其有效性的工具。例如,在布莱克、斯科尔斯和默顿的期权定价理论中,提出了一个股票价格变动的模型,结合无风险投资将获得无风险收益率的理论,研究者们推断出可以给期权分配一个价值。
my-assignmentexpert™金融数学Financial Mathematics作业代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。my-assignmentexpert™, 最高质量的金融数学Financial Mathematics作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于统计Statistics作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此金融数学Financial Mathematics作业代写的价格不固定。通常在经济学专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
my-assignmentexpert™ 为您的留学生涯保驾护航 在金融数学Financial Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的金融数学Financial Mathematics代写服务。我们的专家在金融数学Financial Mathematics代写方面经验极为丰富,各种金融数学Financial Mathematics相关的作业也就用不着 说。
我们提供的金融数学Financial Mathematics及其相关学科的代写,服务范围广, 其中包括但不限于:
- 随机微积分 Stochastic calculus
- 随机分析 Stochastic analysis
- 随机控制理论 Stochastic control theory
- 微观经济学 Microeconomics
- 数量经济学 Quantitative Economics
- 宏观经济学 Macroeconomics
- 经济统计学 Economic Statistics
- 经济学理论 Economic Theory
- 计量经济学 Econometrics
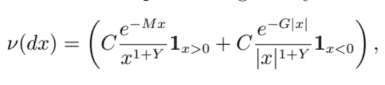
经济代写
数学代写|金融数学作业代写Financial Mathematics代考|L´evy Process
Let $\left(X_{t}\right){t \geq 0}$ be a stochastic process on a probability space. $X{t}$ is a Lévy process if it has the following properties
- $X_{0}=0$.
- $X_{t}$ has independent increments.
- $X_{t}$ has stationary increments.
- $X_{t}$ is stochastically continuous. More precisely, $\forall t \geq 0$ and $a>0$
$$
\lim {s \rightarrow t} P\left[\left|X{s}-X_{t}\right|>a\right]=0 .
$$ - $X_{t}$ is a cadlag.
数学代写|金融数学作业代写 Financial Mathematics代考|CGMY Process
The Lévy measure for the CGMY process is given by
$$
\nu(d x)=\left(C \frac{e^{-M x}}{x^{1+Y}} \mathbf{1}{x>0}+C \frac{e^{-G|x|}}{|x|^{1+Y}} \mathbf{1}{x<0}\right), $$ where $C, G, M>0,0 \leq Y<2$. These parameters fully describe the properties of the CGMY process. In other words, $G$ and $M$ show the rate of decay of the left and right tail, respectively. $Y$ is the exponential exponent which plays a major role in determining the rate of decay of the tails.
The CGMY processes are obtained by tempering the tails of stable processes. For this reason, it is also referred to as the classical tempered stable process. There is a relationship between the Lévy measure for the stable process and CGMY process. By setting $G=M=0$ and choosing different values for parameter $C$ for the left and right one can achieve the Lévy measure of the stable process. A drawback of the stable process is that only the first moment exists. By tempering the tails of the stable process, a finite moment of any order can be obtained. Some researchers use the tempered stable process instead of the CGMY process. Detailed information about the stable and tempered stable process can be found in Samorodnitsky and Taqqu (1994) and Rachev et al. (2011).
数学代写|金融数学作业代写 FINANCIAL MATHEMATICS代考|Stochastic Differential Equation
If the dynamics of the asset price process are characterized by the semimartingale property, the stochastic differential equation of the option price represents a useful model for empirical application. More specifically, the absence of arbitrage is
equivalent with semimartingale property. The stochastic differential equation for the option price when the dynamics of the asset price is a CGMY process can be obtained by constructing a simple portfolio with two risky and risk-free assets. More precisely, let $N_{t}$ be the price process for the risk-free asset and $S_{t}$ the logprice process of a risky asset, then the stochastic differential equation for the CGMY process is given by
$$
\begin{aligned}
d N_{t} &=r N_{t} d t \
d S_{t} &=\left(r-d+\omega_{S}\right) d t+d X_{t},
\end{aligned}
$$
where $X_{t}, r$, and $d$ are the CGMY process, risk-free interest rate, and dividend, respectively, and
$$
\omega_{S}=\Phi\left(-i, X_{t}\right)
$$
where $\omega_{t}$ is the martingale correction. In other words, by choosing a suitable value for $\omega$ one can be sure that the dynamics of the asset price is a martingale under the risk-neutral measure. ${ }^{15}$ It should be noted that the first fundamental theorem of asset pricing shows the necessary and sufficient conditions for absence of arbitrage in a market. It shows that there exists a risk-neutral measure, which is equivalent to the physical measure. ${ }^{16}$
数学代写|金融数学作业代写FINANCIAL MATHEMATICS代考|L´EVY PROCESS
让 $\leftX_{t}\右X_{t}\右{t\geq 0}b和一种s吨○CH一种s吨一世Cpr○C和ss○n一种pr○b一种b一世一世一世吨和sp一种C和.X {t}$ 是一个 Lévy 过程,如果它具有以下属性
- X0=0.
- X吨有独立的增量。
- X吨有固定增量。
- X吨是随机连续的。更确切地说,∀吨≥0和一种>0
$$
\lim {s \rightarrow t} P\left[\left|X {s}-X_{t}\right|>a\right]=0 。
$$ - X吨是一个cadlag。
数学代写|金融数学作业代写 FINANCIAL MATHEMATICS代考|CGMY PROCESS
CGMY 过程的 Lévy 度量由
$$
\nu给出dX=\left(C \frac{e^{-M x}}{x^{1+Y}} \mathbf{1} {x>0}+C \frac{e^{-G|x|}} {|x|^{1+Y}} \mathbf{1} {x<0}\right), $$ 其中C,G,米>0,0≤和<2. 这些参数充分描述了 CGMY 过程的特性。换一种说法,G和米分别显示左右尾巴的衰减率。和是指数指数,它在确定尾部衰减率中起主要作用。
CGMY 工艺是通过对稳定工艺的尾部进行回火而获得的。因此,它也被称为经典的回火稳定过程。稳定过程的 Lévy 测度与 CGMY 过程之间存在关系。通过设置G=米=0并为参数选择不同的值C对于左右一个可以实现稳定过程的Lévy测度。稳定过程的一个缺点是只存在第一时刻。通过回火稳定过程的尾部,可以获得任意阶的有限矩。一些研究人员使用回火稳定工艺代替 CGMY 工艺。有关稳定和回火稳定过程的详细信息,请参见 Samorodnitsky 和 Taqqu1994和拉切夫等人。2011.
数学代写|金融数学作业代写 FINANCIAL MATHEMATICS代考|STOCHASTIC DIFFERENTIAL EQUATION
如果资产价格过程的动态具有半鞅性质,则期权价格的随机微分方程代表了一个有用的经验模型。更具体地说,没有套利是
等价于半鞅性质。资产价格动态为 CGMY 过程时期权价格的随机微分方程可以通过构建具有两种风险资产和无风险资产的简单投资组合得到。更准确地说,让ñ吨是无风险资产的价格过程和小号吨风险资产的对数价格过程,则 CGMY 过程的随机微分方程由下式给出
dñ吨=rñ吨d吨 d小号吨=(r−d+ω小号)d吨+dX吨,
在哪里X吨,r, 和d分别是 CGMY 过程、无风险利率和股息,以及
ω小号=披(−一世,X吨)
在哪里ω吨是鞅修正。换句话说,通过选择一个合适的值ω可以肯定的是,在风险中性测度下,资产价格的动态是一种鞅。15需要注意的是,资产定价第一基本定理表明了市场上不存在套利的充分必要条件。它表明存在一个风险中性的度量,它等效于物理度量。16

计量经济学代写请认准my-assignmentexpert™ Economics 经济学作业代写
微观经济学代写请认准my-assignmentexpert™ Economics 经济学作业代写
宏观经济学代写请认准my-assignmentexpert™ Economics 经济学作业代写