如果你也在 怎样代写凸分析Convex Analysis这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。凸分析Convex Analysis是数学的一个分支,专门研究凸函数和凸集的属性,通常应用于凸最小化,这是优化理论的一个子领域。
my-assignmentexpert™ 凸分析Convex Analysis作业代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。my-assignmentexpert™, 最高质量的凸分析Convex Analysis作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于统计Statistics作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此凸分析Convex Analysis作业代写的价格不固定。通常在经济学专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
my-assignmentexpert™ 为您的留学生涯保驾护航 在数学mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的凸分析Convex Analysis作业代写代写服务。我们的专家在数学mathematics代写方面经验极为丰富,各种凸分析Convex Analysis相关的作业也就用不着 说。
我们提供的凸分析Convex Analysis及其相关学科的代写,服务范围广, 其中包括但不限于:、
- 优化理论 optimization theory
- 变分法 Calculus of variations
- 最优控制理论 Optimal control
- 动态规划 Dynamic programming
- 鲁棒优化 Robust optimization
- 随机优化 Stochastic programming
- 组合优化 Combinatorial optimization

数学代考|凸分析作业代写Convex Analysis代考|Rademacher’s Theorem
We mentioned Rademacher’s fundamental theorem on the differentiability of Lipschitz functions in the context of the Intrinsic Clarke subdifferential formula (Theorem 6.2.5):
$$
\partial_{\circ} f(x)=\operatorname{conv}\left\{\lim _{r} \nabla \dot{f}\left(x^{r}\right) \mid x^{r} \rightarrow x, x^{r} \notin Q\right\}
$$
valid whenever the function $f: \mathbf{E} \rightarrow \mathbf{R}$ is locally Lipschitz around the point $x \in \mathbf{E}$ and the set $Q \subset \mathbf{E}$ has measure zero. We prove Rademacher’s theorem in this section, taking a slight diversion into some basic measure theory.
数学代考|凸分析作业代写Convex Analysis代考|Proximal Normals and Chebyshev Sets
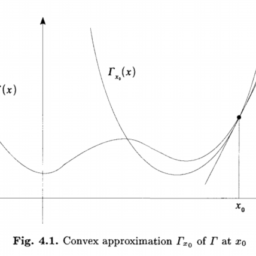
We introduced the Clarke normal cone in Section $6.3$ (Tangent Cones), via the Clarke subdifferential. An appealing alternative approach begins with a more geometric notion of a normal vector. We call a vector $y \in \mathbf{E}$ a proximal normal to a set $S \subset \mathbf{E}$ at a point $x \in S$ if, for some $t>0$, the nearest point to $x+t y$ in $S$ is $x$. The set of all such vectors is called the proximal normal cone, which we denote $N_{S}^{p}(x)$.
The proximal normal cone, which may not be convex, is contained in the Clarke normal cone (Exercise 3 ). The containment may be strict, but we can reconstruct the Clarke normal cone from proximal normals using the following result.
数学代考|凸分析作业代写Convex Analysis代考|Amenable Sets and Prox-Regularity
In the previous section we saw that nonempty closed convex subsets $S$ of the Euclidean space $\mathbf{E}$ are characterized by the attractive global property that every point in $\mathbf{E}$ has a unique nearest point in $S$. The corresponding local property is also a useful tool: we begin with a condition guaranteeing this property.
We call the closed set $S$ prox-regular at a point $\bar{x}$ in $S$ if there exists a constant $\rho>0$ such that all distinct points $x, x^{\prime} \in S$ near $\bar{x}$ and small vectors $v \in N_{S}(x)$ satisfy the inequality
$$
\left\langle v, x^{\prime}-x\right\rangle<\rho\left\|x^{\prime}-x\right\|^{2}
$$
Geometrically, this condition states that the ball centered at the point $x+\frac{1}{2 \rho} v$ containing the point $x$ on its boundary has interior disjoint from $S$.
数学代考|凸分析作业代写CONVEX ANALYSIS代考|RADEMACHER’S THEOREM
我们在 Intrinsic Clarke 次微分公式的背景下提到了关于 Lipschitz 函数可微性的 Rademacher 基本定理吨H和这r和米6.2.5:
∂∘F(X)=转化率{林r∇F˙(Xr)∣Xr→X,Xr∉问}
只要函数有效F:和→R在该点附近是局部 LipschitzX∈和和集合问⊂和测量为零。我们在本节证明 Rademacher 定理,稍微转移到一些基本的测度理论。
数学代考|凸分析作业代写CONVEX ANALYSIS代考|PROXIMAL NORMALS AND CHEBYSHEV SETS
我们在章节中介绍了克拉克法向锥6.3 吨一种nG和n吨C这n和s,通过克拉克次微分。一种吸引人的替代方法始于法线向量的更多几何概念。我们称向量是∈和集合的近端法线小号⊂和在某一点X∈小号如果,对于某些人吨>0, 最近的点X+吨是在小号是X. 所有这些向量的集合称为近端法向锥,我们将其表示为ñ小号p(X).
可能不是凸的近端法向锥包含在克拉克法向锥中和X和rC一世s和3. 包含可能是严格的,但我们可以使用以下结果从近端法线重建克拉克法线锥。
数学代考|凸分析作业代写CONVEX ANALYSIS代考|AMENABLE SETS AND PROX-REGULARITY
在上一节中,我们看到非空闭凸子集小号欧几里得空间和其特点是具有吸引力的全局属性,每个点和有一个唯一的最近点小号. 相应的局部属性也是一个有用的工具:我们从保证该属性的条件开始。
我们称闭集小号prox-regular at a pointX¯在小号如果存在一个常数ρ>0这样所有不同的点X,X′∈小号靠近X¯和小向量v∈ñ小号(X)满足不等式
⟨v,X′−X⟩<ρ‖X′−X‖2
从几何上看,这个条件表明球以该点为中心X+12ρv包含点X在其边界上与小号.

数学代考|凸分析作业代写Convex Analysis代考 请认准UpriviateTA. UpriviateTA为您的留学生涯保驾护航。
更多内容请参阅另外一份复分析代写.