如果你也在 怎样代写概率论Probability Theory 这个学科遇到相关的难题，请随时右上角联系我们的24/7代写客服。概率论Probability Theory作为统计学的数学基础，对许多涉及数据定量分析的人类活动至关重要。概率论的方法也适用于对复杂系统的描述，只对其状态有部分了解，如在统计力学或顺序估计。二十世纪物理学的一个伟大发现是量子力学中描述的原子尺度的物理现象的概率性质。

概率论Probability Theory STAT131的核心课题包括离散和连续随机变量、概率分布和随机过程（为非决定性或不确定的过程或测量量提供数学抽象，这些过程或测量量可能是单一发生的，或以随机方式随时间演变）。尽管不可能完美地预测随机事件，但对它们的行为可以有很多说法。概率论中描述这种行为的两个主要结果是大数法则和中心极限定理。概率论是与概率有关的数学分支。虽然有几种不同的概率解释，但概率论以严格的数学方式处理这一概念，通过一组公理来表达它。

同学们在留学期间，都对各式各样的作业考试很是头疼，如果你无从下手，不如考虑my-assignmentexpert™！

my-assignmentexpert™提供最专业的一站式服务：Essay代写，Dissertation代写，Assignment代写，Paper代写，Proposal代写，Proposal代写，Literature Review代写，Online Course，Exam代考等等。my-assignmentexpert™专注为留学生提供Essay代写服务，拥有各个专业的博硕教师团队帮您代写，免费修改及辅导，保证成果完成的效率和质量。同时有多家检测平台帐号，包括Turnitin高级账户，检测论文不会留痕，写好后检测修改，放心可靠，经得起任何考验！

数学代写|概率论代考Probability Theory代写|Gender bias? Simpson’s paradox

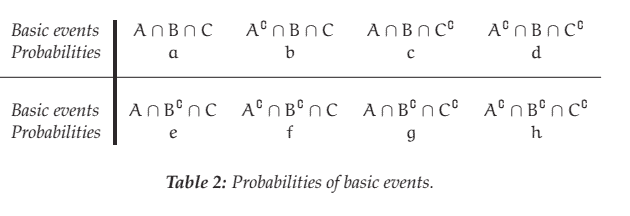
Is there pervasive gender bias in graduate admissions to elite colleges? In the fall of 1973, the Graduate Division of the University of California, Berkeley, admitted $44 \%$ of male applicants and $35 \%$ of female applicants. The apparent gender bias prompted a study of graduate admissions at the university. ${ }^1$ The following hypothetical data for two unusual departments, Social Warfare and Machismatics, in a make-believe university are taken from the Berkeley study and illustrate the perils in making snap judgements.

Comparing admission ratios by department, it is clear that in the Department of Social Warfare the admission rate of $1 / 10$ for women is twice that of the admission rate $1 / 20$ for men, while in the Department of Machismatics the admission rate of 19/20 for women far outstrips the admission rate of $1 / 2$ for men. While the significance of the results may be debated, it is clear that the ratios at any rate suggest an admissions edge for women. The picture changes dramatically, however, if the situation is considered in entirety for both departments put together. Now the cumulative admissions rate for women is $39 / 220$ or about one in five while the men enjoy an admissions rate of $101 / 220$ or about one in two. Viewed holistically, the men seem to have a decided edge in admissions though the individual parts show a fairly pronounced edge for women. How to read this riddle?

Suppose a member of the aspiring graduates is selected at random from the pool of 440 applicants. Let $A$ be the event that the student is admitted, B the event that the student is a woman, and $C$ the event that the student is an applicant to the Department of Social Warfare. In notation then, the apparently paradoxical situation before us is expressed via the observation that while
$$
\mathbf{P}(A \mid B \cap C) \geq \mathbf{P}\left(A \mid B^C \cap C\right) \text { and } \mathbf{P}\left(A \mid B \cap C^C\right) \geq \mathbf{P}\left(A \mid B^C \cap C^C\right) \text {, }
$$
yet, when the situations are combined,
$$
\mathbf{P}(A \mid B) \leq \mathbf{P}\left(\mathrm{A} \mid B^C\right),
$$

and the inequality appears to unfairly change direction.

数学代写|概率论代考Probability Theory代写|The theorem of total probability

The countable additivity of probability measure manifests itself under conditioning in an extremely useful, if elementary, form. Some terminology first. We say that a sequence of events, $\left{A_j, j \geq 1\right}$, forms a measurable partition of $\Omega$ if $A_j \cap A_k=\varnothing$ if $j \neq k$ and $\bigcup_j A_j=\Omega$, that is to say, the event sequence is pairwise disjoint and covers the sample space.

THEOREM OF TOTAL PROBABILITY Suppose $\left{A_j, j \geq 1\right}$ is a measurable partition of $\Omega$ and $\mathbf{P}\left(A_j\right)>0$ for each $j$. Then, for every event $H$, we have
$$
\mathbf{P}(H)=\sum_{j \geq 1} \mathbf{P}\left(A_j \cap H\right)=\sum_{j \geq 1} \mathbf{P}\left(H \mid A_j\right) \mathbf{P}\left(A_j\right)
$$
PROOF: The result follows from the trite observation $H=\bigcup_j\left(A_j \cap H\right)($ as $H \subseteq$ $\left.\Omega=\bigcup_j A_j\right)$. Countable additivity of probability measure completes the proof as $\left{A_j \cap H, j \geq 1\right}$ is a sequence of pairwise disjoint events.

The simplest non-trivial measurable partition of the sample space arises by splitting up $\Omega$ into an event and its complement. Thus, if $A$ is any event in the probability space then $\left{A, A^c\right}$ is a measurable partition of $\Omega$. In consequence,
$$
\mathbf{P}(\mathrm{H})=\mathbf{P}(\mathrm{H} \mid A) \mathbf{P}(A)+\mathbf{P}\left(\mathrm{H} \mid A^c\right) \mathbf{P}\left(A^c\right)
$$
for any pair of events $\mathrm{H}$ and $\mathrm{A}$ where, to obviate trivialities, we suppose that both apparently banal observation turns out to be quite amazingly useful and will in itself pay the rent as we see in the examples that follow.

概率论代写

数学代写|概率论代考PROBABILITY THEORY代写|GENDER BIAS? SIMPSON’S PARADOX

精英大学的研究生录取是否存在普遍存在的性别偏见？1973年秋，加州大学伯克利分校研究生部录取 $44 \%$ 男性申请人和 $35 \%$ 的女性申请人。明显的性别偏见促使对 大学研究生招生进行了研究。 ${ }^1$ 以下关于一所虚构大学中两个不同寻常的系，社会战和机械学的假设数据来自伯克利的研究，并说明了做出仓促判断的危险。
比较各部门的录取率，很明显，在社会战争系， $1 / 10$ 女性是录取率的两倍 $1 / 20$ 对于男性，而在机械学系，女性的 $19 / 20$ 录取率远远超过了 $1 / 2$ 对于男人。虽然结果 的重要性可能存在争议，但很明显，无论如何，这些比率表明女性在录取方面具有优势。然而，如果将两个部门放在一起整体考虑情况，情况就会发生巨大变化。 现在女性的累计录取率为 $39 / 220$ 或大约五分之一，而男性的录取率为 $101 / 220$ 或大约二分之一。从整体上看，男性似乎在录取方面具有决定性优势，尽管个别部 分对女性表现出相当明显的优势。这个蒾语怎么读?
假设从 440 名申请者中随机选出一名有抱负的毕业生。让 $A$ 是学生被录取的事件， $B$ 学生是女性的事件，以及如果学生是社会战部的申请人。那么在符号中，摆 在我们面前的明显矛盾的情况是通过观察来表达的，虽然
$$
\mathbf{P}(A \mid B \cap C) \geq \mathbf{P}\left(A \mid B^C \cap C\right) \text { and } \mathbf{P}\left(A \mid B \cap C^C\right) \geq \mathbf{P}\left(A \mid B^C \cap C^C\right),
$$
然而，当情况结合在一起时，
$$
\mathbf{P}(A \mid B) \leq \mathbf{P}\left(\mathrm{A} \mid B^C\right)
$$
不平等似乎不公平地改变了方向。

数学代写|概率论代考PROBABILITY THEORY代写|THE THEOREM OF TOTAL PROBABILITY

概率测度的可数可加性在条件下以极其有用的（如果是基本的）形式表现出来。首先是一些术语。我们说一系列事件， \left{A_j,j $\backslash g e q 1 \backslash r i g h t}$, 形成一个可测量的分 区 $\Omega$ 如果 $A_j \cap A_k=\varnothing$ 如果 $j \neq k$ 和 $\bigcup_j A_j=\Omega$ ，也就是说，事件序列是成对不相交的，并且票盖了样本空间。
$$
\mathbf{P}(H)=\sum_{j \geq 1} \mathbf{P}\left(A_j \cap H\right)=\sum_{j \geq 1} \mathbf{P}\left(H \mid A_j\right) \mathbf{P}\left(A_j\right)
$$
样本空间的最简单的非平凡可测分区是通过分裂产生的 $\Omega$ 变成事件及其补充。因此，如果 $A$ 是概率空间中的任何事件然姤 $\backslash$ 左 ${\mathrm{A}, \mathrm{A} C \backslash$ 右 $}$ 是一个可测量的分区 $\Omega$. 结 果
$$
\mathbf{P}(\mathrm{H})=\mathbf{P}(\mathrm{H} \mid A) \mathbf{P}(A)+\mathbf{P}\left(\mathrm{H} \mid A^c\right) \mathbf{P}\left(A^c\right)
$$
对于任何一对事件H和 $\mathrm{A}$ 在这里，为了避免顼碎的事情，我们假设表面上平淡无奇的观察结果非常有用，并且本身会支付租金，正如我们在下面的例子中看到的那 样。

数学代写|概率论代考Probability Theory代写 请认准exambang™. exambang™为您的留学生涯保驾护航。

微观经济学代写

微观经济学是主流经济学的一个分支，研究个人和企业在做出有关稀缺资源分配的决策时的行为以及这些个人和企业之间的相互作用。my-assignmentexpert™ 为您的留学生涯保驾护航 在数学Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在图论代写Graph Theory代写方面经验极为丰富，各种图论代写Graph Theory相关的作业也就用不着 说。

线性代数代写

线性代数是数学的一个分支，涉及线性方程，如：线性图，如：以及它们在向量空间和通过矩阵的表示。线性代数是几乎所有数学领域的核心。

博弈论代写

现代博弈论始于约翰-冯-诺伊曼（John von Neumann）提出的两人零和博弈中的混合策略均衡的观点及其证明。冯-诺依曼的原始证明使用了关于连续映射到紧凑凸集的布劳威尔定点定理，这成为博弈论和数学经济学的标准方法。在他的论文之后，1944年，他与奥斯卡-莫根斯特恩（Oskar Morgenstern）共同撰写了《游戏和经济行为理论》一书，该书考虑了几个参与者的合作游戏。这本书的第二版提供了预期效用的公理理论，使数理统计学家和经济学家能够处理不确定性下的决策。

微积分代写

微积分，最初被称为无穷小微积分或 “无穷小的微积分”，是对连续变化的数学研究，就像几何学是对形状的研究，而代数是对算术运算的概括研究一样。

它有两个主要分支，微分和积分；微分涉及瞬时变化率和曲线的斜率，而积分涉及数量的累积，以及曲线下或曲线之间的面积。这两个分支通过微积分的基本定理相互联系，它们利用了无限序列和无限级数收敛到一个明确定义的极限的基本概念 。

计量经济学代写

什么是计量经济学？
计量经济学是统计学和数学模型的定量应用，使用数据来发展理论或测试经济学中的现有假设，并根据历史数据预测未来趋势。它对现实世界的数据进行统计试验，然后将结果与被测试的理论进行比较和对比。

根据你是对测试现有理论感兴趣，还是对利用现有数据在这些观察的基础上提出新的假设感兴趣，计量经济学可以细分为两大类：理论和应用。那些经常从事这种实践的人通常被称为计量经济学家。

Matlab代写

MATLAB 是一种用于技术计算的高性能语言。它将计算、可视化和编程集成在一个易于使用的环境中，其中问题和解决方案以熟悉的数学符号表示。典型用途包括：数学和计算算法开发建模、仿真和原型制作数据分析、探索和可视化科学和工程图形应用程序开发，包括图形用户界面构建MATLAB 是一个交互式系统，其基本数据元素是一个不需要维度的数组。这使您可以解决许多技术计算问题，尤其是那些具有矩阵和向量公式的问题，而只需用 C 或 Fortran 等标量非交互式语言编写程序所需的时间的一小部分。MATLAB 名称代表矩阵实验室。MATLAB 最初的编写目的是提供对由 LINPACK 和 EISPACK 项目开发的矩阵软件的轻松访问，这两个项目共同代表了矩阵计算软件的最新技术。MATLAB 经过多年的发展，得到了许多用户的投入。在大学环境中，它是数学、工程和科学入门和高级课程的标准教学工具。在工业领域，MATLAB 是高效研究、开发和分析的首选工具。MATLAB 具有一系列称为工具箱的特定于应用程序的解决方案。对于大多数 MATLAB 用户来说非常重要，工具箱允许您学习和应用专业技术。工具箱是 MATLAB 函数（M 文件）的综合集合，可扩展 MATLAB 环境以解决特定类别的问题。可用工具箱的领域包括信号处理、控制系统、神经网络、模糊逻辑、小波、仿真等。